Three Dimensional Geometry

SYNOPSIS

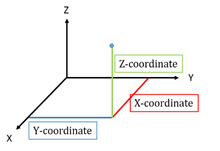
1. 3D geometry is a three-dimensional geometry where each point is described in three-dimensional space with three coordinate axes named X, Y and Z.
   
   

2. Section Formula:
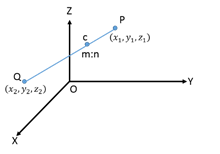
   If c is the point which divides a line PQ in the ratio m:n, then the coordinates of c are given by
   c =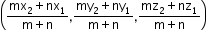
   
   

3. Direction Cosines:
   The angles α, β and γ made by the directed line L passing through the origin with x, y and z axes respectively are called direction angles.
   Cosines of these angles (cos α, cos β and cos γ) are called the direction cosines of line L.
   Note: The direction cosines of the directed line not passing through the origin can be obtained by drawing a line parallel to it which passes through the origin.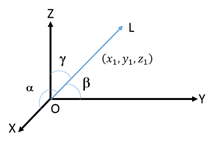
   
   

4. Direction Ratios:
   If l, m and n are the cosines of the vector  and a, b and c are three numbers such that they are proportional to l, m and n respectively, then a, b and c are called the direction ratios of the vector  .
   Also,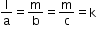 

5. For points P(x₁, y_1, z₁) and Q(x₂, y₂, z₂), the direction ratios of line PQ are
   .

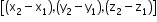6. A line is uniquely determined if it passes through
   

   1.  a given point and parallel to a vector/line having direction cosines
   2.  the two given points
7. Two lines having direction cosines l₁, m₁, n₁ and l₂, m₂, n₂ are
   
   1. Perpendicular if and only if l₁ l₂ + m₁ m₂ + n₁ n₂ = 0
   2.  Parallel if 
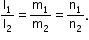
8. Two lines having direction ratios a₁, b₁, c₁ and a₂, b₂, c₂ are
   
   1. Perpendicular if and only if a₁ a₂ + b₁ b₂ + c₁ c₂ = 0
   2.  Parallel if 
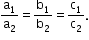
9. If two lines intersect at a point, then the shortest distance between them is zero.
10. If two lines are parallel, then the shortest distance between them is the perpendicular distance.
11. Two lines which are neither parallel nor intersect are called skew lines. 
    These lines are non-coplanar, i.e. they do not belong to the same 2D plane.
    
    
12. Angle between two skew lines:
    Angle between two skew lines is the angle between two lines which are drawn from any point and parallel to each of the skew lines.
13. A normal vector, simply called normal, is a vector perpendicular to a surface.
14. Plane:
    A plane is a surface such that a line joining any two points taken on it lies completely on the surface.
    A plane is uniquely determined if

    1. The normal to the plane and its distance from the origin is given.
    2. It passes through a point and perpendicular to a given direction.
    3. It passes through three given non-collinear points.
       Note: A line containing three collinear points can be a part of many planes.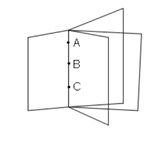
       
       

15. Angle between two planes is the angle between their normals.
16. Planes a₁x + b₁y + c₁z + d₂ = 0 and a₂x + b₂y + c₂z +d₂ = 0 are
    
    1. Perpendicular if a₁a₂ + b₁b₂ + c₁c₂ = 0
    2. Parallel if 
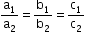
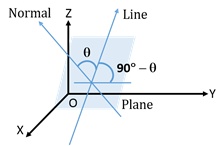
17. The angle between a line and a plane is the complement of the angle between a line and the normal to the plane.
    
    
18. Distance of a point from a plane is the length of the line (perpendicular to the plane) from the plane to the point.