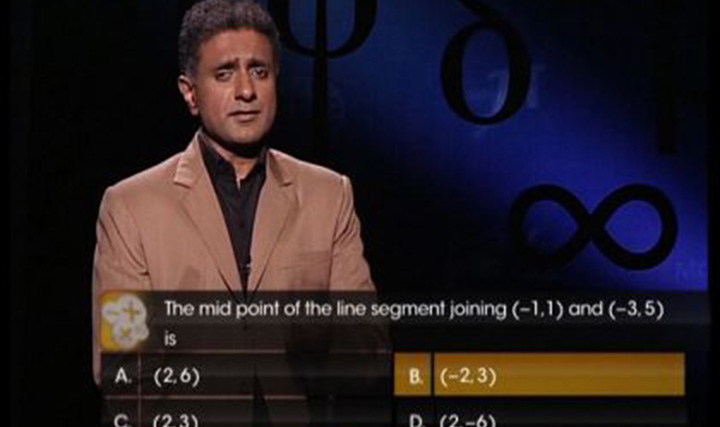
CBSE Class 11-science - Normal and General Form of A Line Videos
- Find the equation of a line whose perpendicular distance from the origin is 6 units and the angle made by the perpendicular with positive x-axis is 60°.
- Reduce the equation 4x + 3y – 9 = 0 to the normal form and find their length of perpendicular from origin to the line
- Find the distance of the point (2, 5) from the line 4 (x + 5) = 7 (2y - 3).
- Find the distance between the parallel lines 3x + 5y + 13 = 0 and 3x + 5y – 24 = 0.
-
Find the equation of the line parallel to the line 24x
5y + 23 = 0 and passing through the point (3, -5).
- Find the coordinates of the foot of the perpendicular from the point (3, -4) to the line 4x - 15y + 17 = 0.
- Find the equation of the line perpendicular to the line 2x - 3y + 7 = 0 and having x-intercept 4.
- If p and q are the lengths of perpendicular from the origin to the lines x cos q - y sin q = k cos 2 q and x sec q + y cosec q = k respectively. Prove that p2 + 4q2 = k2.
-
Find the points on the y-axis, whose distances from the line
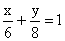are 5 units.
- Find the distance between the parallel lines m (x + y) – n = 0 and mx + my + r = 0.
