CBSE Class 11-science Answered

PRESSURE INSIDE A LIQUID DROP

Consider a small spherical liquid drop of a certain radius 'r'. Have a look at the figure below

Two types of pressure act on this drop acting in opposite directions - pressure due to surface tension inwards (or atmospheric pressure) and internal pressure acting outwards. The pressure (or force) always acts perpendicular to the drop surface. 

Obviously, the liquid drop would not does not automatically collapse into a single point (on account of the same surface tension). 

Thus, the internal pressure  is greater than the surface tension pressure and so the net effect is that pressure exerts outwards, as shown by the arrows in the figure.

This net pressure tends to increase the size of the drop and thus its radius. Let the increase in radius be by 'dr' and the final radius becomes 'r + dr'.

Now, let's do some mathematics...

If we consider the atmospheric pressure to be 'P_s' and the internal pressure to be 'P_i' then the net pressure would be written as

P_net = P_i - P_a

Now, as the radius of the drop has increased, work has been done by the net internal pressure. 

So, this work done will be

W = force x displacement

or

W = (pressure x area) x displacement

and in this case

W = (pressure x area) x increase in drop radius

so, the work done will be

W = (P_net x 4?r²) x dr  (1)

now, the increase in surface area of the liquid drop due to increase in radius (on account of the net internal pressure will be)

dA = final surface area of the drop - initial surface area of the drop 

or

dA = 4?(r + dr)² -  4?r²

or

dA = 4?r² + 2.4?r.dr + dr² - 4?r²

here we will ignore the term 'dr²' as it will be very small to consider (this will have minimal effect on the answer)

by solving further, we get

the increase in surface will be

dA = 8?r.dr  (2)

The potential energy (of the drop) will change (increase) on account of the change (increase) in its radius .

so, we also have to calculate the increase in potential energy of the drop (and it equals work done).

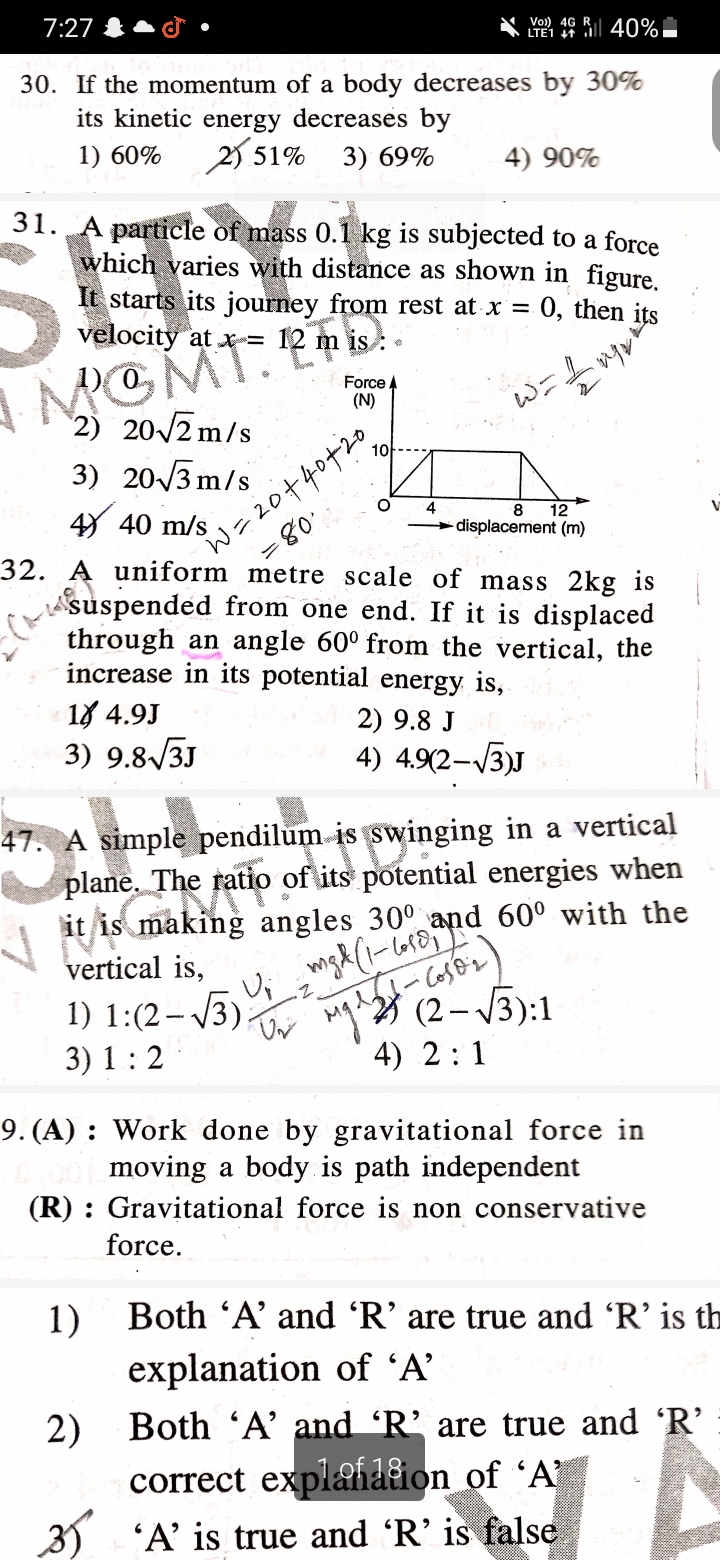
thus,

increase in potential energy = increase in surface area x surface tension (S)

or

dU = dA x S

or

the increase in potential energy would be 

dU = 8?r.dr x S  (3)

now, for a stable condition, the work done will equal the change (increase) in potential energy of the drop

thus,

W = dU

or

from equations (1) and (3), we get

(P_net x 4?r²) x dr = 8?r.dr x S  

or

P_net = (8?r.dr x S) / (4?r² x dr) 

or

the net pressure inside the drop will be 

P_net =  2S / r 

which is the required expression.