CBSE Class 11-science Answered
The flow of fluid through a pipe of uniform (circular) cross-section is known as HagenPoiseuille flow. The equations governing the HagenPoiseuille flow can be derived directly from the NavierStokes equations in cylindrical coordinates by making the following set of assumptions:
- The flow is steady (
 ).
). - The radial and swirl components of the fluid velocity are zero (
 ).
). - The flow is axisymmetric (
 ) and fully developed (
) and fully developed ( ).
).
Then the second of the three NavierStokes momentum equations and the continuity equation are identically satisfied. The first momentum equation reduces to  , i.e., the pressure
, i.e., the pressure  is a function of the axial coordinate
is a function of the axial coordinate  only. The third momentum equation reduces to:
only. The third momentum equation reduces to:
 where
where 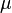 is the dynamic viscosity of the fluid.
is the dynamic viscosity of the fluid.- The solution is

Since  needs to be finite at
needs to be finite at  ,
,  . The no slip boundary condition at the pipe wall requires that
. The no slip boundary condition at the pipe wall requires that 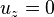 at
at  (radius of the pipe), which yields
(radius of the pipe), which yields
Thus we have finally the following parabolic velocity profile:
The maximum velocity occurs at the pipe centerline ( ):
):
The average velocity can be obtained by integrating over the pipe cross section: