CBSE Class 11-science Answered
In the arrangement shown in the figure, a heavy pulley of mass M is suspended from a spring of stiffness constant K and the mass m is suspended from a string tightly wound on the pulley. If the mass m is given a small downward displacement and released, find the time period of small oscillations (Take pulley as Disc) and string does not slips on the pulley.
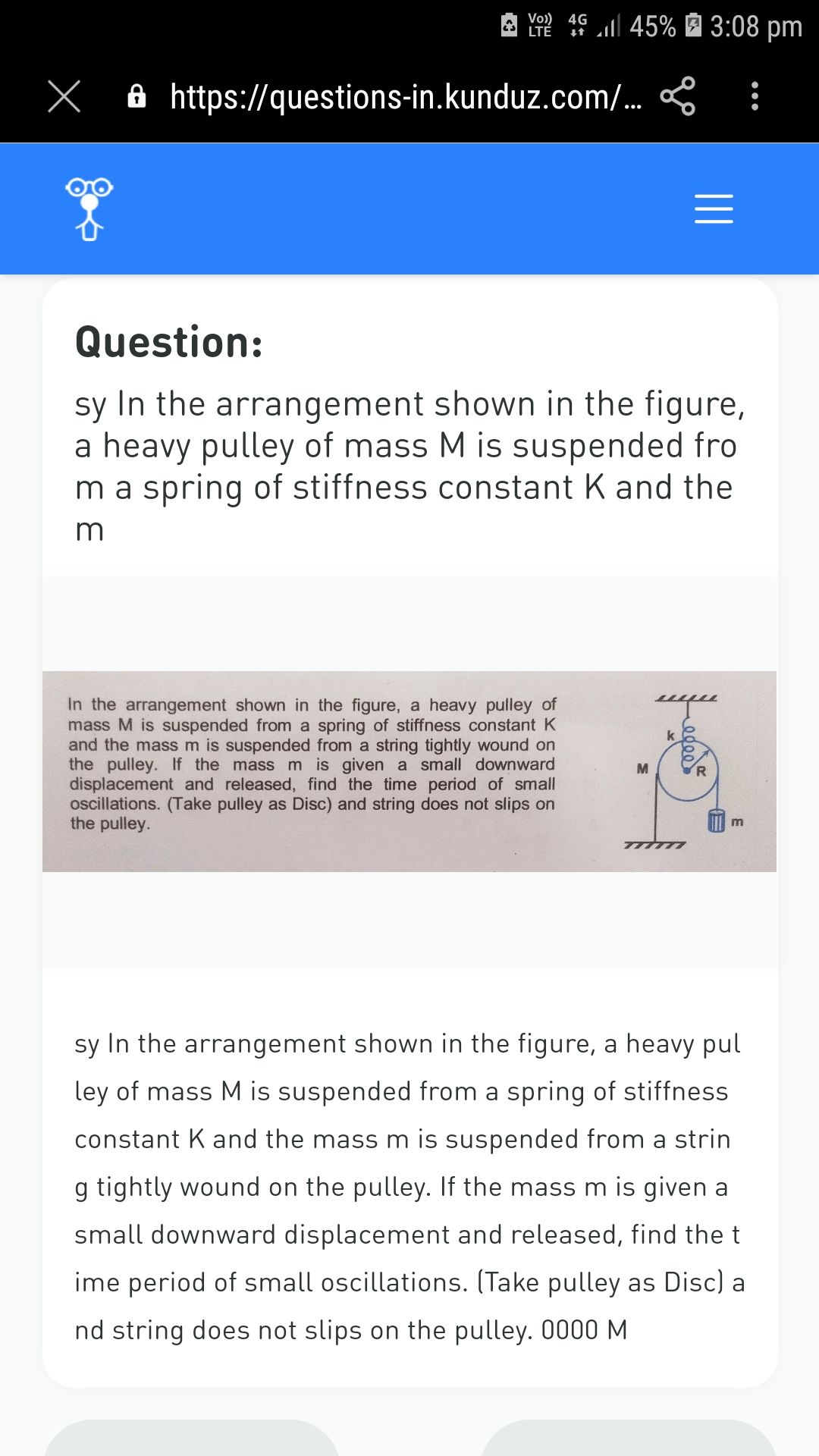
Asked by alkrishna4652 | 24 Feb, 2022, 13:46: PM
Expert Answer
Free body diagrams for pulley of mass M and hanging mass m are shown above.
String does not slip through pulley and pulley gets rotational motion .
If pulley rotates in clockwise direction there is a net force (T1 - T2 ) gives the required torque,
where T1 is the tension force in part of the string between pulley and mass.
T2 is the tension force in part of the string between puleey and fixed point of string.
Let us consider the mass is pulled down slightly and released so that oscillating motion of
small amplitude is set up in the system.
If we apply Newton's law to hanging mass m , we get
( m g ) - T1 = - m (2a ) ............................(1)
where 2a is acceleration of hanging mass m, if acceleration of translational motion of pulley is a
( for a displacement x of pulley , displacement of hanging mass is 2x due to constant length of string.
Similarly acceleration of hanging mass is twice of acceleration of pulley )
If we apply Newton's law to rotational motion of pulley , we get
( T1 - T2 ) R = I α
LHS of above expression is torque acting on pulley .
I is moenet of inertia and α is angular acceleration of rotational motion.
By considering pulley as a disc of mass M and radius R, above expression is written as
( T1 - T2 ) R = (1/2) M R2 ( a / 2R )
Above expression is simplified as , ( T1 - T2 ) = ( 1/4 ) M a .........................(2)
If we apply Newton's law to translational motion of pulley , we get
T1 + T2 + ( M g ) - kx = ( M a ) ............................. (3)
By substituting for tension forces T1 and T2 using eqn.(1) and (2) ,
eqn.(3) is written for acceleration a of pulley as
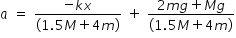.............................(4)First term in RHS is due to oscilatory motion and second term in RHS is due to damping.
From the first term of oscillatory motion, we get angular frequency ω as
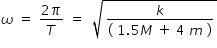
where T is period of oscillatory motion
Hence period T of oscillatory motion is given as
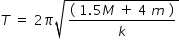
Answered by Thiyagarajan K | 25 Feb, 2022, 09:26: AM
Concept Videos
-
Free Body Problems
This video explains applications of a free body diagram in physics and tips... -
Free Body Problems
This video describes the functioning of an Atwood machine which is an examp... -
Free Body Problems
This video contains practice questions and numerical problems on the mechan...
CBSE 11-science - Physics
Asked by bhavishyapal41 | 23 Sep, 2024, 22:44: PM
ANSWERED BY EXPERTCBSE 11-science - Physics
Asked by pc2091054 | 28 May, 2024, 22:03: PM
ANSWERED BY EXPERTCBSE 11-science - Physics
Asked by sakethrockzz007 | 14 Aug, 2023, 16:30: PM
ANSWERED BY EXPERT
CBSE 11-science - Physics
Asked by alkrishna4652 | 24 Feb, 2022, 13:46: PM
ANSWERED BY EXPERTCBSE 11-science - Physics
Asked by unknownpass66 | 04 Jul, 2021, 11:28: AM
ANSWERED BY EXPERTCBSE 11-science - Physics
Asked by donehacking97 | 22 Dec, 2020, 06:09: AM
ANSWERED BY EXPERT
CBSE 11-science - Physics
Asked by lovemaan5500 | 23 Jan, 2019, 20:17: PM
ANSWERED BY EXPERT