NEET Class neet Answered

How to solve this question?

Asked by anshadali111 | 07 May, 2021, 04:08: PM

Expert Answer

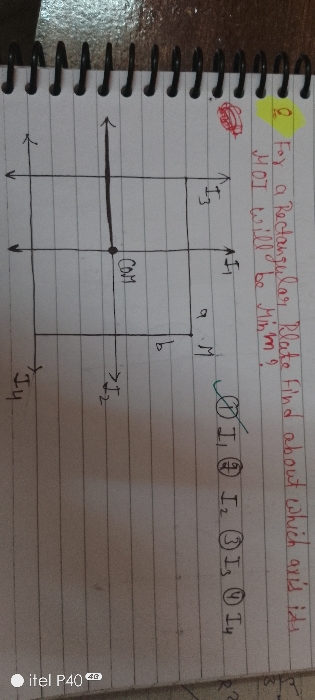
Figure shows an equilateral triangle OAB and axis of rotation passes through a corner O.

Moment of inertia I of system about the given axis of rotation is given as

I = I_AB + I_OA + I_OB

Where I_AB Is moment of inertia of rod AB . Similarly moment of inertia of other two rods are considered.

Since thin rod AB is symmetrically placed about axis of rotation, we have

I_AB = M × [ ( √3 /2 ) L ]²= (3/4) M L²

Moment of inertia I_OA of rod OA is determined as follows

Let ρ be the linear density of rod , ρ = ( M / L ) .

Let us consider small element of length dl in the rod at a distaance l along the rod from O as shown in figure.

Moment of inertia dI of this small element , dI = dm (l cos30)² .

Moment of inertia of rod of full length OA is determined as

begin mathsize 14px style I subscript O A end subscript space equals space integral subscript 0 superscript L d m space r squared space equals space integral subscript 0 superscript L rho d l space left parenthesis space l space cos 30 space right parenthesis squared space equals space 3 over 4 rho integral subscript 0 superscript L l squared space d l space equals space 1 fourth open parentheses rho L close parentheses space L squared space equals space 1 fourth M L squared end style

Similarly we get , I_OB = (1/4) M L²

I = I_AB + I_OA + I_OB = M L²[ (3/4) + (1/4) + (1/4) ] = (5/4) M L²

Answered by Thiyagarajan K | 07 May, 2021, 06:18: PM

Please Login or Sign up to continue!