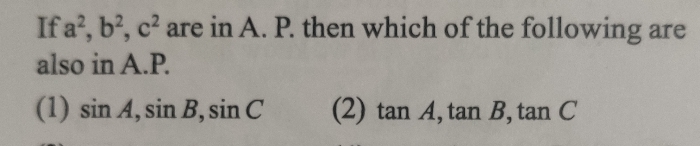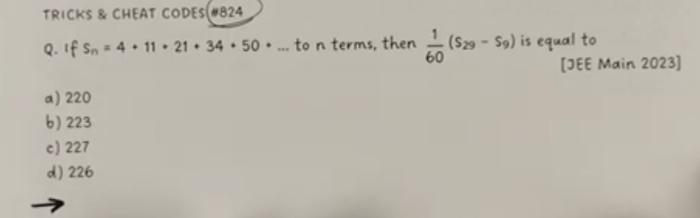JEE Class main Answered
(x-1)/(x+1) + (1/2)(x^2-1)/(x+1)^2 + (1/3)(x^3-1)/(x+1)^3+ ... infinity
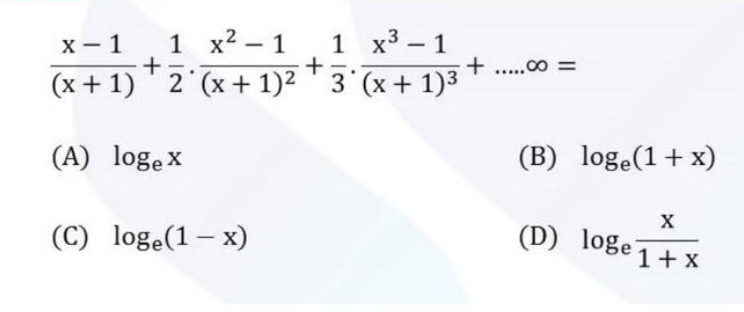
Asked by apoorv.bhardwaj100 | 30 Oct, 2022, 08:06: AM

Above series can be written as , S = S1 - S2
where 

and 

Let  and
and 
 and
and 
Then we get


Sum of series S1 and S2 are written as


Answered by Thiyagarajan K | 20 Dec, 2022, 05:25: PM
JEE main - Maths
Asked by vishwajeetsinghrajput2018 | 17 Mar, 2024, 12:27: AM
JEE main - Maths
Asked by sabeenashaik575 | 09 Jan, 2024, 09:53: AM
JEE main - Maths
Asked by kunmunkumar08032008 | 08 Oct, 2023, 01:15: PM
JEE main - Maths
Asked by nitinsolanki14102001 | 16 Sep, 2023, 03:01: PM
JEE main - Maths
Asked by joanmaria916 | 08 Jul, 2023, 04:37: PM
JEE main - Maths
Asked by vishnuramrs07 | 30 May, 2023, 09:05: PM
JEE main - Maths
Asked by chiyalaxmi28 | 18 Mar, 2023, 10:34: AM
JEE main - Maths
Asked by thakuranurag0987 | 27 Dec, 2022, 02:31: PM