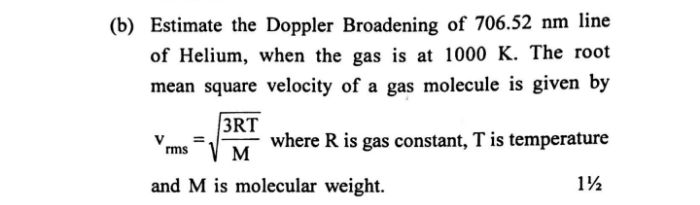
JEE Class main Answered

YDSE

Asked by swayamagarwal2114 | 17 Jul, 2022, 12:05: PM

Expert Answer

Part (i)

Light ray incident on slits S1 and S2 at angle α = (1.8/π)^o .

Hence additional phase Δφ₂at S₂ = ( d sinα ) × ( 2π / λ )

additional phase Δφ₂ at S₂calculated from above expression is

Δφ₂= 10^-3 × sin (1.8/π) × [ 2π / ( 6000× 10^-10 ) ] = (100/3)π

After slit S₁ , there is a transparent film of thickness t and refractive index μ .

hence phase difference Δφ₁ between light rays emerging from S₁ and S₂ is

Δφ₁= ( μ - 1 ) t × ( 2π / λ ) = (1/2) × 4 × 10^-6 × [ 2π / ( 0.6 × 10^-6 ) ]

Δφ₁= (20/3)π

Net phase difference = Δφ₂ - Δφ₁ = (80/3)π

Let I_o be the intensity of light emerging from slit S₁ . Thin film absorbs 50% intensity.

Hence after thin film light intensity ( I_o / 2 )

Width of slit S₂ is twice of that of S₁ . hence light intensity from slit S₂ is ( 2 I_o )

when two light rays of intensities I₁ and I₂ are added , we get resultant intensity I_R as

I_R = I₁ + I₂ + 2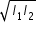

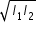begin mathsize 14px style square root of I subscript 1 I subscript 2 end root end style

cosΔφ

where Δφ is the phase difference between two light rays.

---------------------------------

Part (i)

Intensity at centre point of screen = (I_o/2) + 2I_o + 2 [ (I_o/2)(2I_o) ]^1/2 cos [ (80/3)π ) = (3/2) I_o

Maximum intensity occurs at a point where phase difference is zero

Hence Maximum intensity = (I_o/2) + 2I_o + 2 [ (I_o/2)(2I_o) ]^1/2 = (9/2) I_o

If we denote maximum intensity (9/2) I_o= I ,

then Intensity at centre point of screen = { [ (3/2) I_o ] / [ (9/2) I_o ] } × I = (1/3) I

-------------------------

Part (ii)

Minimum intensity is when phase difference Δφ = π

Mimimum intensity = (I_o/2) + 2I_o + 2 [ (I_o/2)(2I_o) ]^1/2 cos [ π ] = I_o/2

If we denote maximum intensity (9/2) I_o= I ,

then Intensity at centre point of screen = { [ (1/2) I_o ] / [ (9/2) I_o ] } × I = (1/9) I

----------------------------------------

Part (iii)

Maxima of intensity occure at angular position θ_n , if following condition is satisfied

Δp + ( d sinθ_n ) = nλ

where Δp is initial phase difference at slits position.

Let y_nbe the vertical distance from O on screen for n-th maximum, then we have

Δp + ( d × (y_n/D ) = nλ .......................... (1)

Let y_n+1be the vertical distance from O on screen for (n+1)-th maximum, then we have

Δp + ( d × (y_n+1/D ) = (n+1)λ ......................(2)

By subracting eqn.(1) from eqn.(2) , we get

(d/D) [ y_n+1 - y_n ] = λ

Hence fringe width = [ y_n+1 - y_n ] = ( λ D ) /d = ( 6000 × 10^-10 ) / 10^-3 = 6 × 10^-4 m

fringe width = 0.6 mm

-----------------------------------------------------

Part (iv)

we have seen from Part (i) ,Net phase difference at O = Δφ₂ - Δφ₁ = (80/3)π

Net phase difference at O = [13+ (1/3)]2π

To get phase difference as integer multiples of 2π ,

required additional phase difference = (2/3) (2π ) = (4/3)π

Hence required path difference = (4/3)π × ( λ / 2π ) = (2/3) λ

angular position θ to get this additional phase difference is determined from the following equation

d sinθ ≈ d tanθ = d × (y/D) = (2/3)λ

where y is distance of nearest maximum from O and D is slit-to-screen distance

y = [ (2/3) × 6000 × 10^-10 ] / ( 10^-3 ) = 4 × 10^-4 m = .4 mm

Hence nearest maximum from O is at adistance y = 0.4 mm

-------------------------------

part (v)

Central maximum occurs when phase difference between two rays emerging from slits S₁ and S₂ is zero.

we have seen from Part (i) ,Net phase difference at O = Δφ₂ - Δφ₁ = (80/3)π

Equivalenet path difference = (80/3)π × ( λ / 2π ) = (40/3) λ

To reduce above path difference , required angular position θ is determined from the following expression

d sin

begin mathsize 14px style theta end style

begin mathsize 14px style almost equal to end style

d tan

begin mathsize 14px style theta end style

= (40/3) λ

d tan

begin mathsize 14px style theta end style

= d ( y / D ) = (40/3) λ

y = (40/3d) λ = [ 40 / ( 3 × 10^-3 ) ] ( 6000 × 10^-10 ) = 8 × 10^-3 m = 8 mm

Hence central maximum occurs at a point 8 mm above O

-------------------------------------------------------------------

part ( vi )

Path difference at a distance 4 mm above O is determined as

Δp = d sinθ ≈ d tanθ = 10^-3 × (4/1000) = 4 × 10^-6 m

Equivalent phase difference = 4 × 10^-6 × ( 2π / λ ) = 4 × 10^-6 × [ 2π / ( 6000 × 10^-10 ) ]

Equivalent phase difference =(40/3)π

Phase didfference at a distance 4 mm above O = (80/3)π - (40/3)π = (40/3)π

I_R = I₁ + I₂ + 2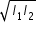

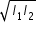begin mathsize 14px style square root of I subscript 1 I subscript 2 end root end style

cosΔφ

(I_o/2) + 2I_o + 2 [ (I_o/2)(2I_o) ]^1/2 cos [ (40/3)π ) = (3/2) I_o

If we denote maximum intensity (9/2) I_o= I ,

then Intensity at 4 mm above O = { [ (3/2) I_o ] / [ (9/2) I_o ] } × I = (1/3) I

-----------------------------------------------------------------------------------------------

Answered by Thiyagarajan K | 17 Jul, 2022, 23:49: PM

Please Login or Sign up to continue!

JEE Class main Answered

Choose Filters

Open In Ask A Doubt App ?

Get in Touch