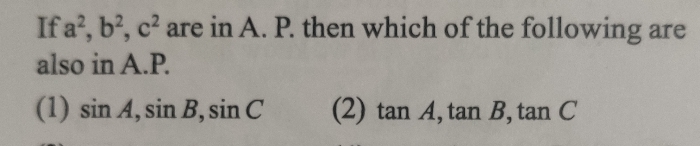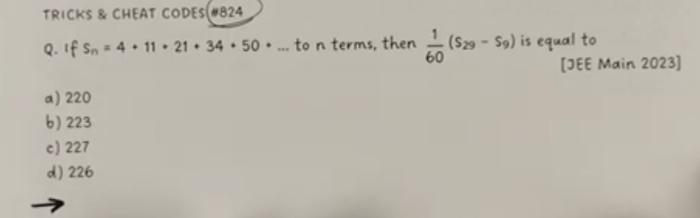Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

Please solve it
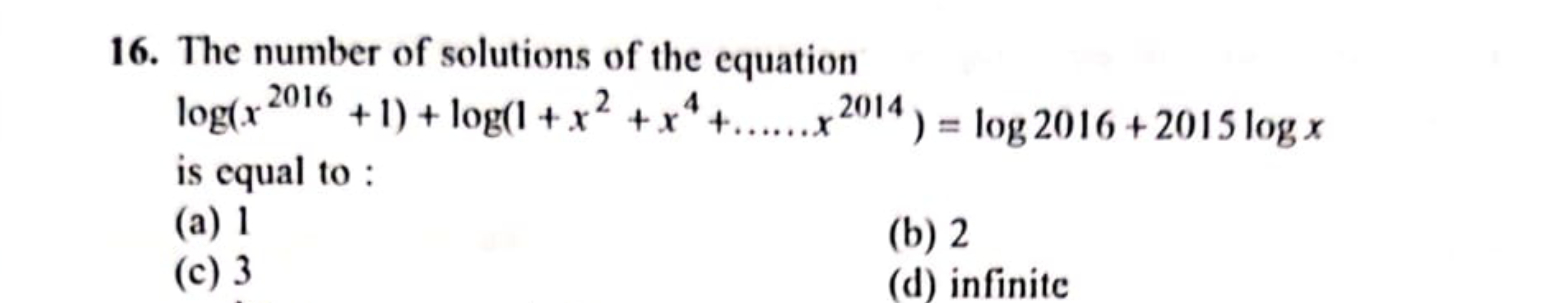
question image
Asked by amrit2002maurya | 09 Aug, 2020, 13:38: PM
answered-by-expert Expert Answer
Question: The number of solutions of the equation log(x2016 + 1)+log(1+x2+x4+...+x2014)=log(2016) + 2015 log x
Solution:
log open parentheses straight x to the power of 2016 plus 1 close parentheses plus log open parentheses 1 plus straight x squared plus straight x to the power of 4 plus.... plus straight x to the power of 2014 close parentheses equals log space 2016 space plus space 2015 space log space straight x rightwards double arrow log space open square brackets open parentheses straight x to the power of 2016 plus 1 close parentheses open parentheses 1 plus straight x squared plus straight x to the power of 4 plus.... plus straight x to the power of 2014 close parentheses close square brackets equals log space 2016 space plus space log space straight x to the power of 2015 rightwards double arrow log space open square brackets open parentheses straight x to the power of 2016 plus 1 close parentheses open parentheses 1 plus straight x squared plus straight x to the power of 4 plus.... plus straight x to the power of 2014 close parentheses close square brackets equals log space open parentheses 2016 space straight x to the power of 2015 close parentheses rightwards double arrow open parentheses straight x to the power of 2016 plus 1 close parentheses open parentheses 1 plus straight x squared plus straight x to the power of 4 plus.... plus straight x to the power of 2014 close parentheses equals 2016 space straight x to the power of 2015 rightwards double arrow 1 plus straight x squared plus straight x to the power of 4 plus.... plus straight x to the power of 2014 plus straight x to the power of 2016 plus straight x to the power of 2018 plus straight x to the power of 2020 plus.... plus straight x to the power of 4030 equals 2016 space straight x to the power of 2015 rightwards double arrow fraction numerator open parentheses straight x squared close parentheses to the power of 2016 minus 1 over denominator straight x squared minus 1 end fraction equals 2016 space straight x to the power of 2015 space space space.... space Sum space of space straight a space finite space straight G. straight P. rightwards double arrow fraction numerator straight x to the power of 4032 minus 1 over denominator straight x squared minus 1 end fraction equals 2016 space straight x to the power of 2015 Left space hand space side space has space even space power space of space straight x space wherere space as space right space hand space side space has space odd space power So comma space no space solution space exist space for space this space equation But space as space log open parentheses 1 to the power of 2016 plus 1 close parentheses plus log open parentheses 1 plus 1 squared plus 1 to the power of 4 plus.... plus 1 to the power of 2014 close parentheses equals log space 2 space plus space log space 1007 space equals space log space 2016 space equals space log space 2016 space plus space 2015 space log space 0 Thus comma space straight x equals 1 space satisfies space the space given space equations Hence comma space there space is space only space one space solution space exist space for space the space given space equation.
Answered by Renu Varma | 09 Aug, 2020, 15:52: PM
Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Maths
the sum of infinite series 1/2(1/3+1/4)-1/4(1/3^2+1/4^2)+1/6(1/3^3+1/4^3)+
Asked by pritamlovevanshi5 | 15 Sep, 2024, 08:24: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
s=1+1/3+1/9+1/27 value of s is
Asked by vishalsarap0022 | 19 May, 2024, 10:30: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
23,47,1p156 relation
Asked by obravickykumar50 | 07 May, 2024, 09:27: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
solv
question image
Asked by vishwajeetsinghrajput2018 | 17 Mar, 2024, 00:27: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
solve
question image
Asked by mailtome.aj86 | 26 Feb, 2024, 20:29: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
the value 21/4. 41/8. 81/6..... is
Asked by sabeenashaik575 | 09 Jan, 2024, 09:53: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
solution
question image
Asked by 20sanjaykoley20 | 19 Dec, 2023, 00:57: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
Let S be the sum P the product and R the sum of reciprocal
Asked by kunmunkumar08032008 | 08 Oct, 2023, 13:15: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
Let Tr be the rth term of an AP for r=1,2,3,.... If for some positive integers m, n. We have Tm=1/n and Tn=1/m, then Tmn equals to
question image
Asked by nitinsolanki14102001 | 16 Sep, 2023, 15:01: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
if an= -2/(4n^2)-(16n)+(15),      [(4n^2)-(16n)+(15) dis entire thing is the denominator)] then: opt a) a1+a2+....+a10= 20/51 opt b) a1+a2+.....a10=30/51 opt c) a1+a2+......a25=50/141 opt d) a1+a2+.....a25=60/141 Its more than one ans type q, pls tell me a detailed explanation on how to do it as i din understand 
Asked by joanmaria916 | 08 Jul, 2023, 16:37: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt