Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 12-science Answered

a thin stick of length f/5 is placed along principle axis of a concave mirror of focal length f such that it's image which is real and elongated just touches the stick .what is the magnification
Asked by amishavasave56.12dgatl | 27 Aug, 2021, 17:25: PM
answered-by-expert Expert Answer
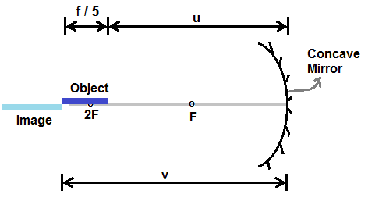
Let u be the distance between pole of concave mirror and front edge of the rod.
It is given that real and elongated image is obtained . Hence the front edge of the rod is
between F and 2F. Since it is given that image touches the rod , mirror-to-image distance
is ( u + ( f/5) ] as shown in figure because length of rod is f/5 .
 
we have mirror equation,  (1/v) + (1/u) = 1/f
 
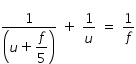
begin mathsize 14px style fraction numerator 1 over denominator open parentheses u plus begin display style f over 5 end style close parentheses end fraction space plus space 1 over u space equals space 1 over f end style
From above expression , we get a quadratic equation , 5 u2 - 9f u - f2 = 0
 
From above quadratic expression we express u in terms of f as , u = 1.9 f
 
Magnification M = v / u 
 
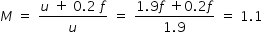
begin mathsize 14px style M space equals space fraction numerator u space plus space 0.2 space f over denominator u end fraction space equals space fraction numerator 1.9 f space plus 0.2 f over denominator 1.9 end fraction space equals space 1.1 end style
Answered by Thiyagarajan K | 28 Aug, 2021, 08:33: AM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 12-science - Physics
an object 2cm high is placed at a distance of 16cm from a concave mirror which produces a virtual image 3cm high what is the focal length of the mirror ?
Asked by pushpamagadum21 | 09 Jul, 2024, 20:43: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
a thin stick of length f/5 is placed along principle axis of a concave mirror of focal length f such that it's image which is real and elongated just touches the stick .what is the magnification
Asked by amishavasave56.12dgatl | 27 Aug, 2021, 17:25: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
Sign convention in concave mirror
Asked by raushankumarmushkipur | 02 Apr, 2021, 10:08: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
When a person is moving with a constant speed the speed of his/her image appears to increase substantially as he/she moves closer to the mirror as noticed by a person sitting in a stationary car. Why? 
Asked by prakriti12oct | 11 Feb, 2020, 14:48: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
Use the mirror equation to deduce that The virtual image produced by a convex mirror is always diminished in size.  
Asked by prakriti12oct | 27 Jun, 2019, 02:20: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
Derive mirror formula for concex mirror.
Asked by polyrelation | 15 Mar, 2019, 16:42: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
A convex mirror of radius of curvature 70 cm and a plane mirror are placed facing each other. The distance between them is 28 cm.  A point object is put midway between them on the principle axis of the mirror. Two images of the object are seen in the plane mirror. Find their distances from the plane mirror. 
Asked by mridulabarua05 | 11 Feb, 2019, 13:10: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
1. The interior of a hollow metal sphere is polished to serve as a mirror. At a point 12cm from the centre of the sphere of radius 24 cm, a point source of light is placed. Locate its image formed by two successive reflections, the first reflection taking place at the  (a) far wall (b) near wall.  2. And image produced by a concave mirror is one fourth the size of the object.  If the object is moved 10 cm towards the mirror, its image will be only half the size of the object. Calculate the focal length of the mirror. 
Asked by mridulabarua05 | 07 Feb, 2019, 09:10: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
A point luminous source P is placed on the principle axis of a concave mirror of radius of radius of curvature 1m at a distance of 75 cm from its pole. At what distance from the concave mirror should a plane mirror be set so that the rays from P, after reflection from the concave mirror and then from the plane mirror again converge at the point P? Does the point of convergence of the rays change if they are first reflected at the plane mirror and then at the concave mirror?
Asked by mridulabarua05 | 02 Feb, 2019, 11:23: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Physics
derive mirror formula  
Asked by Aadeshveersingh03 | 07 Jan, 2019, 07:57: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt