CBSE Class 12-science Answered
A plane which remains at a constant distance 3p from the origin cuts the co-ordinate axes at A, B and C. Show that the locus of the centroid of triangle ABC is x-2 + y-2 + z-2 = p-2.
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
Let a, b, c are the intercepts made by the plane with the co-ordinate axes, then the co-ordinates of A, B and C are (a, 0, 0), (0, b, 0) and (0, 0, c) respectively.
Then the equation of the plane is 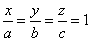 . …(1)
. …(1)
Also, the perpendicular distance of this plane from the origin is 3p.

Now, let (x, y, z) be the co-ordinates of the centroid of the triangle ABC, then
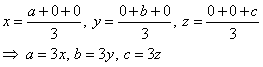
On putting the values of a, b and c in (2), we get
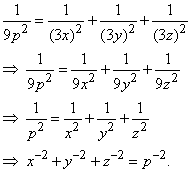
Answered by | 04 Jun, 2014, 15:23: PM
Concept Videos
CBSE 12-science - Maths
Asked by kishanradha7392 | 05 Jul, 2022, 16:26: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 27 Feb, 2015, 15:56: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM




