CBSE Class 12-science Answered

Monochromatic light.

Asked by alanpeter9611 | 18 Feb, 2019, 11:21: PM

Expert Answer

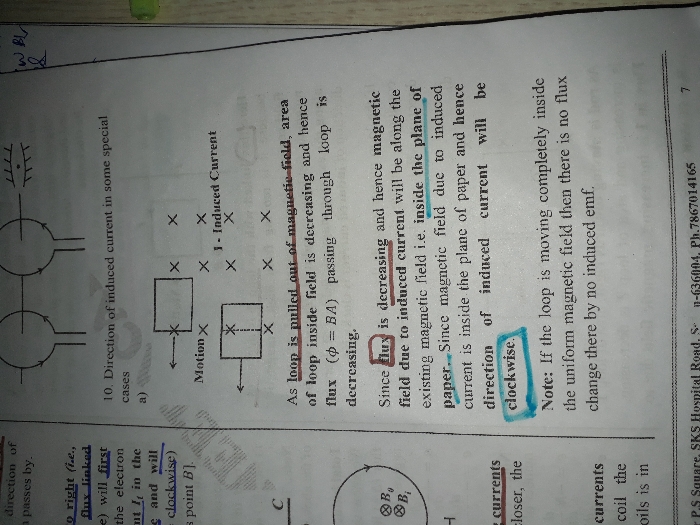
Figure shows a parallel beam of light falling normally on a single slit LN of width a. The diffracted light goes on to meet a screen.

The midpoint of the slit is M. A straight line through M perpendicular to the slit plane meets the screen at C. We want the intensity at any point P on the screen. Straight lines joining P to the different points L,M,N, etc., can be treated as parallel, making an angle θ with the normal MC.
The basic idea is to divide the slit into much smaller parts, and add their contributions at P with the proper phase differences. We are treating
different parts of the wavefront at the slit as secondary sources. Because the incoming wavefront is parallel to the plane of the slit, these sources
are in phase.
The path difference NP – LP between the two edges of the slit can be
calculated exactly as NP – LP = NQ as shown in figure

NQ = a sin θ ≈ aθ

Similarly, if two points M1 and M2 in the slit plane are separated by y, the path difference M₂P – M₁P ≈ yθ.

We now have to sum up equal, coherent contributions from a large number of sources, each with a different phase.

The main features of the diffraction pattern can be understood by simple arguments.

At the central point C on the screen, the angle θ is zero. All path differences are zero and hence all the parts of the slit contribute in phase.

This gives maximum intensity at C. Experimental observation shown in the above figure indicates that the intensity has a
central maximum at θ = 0 and other secondary maxima at θ ≈ (n+1/2) λ/a, and has minima (zero intensity) at θ ≈ nλ/a,
where n = ±1, ±2, ±3, ....

It is easy to see why it has minima at these values of angle. Consider first the angle θ where the path difference aθis λ.

Then, θ ≈ λ /a . .....................................(1)

Now, divide the slit into two equal halves LM and MN each of size a/2. For every point M₁ in LM, there is a point M₂ in MN such that
M₁M₂ = a/2. The path difference between M₁ and M₂ at P = M₂P – M₁P = θ (a/2) = λ/2 for the angle chosen.

This means that the contributions from M1 and M2 are 180º out of phase and cancel in the direction θ = λ/a.

Contributions from the two halves of the slit LM and MN, therefore, cancel each other.

Equation (1) gives the angle at which the intensity falls to zero. One can similarly show that the intensity is zero for θ = nλ/a,

with n being any integer (except zero!). Notice that the angular size of the central maximum increases when the slit width a
decreases.

It is also easy to see why there are maxima at θ (n + 1/2) ≈λ/a and why they go on becoming weaker and weaker with increasing n.

Consider an angle θ = 3λ/2a which is midway between two of the dark fringes. Divide the slit into three equal parts.

If we take the first two thirds of the slit, the path difference between the two ends would be

begin mathsize 12px style 2 over 3 a cross times theta space equals space 2 over 3 a cross times fraction numerator lambda over denominator begin display style open parentheses bevelled 2 over 3 close parentheses a end style end fraction space equals space lambda end style

The first two-thirds of the slit can therefore be divided into two halves which have a λ/2 path difference. The contributions of these two halves

cancel in the same manner as described earlier. Only the remaining one-third of the slit contributes to the intensity at a point between the two
minima. Clearly, this will be much weaker than the central maximum (where the entire slit contributes in phase).

One can similarly show that there are maxima at (n + 1/2) θ/a with n = 2, 3, etc.

These become weaker with increasing n, since only one-fifth, one-seventh, etc., of the slit contributes in these cases.

----------------------

Since First dark fringe occurs when semi-angle θ = λ/a, angular width of central maximum of bright fringe 2λ/a

Second dark fringe occurs when semi-angle θ = 2λ/a, angular width of first maximum of bright fringe 4λ/a which is twice of

angular width of central maximum

Answered by Thiyagarajan K | 20 Feb, 2019, 12:03: PM