Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

solve
question image
Asked by sarveshvibrantacademy | 07 Apr, 2019, 11:24: AM
answered-by-expert Expert Answer
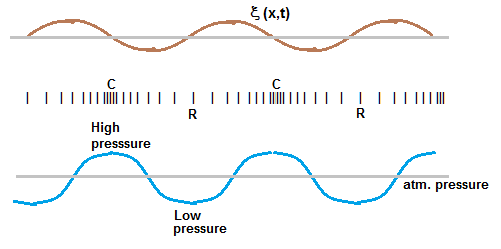
Figure shows the longitudinal displacement variation of air particles along the wave propagation direction (x-axis).
Corresponding pressure variation also is shown in figure.
 
(A) |∂ξ /∂x|C = |∂ξ /∂x|R .  This is true.  At maximum compression point the slope is decreasing. At maximum rarifaction point slope is increasing.
                                            But the magnitude of rate of change of displacement with respect to distance is same. This can be seen from figure.
 
(B) |∂ξ /∂t|C = |∂ξ /∂t|R = 0 . This is true. At the compression and rarifaction points, displacement of air particles is almost nil. 
                                               Hence time variation of displacement at these points also zero.
 
(C) PC - PR = 2 |∂ξ /∂x|C × Bulk-Modulus . This true as per the explanation given below
                         Let  PC - PR = ΔP  , change in pressure at compression point C.
                         
                         2 ∂ξ is the net displacement of air particles around compression point C.
                         if we consider an area ΔA perpendicular to the longitudinal motion,
                          then (2 ∂ξ )ΔA is change in volume ΔV.   original volume of air particles is ∂xΔA

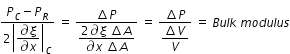
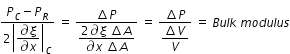
                          Hence begin mathsize 12px style fraction numerator P subscript C minus P subscript R over denominator 2 open vertical bar begin display style fraction numerator partial differential xi over denominator partial differential x end fraction end style close vertical bar subscript C end fraction space equals space fraction numerator increment P over denominator begin display style fraction numerator 2 partial differential xi space increment A over denominator partial differential x space increment A end fraction end style end fraction space equals space fraction numerator increment P over denominator begin display style fraction numerator increment V over denominator V end fraction end style end fraction space equals space B u l k space m o d u l u s end style
 
 
(D) Particles of air are stationary between C and R - False
       Maximum displacement occurs at mid point of C and R. Hence particles of air are not stationary
Answered by Thiyagarajan K | 07 Apr, 2019, 21:05: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt