Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

Please solve the question 
question image
Asked by anshuman.anshuman090 | 30 Oct, 2019, 12:24: PM
answered-by-expert Expert Answer
To get the velocity of charge at surface of charged sphere, we equate the change in potential energy of charge from point P
to a point at surface of spher to change in kinetic energy of charge from point P to the point on the surface of sphere.
 
change in potential energy  ΔU is given by
 
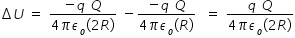begin mathsize 14px style increment U space equals space fraction numerator negative q space Q over denominator 4 pi epsilon subscript o left parenthesis 2 R right parenthesis end fraction space minus fraction numerator negative q space Q over denominator 4 pi epsilon subscript o left parenthesis R right parenthesis end fraction space space equals space fraction numerator q space Q over denominator 4 pi epsilon subscript o left parenthesis 2 R right parenthesis end fraction end style  .............................(1)
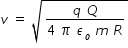
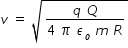
hence velocity at a point on surface of charged sphere :-  ΔU = (1/2)mv2 or velociy  begin mathsize 14px style v space equals space square root of fraction numerator q space Q over denominator 4 space pi space epsilon subscript o space m space R end fraction end root end style 
---------------------------------------------------------------
 
Change in potential from a point on surface of charged sphere to centre of sphere is calculated as follows
 
Electric field Er = - [ qQ / (4πεo) ] ( r/R3)
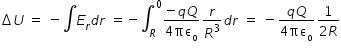
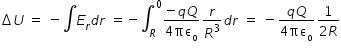
potential difference begin mathsize 14px style increment U space equals space minus integral E subscript r d r space equals negative integral subscript R superscript 0 fraction numerator negative q Q over denominator 4 πε subscript straight o end fraction r over R cubed d r space equals space minus fraction numerator q Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator 2 R end fraction end style
Hence if we equate change in potential energy to change in kinetic energy
 
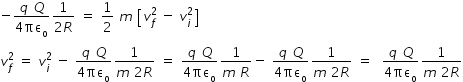
begin mathsize 14px style negative fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator 2 R end fraction space equals space 1 half space m space open square brackets v subscript f superscript 2 space minus space v subscript i superscript 2 close square brackets v subscript f superscript 2 space equals space v subscript i superscript 2 space minus space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction space equals space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space R end fraction minus space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction space equals space space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction end style
 
Hence velocity of charge at centre ,
 
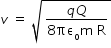
begin mathsize 14px style v space equals space square root of fraction numerator q Q over denominator 8 πε subscript straight o straight m space straight R end fraction end root end style

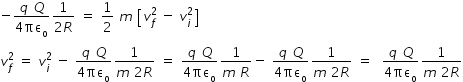
begin mathsize 14px style negative fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator 2 R end fraction space equals space 1 half space m space open square brackets v subscript f superscript 2 space minus space v subscript i superscript 2 close square brackets v subscript f superscript 2 space equals space v subscript i superscript 2 space minus space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction space equals space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space R end fraction minus space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction space equals space space fraction numerator q space Q over denominator 4 πε subscript straight o end fraction fraction numerator 1 over denominator m space 2 R end fraction end style
Answered by Thiyagarajan K | 01 Nov, 2019, 13:48: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt