Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

CBSE Class 11-science Answered

Find the distance between the lines 4x-3y+5=0 and 8x-6y+7=0..
Asked by Suryavanshikatoch | 11 Feb, 2019, 19:37: PM
answered-by-expert Expert Answer

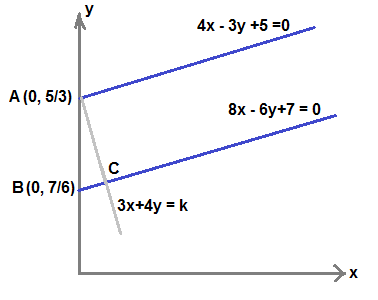
 lines 4x-3y+5=0 and 8x-6y+7 =0 are parallel lines with slope 4/3 as shown in figure.

Point of intersection of both lines with y-axis is obtained by substituting x=0 in the equations.
 
Points of intersection A (0, 5/3) and B(0,7/6) are shown in figure.
 
Perpendiculr line to both the lines is in the form 3x+4y = k . This perpendicular line passes through A(0, 5/3)
by substituting values for x and y in the equation we get k = 20/3
 
point of intersection is obtained by solving the equations 8x-6y+7=0  and 3x+4y = (20/3)
 
we get point of intersection as C ( 0.24, 1.5)
 
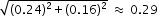
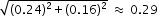
distance between the lines = begin mathsize 12px style square root of open parentheses 0.24 close parentheses squared plus open parentheses 0.16 close parentheses squared end root space almost equal to space 0.29 end style
 
Answered by Thiyagarajan K | 11 Feb, 2019, 22:24: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 11-science - Maths
if log 1/2 x greater than log 1/2 y then x greater than y
Asked by megaladevi07 | 24 Jul, 2024, 11:01: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
1+sinx+cosx/1+sinx-cosx=cotx/2
Asked by mihirkumarjha356 | 18 Jul, 2024, 21:51: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
find the circumcentre of (1,3) (0,-2) (-3,1)
Asked by vijaya198724 | 08 Jul, 2024, 22:33: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
if a = [[2, 3], [1, 4]] and b = [[5, 0], [3, 2]] then find a + b ?
Asked by shshnkmanjula | 08 Jul, 2024, 17:25: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
|x-3|+2|x+1|=4
Asked by harshdasisode | 04 Jul, 2024, 00:07: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
ax^2+bx+c=0 then x=
Asked by manjushakothavade1979 | 25 Jun, 2024, 10:29: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
prove using identities cos4xsin3x-cos2xsinx/sin4xsinx+cos6xcosx =tanx
Asked by ishanvi.pahil | 22 Jun, 2024, 22:31: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
answer
question image
Asked by jagathaiah.kamsali | 13 Jun, 2024, 20:20: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
find distance between two points A(5,-4) B(7,6)
Asked by nandhandheeraj7474 | 11 Jun, 2024, 17:23: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 11-science - Maths
3. Calculate the mean and standard deviation using short cut method for the following distribution Marks Frequency 20-30 3 30-40 6 40-50 13 50-60 15 60-70 14 70-80 5 80-90 4
Asked by nvprithica | 07 Jun, 2024, 11:53: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Are You a Teacher or a Parent Seeking Customised Tests? Click here
×