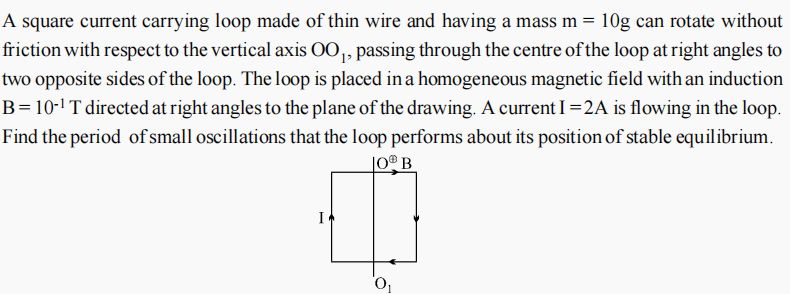
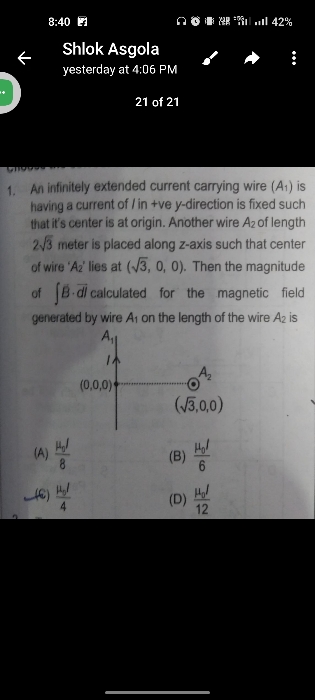
JEE Class main Answered
Find magnetic field Bp/Bc=?
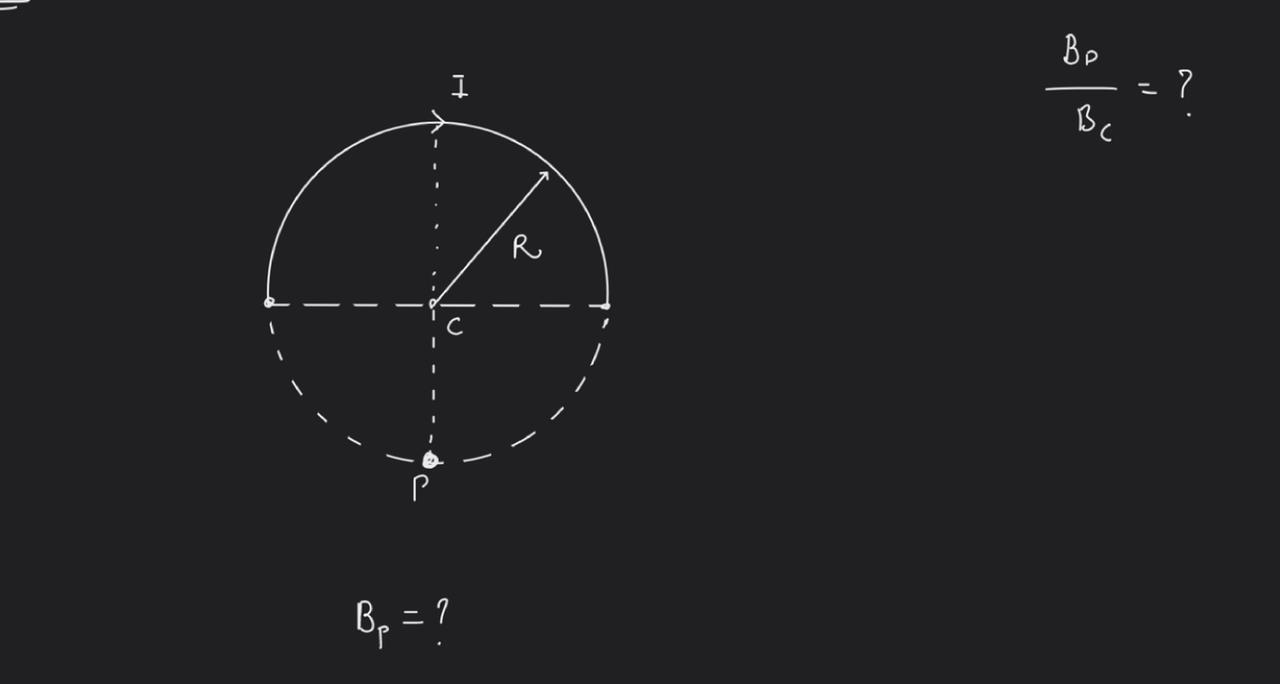
Asked by Vipulgautam050 | 27 Oct, 2021, 23:47: PM
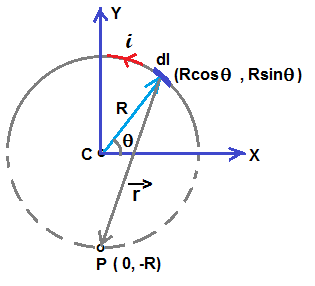
Let us consider a small current element of length dl at angular position θ as shown in figure.
magnetic field dB at P is given as
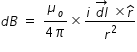 ...............................(1)
...............................(1)let us condier dl as arc length ( R dθ ), where dθ is angle subtended by dl at centre C
vector 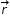 = ( -R cosθ )
= ( -R cosθ ) 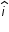 - ( R + R sinθ )
- ( R + R sinθ ) 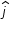
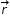 = ( -R cosθ )
= ( -R cosθ ) 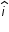 - ( R + R sinθ )
- ( R + R sinθ ) 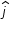
unit vector 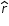 = (1/r ) [ ( -R cosθ )
= (1/r ) [ ( -R cosθ ) 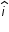 - ( R + R sinθ )
- ( R + R sinθ ) 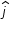 ]
]
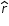 = (1/r ) [ ( -R cosθ )
= (1/r ) [ ( -R cosθ ) 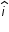 - ( R + R sinθ )
- ( R + R sinθ ) 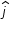 ]
]where r is magnitude of vector 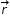
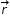
We get magnetic field dB from eqn.(1) as
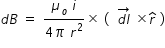 .........................(2)
.........................(2)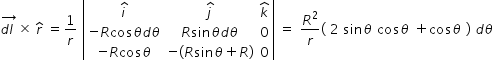
From above expression of ( dl ×  ) , we see that if we integrate ( dl ×
) , we see that if we integrate ( dl ×  ) from θ = 0 to θ = π
) from θ = 0 to θ = π
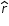 ) , we see that if we integrate ( dl ×
) , we see that if we integrate ( dl × 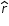 ) from θ = 0 to θ = π
) from θ = 0 to θ = π to get magnetic field due to full semicircular arc , we get zero
hence BP = 0
Magnetic field BC at centre is determined as

Answered by Thiyagarajan K | 28 Oct, 2021, 11:47: AM
JEE main - Physics
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
JEE main - Physics
Asked by mohammedimroz | 13 Apr, 2024, 21:48: PM
JEE main - Physics
Asked by aaryamanmodern | 14 Aug, 2023, 10:20: AM
JEE main - Physics
Asked by mc8may | 18 Jul, 2023, 22:21: PM
JEE main - Physics
Asked by aaryamanmodern | 15 Jul, 2023, 13:02: PM
JEE main - Physics
Asked by yashsanvordekar | 24 Jun, 2022, 22:42: PM
JEE main - Physics
Asked by manvirsingh2242 | 21 Jun, 2022, 10:20: AM
JEE main - Physics
Asked by arjunsah797 | 25 Jan, 2022, 09:31: AM
JEE main - Physics
Asked by arjunsah797 | 24 Jan, 2022, 16:14: PM