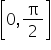CBSE Class 12-science Answered
Discuss the applicability of Rolle’s theorem for the following function on the indicated interval:
f(x) = |x| on [–1, 1]
Asked by Topperlearning User | 04 Aug, 2014, 15:57: PM
We have, f (x) = |x| = 
Since a polynomial function is everywhere continuous and differentiable. Therefore, f (x) is continuous and differentiable for all x < 0 and for all x > 0 except possibly at x = 0.
So, consider the point x = 0.
We have,
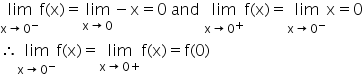
Thus, f (x) is continuous at x = 0
Hence, f (x) is continuous on [–1, 1]
Now,
(LHD at x = 0) = 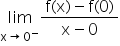
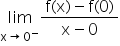
Þ (LHD at x = 0) = 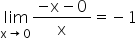 [Q f (x) = – x for x < 0 and f (0) = 0]
[Q f (x) = – x for x < 0 and f (0) = 0]
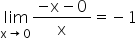 [Q f (x) = – x for x < 0 and f (0) = 0]
[Q f (x) = – x for x < 0 and f (0) = 0]and, (RHD at x = 0) = 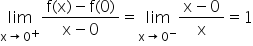 [Q f (x) = x for x ³ 0]
[Q f (x) = x for x ³ 0]
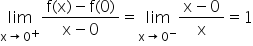 [Q f (x) = x for x ³ 0]
[Q f (x) = x for x ³ 0]\ (LHD at x = 0) ¹ RHD at x = 0
This shows that f (x) is not differentiable at x = 0 Î (– 1, 1). Thus, the condition of derivability at each point of (– 1, 1) is not satisfied.
Hence, Rolle’s theorem is not applicable to f (x) = |x| on [–1, 1]
Answered by | 04 Aug, 2014, 17:57: PM
Concept Videos
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 15:57: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 16:00: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 16:03: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 16:11: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Jun, 2014, 13:23: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 05 Aug, 2014, 08:38: AM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 15:32: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 15:48: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 16:16: PM
CBSE 12-science - Maths
Asked by Topperlearning User | 04 Aug, 2014, 16:35: PM





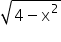 on [– 2, 2].
on [– 2, 2].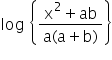 on [a, b], where 0 < a < b.
on [a, b], where 0 < a < b.