Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

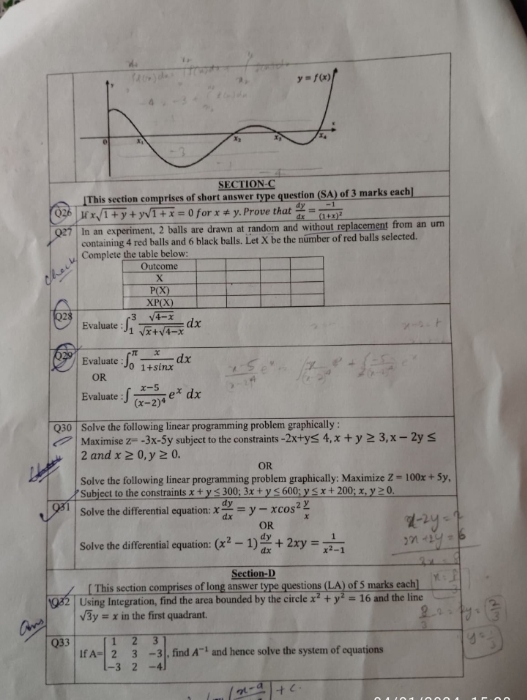
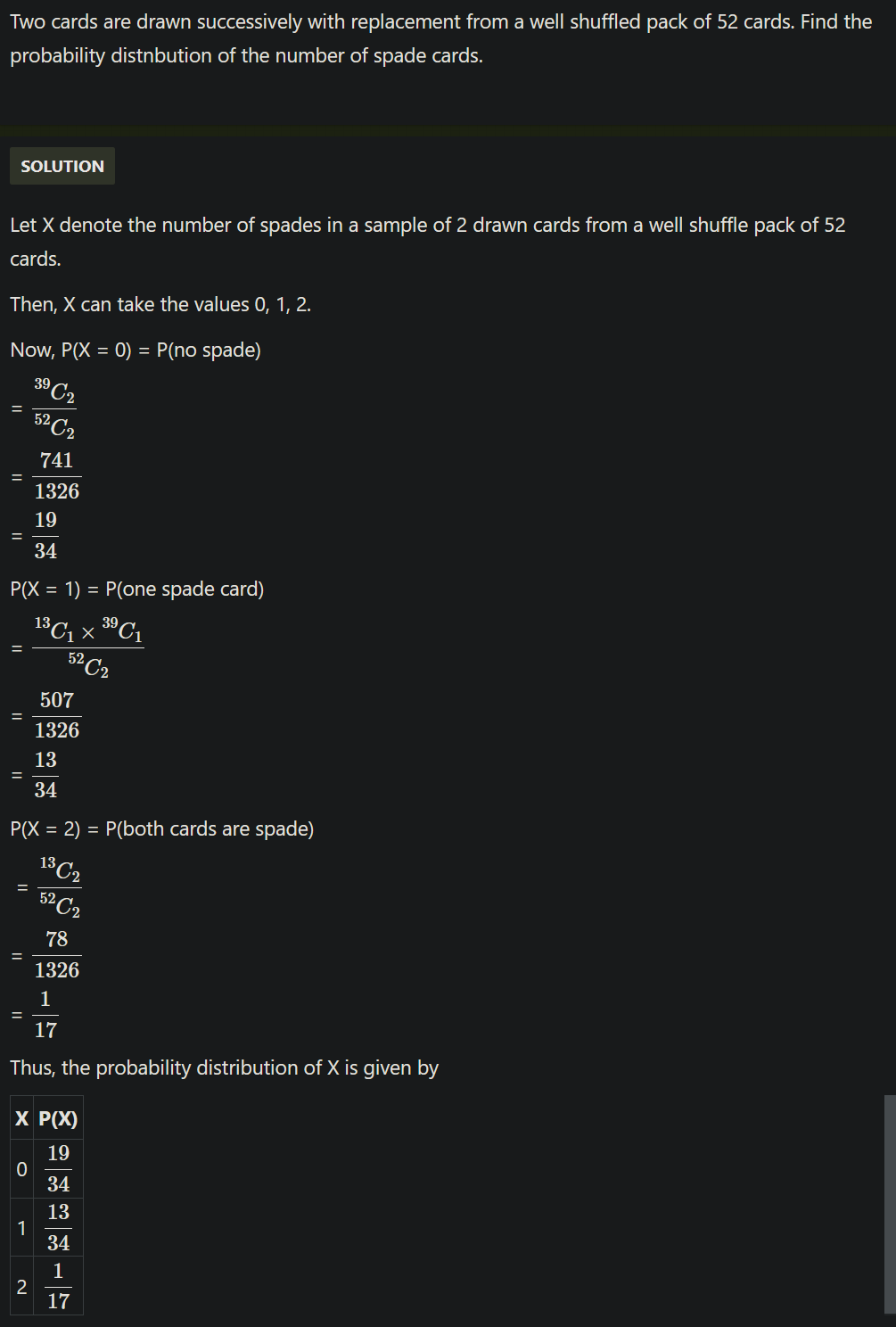
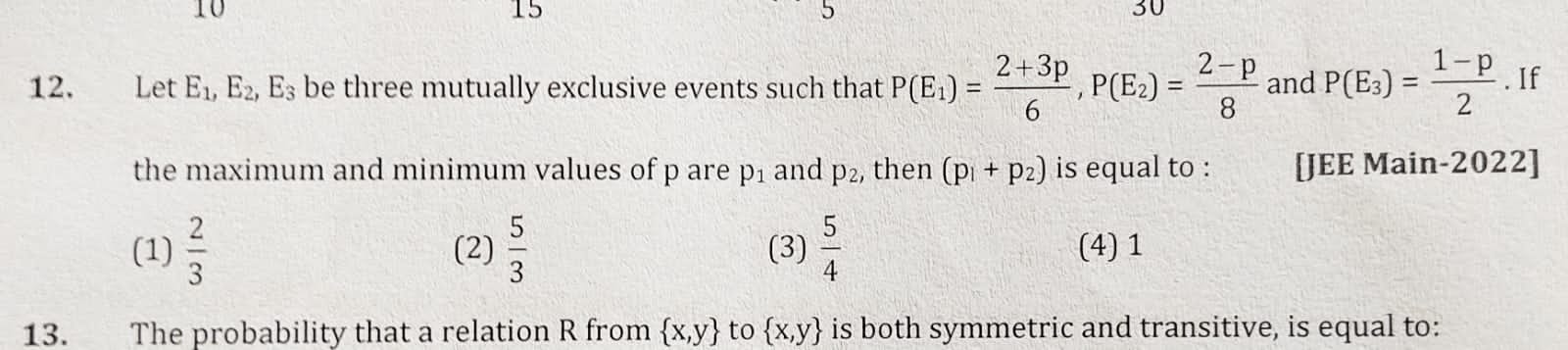CBSE Class 12-science Answered

consider a town with n people. A person spreads a rumuor to a second, who in turn repeats it to the third and so on. Suppose that at each stage the recipient of the rumour is chosen at random from the remaining (n-1) peoples. what is the probability that the rumour will be repeated n times (i) without being repeated to any person (ii) without being repeated to the originator?
Asked by deepak.embedded | 20 Oct, 2010, 12:00: AM
answered-by-expert Expert Answer
Dear Student
Here is the solution of your question
Since there are n people in the village so the rumour will be spread n times so the possible chances will be nn
(i) For the first case possible cases will be n.(n-1).(n-2)......1 since the rumor is not repeated to any person
So required probability = n.(n-1).(n-2)......1/nn
(ii) Here, apart from first rumor can be repeated to any person
so possible cases are n(n-1)(n-1)
So the probability = n(n-1)(n-1) /nn
Regards
Team Topperlearning
Answered by | 22 Oct, 2010, 01:13: PM

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
CBSE 12-science - Maths
The probability that a pen manufactured by a company will be defective is 0.1. If 12 such pens are selected find the probability that i) exactly 2 will be detective ii) at least 2.will be defective iii)none will he defective
Asked by annapoornaa429 | 31 Mar, 2024, 08:23: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
bag 1 contains 8 red and 2 black balls. bag 2 contains 7 red and 5 Black balls. one of the bags is chosen at random and a ball is drawn from it, which is found to be black. find the probability that the ball is drawn from bag 1.
Asked by janusingh736 | 13 Mar, 2024, 04:19: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Q27 Check In an experiment, 2 balls are drawn containing 4 red balls and 6 black balls. Let X be the number of red balls selected. Complete the table below: Outcome overline P(X) XP(X)
question image
Asked by khushisjkvm | 04 Jan, 2024, 07:14: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
the question and the solution is in the attachment, can you explain how can they use combinations when combinations does not account for replacement while it is mentioned in the question that replacement is to be taken into account? And can you provide the correct answer.
question image
Asked by ashwinskrishna2006 | 26 Dec, 2023, 07:34: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
doubt
question image
Asked by armaanjain840 | 20 Dec, 2023, 05:59: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Two urns contains 1 white,6 red and 4 white,3 red balls. One of t 3 he urn is selected at random and a ball is drawn from it. Find: (1) The probability of drawing a red ball. (ii) The probability of drawing the ball from the first urn if b all drawn is red.
Asked by babitdevi283 | 14 Nov, 2023, 09:42: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
4. The probability that a visitor in the dining room will need the first course is equal to 0.8.  Find the probability that out of 100 visitors, no less than 75 and no more than 90 visitors will order the first dish.
Asked by surajsharmaisonline | 20 Jan, 2023, 12:28: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Assuming the probability of a male birth as 1/2, find the probability that a family of 3 children will have: a) two boys and one girl b) at most two girls 
Asked by arjunsah797 | 03 May, 2022, 10:04: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
A Five digits number is written down at random. Find the probability that number is divisible by 5 and no two consecutive digits are identical.
Asked by rairp2017 | 27 May, 2021, 12:21: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
CBSE 12-science - Maths
Find the probability of success p for a binomial distribution, if n = 6 and 4 × P(X=4) = P(X=2)
Asked by 1999shaileshraj | 22 Feb, 2020, 11:50: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt
Get Latest Study Material for Academic year 24-25 Click here
×