CBSE Class 10 Answered

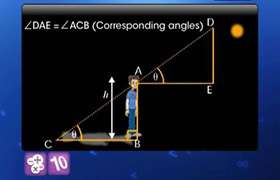
Consider a top view, as shown in figure,

If the height of tower is h, and horizontal distance from station A to the tower bottom is AC, the distance from station A to the top of the tower is AD. Since we are using top view points C and D will coincide.

Also the horizontal distance from station B to the bottom of tower is BC and to the top is BD.

Now sin a = h/AD; sin b = h/BD

Squaring,

sin² a = h²/AD²;       sin² b = h²/BD² ..................(1)

AD² = AC² + h²; and BD² = BC² + h²

 But BC² = AB² + AC²

BD² = AB² + AC² + h²

Put for AC² + h² = AD² = h²/sin²a from (1)

BD² = AB² + h²/sin²a

Therefore,

sin² b = h²/BD² , becomes,

sin² b = h²/ (AB² + h²/sin²a)

sin² b(AB² + h²/sin²a) = h²

AB² sin² b  + h²sin²b/sin²a = h²

AB² sin² b  =  h²(1 - sin²b/sin²a) 

AB² sin² b sin²a =  h²( sin²a - sin²b)

 h² = AB² sin² b sin²a/(sin²a - sin²b)

h = AB sin b sina/(sin²a - sin²b)

Regards,

Team,

TopperLearning.