Class 9 FRANK Solutions Maths Chapter 20: Constructions of Quadrilaterals
Revise some geometry Frank Solutions for ICSE Class 9 Mathematics Chapter 20 Constructions of Quadrilaterals. Be it a trapezium or a parallelogram, go through the step-wise instructions to construct a quadrilateral. Our chapter-wise textbook solutions include model answers by experts that build your skills for doing well in your Maths exam.
If constructing geometric figures is a challenge for you, our Frank textbook solutions can guide you with the steps to create the accurate constructions. TopperLearning further supports your Maths learning by equipping you with online study materials like online practice tests, Selina solutions, etc.
Constructions of Quadrilaterals Exercise Ex. 20.1
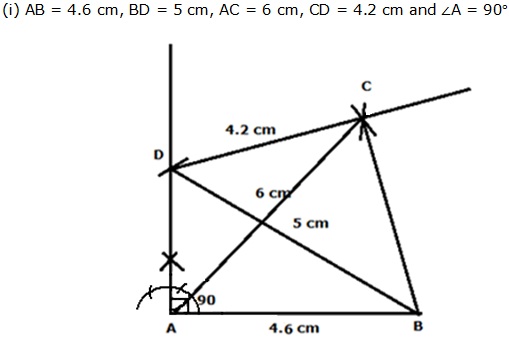
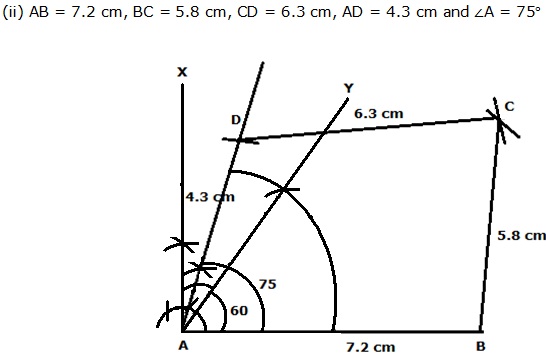
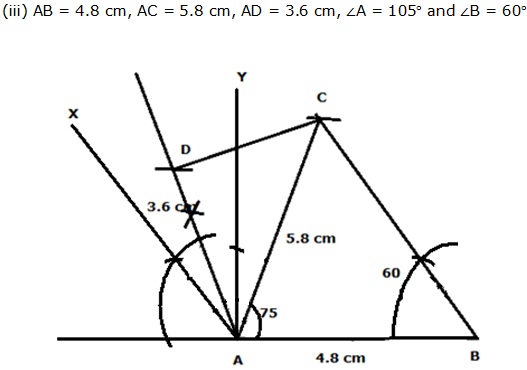
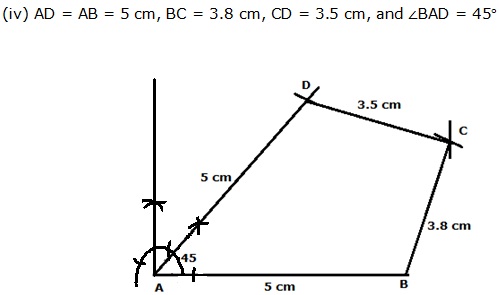
Solution 1




Solution 2
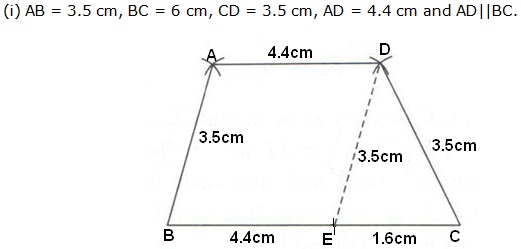

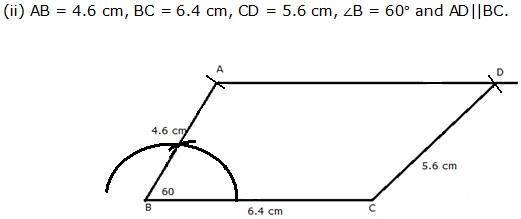

Solution 3
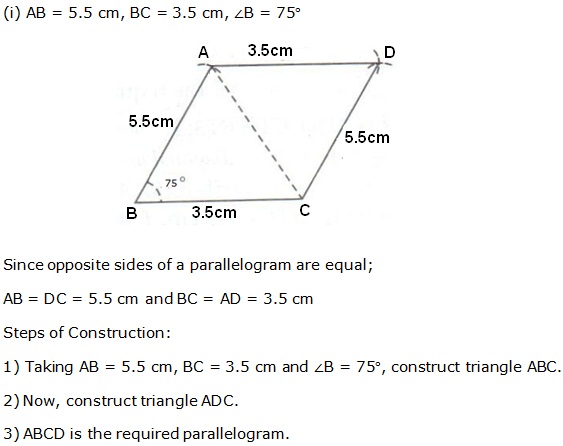
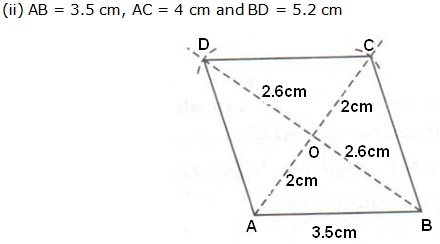

Solution 4
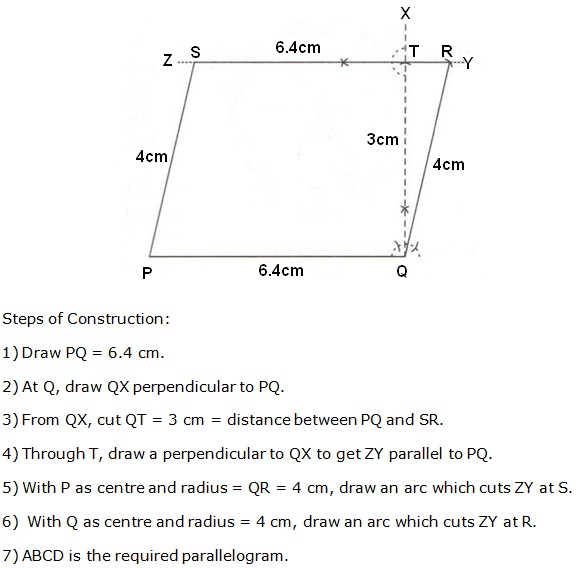
Solution 5
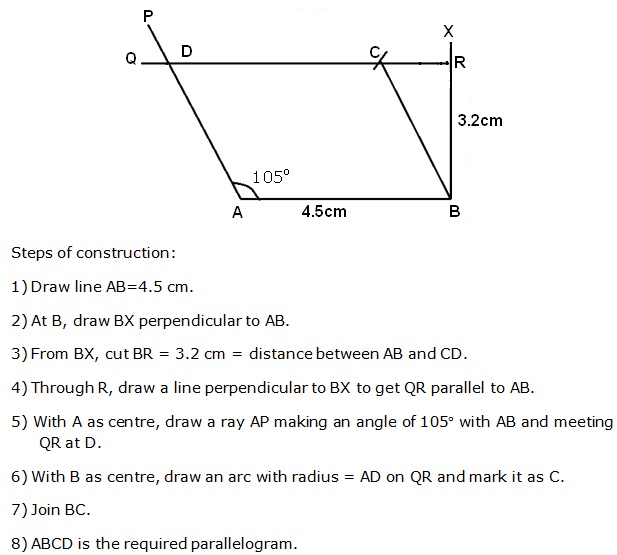
Solution 6
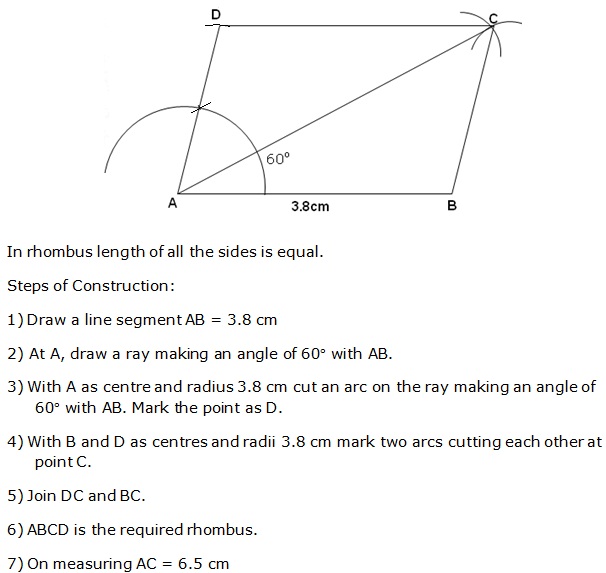
Solution 7
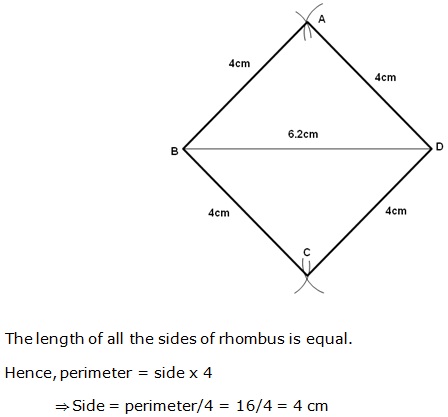

Solution 8
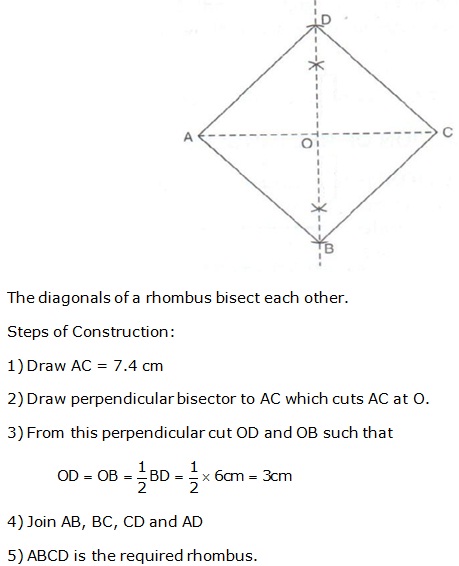
Solution 9
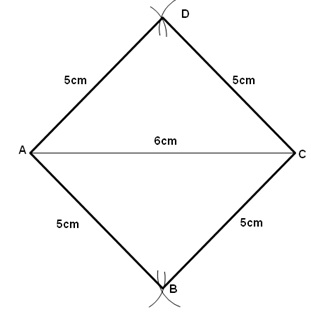

Solution 10

Solution 11
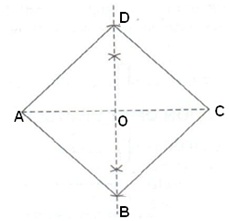
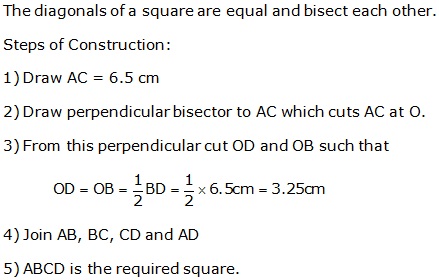
Solution 12
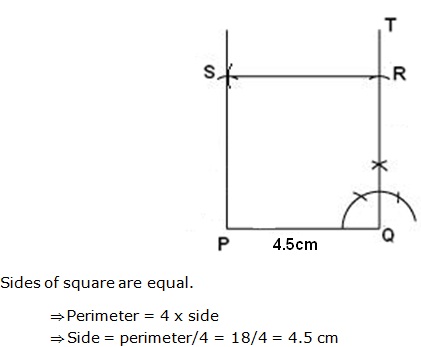

Solution 13
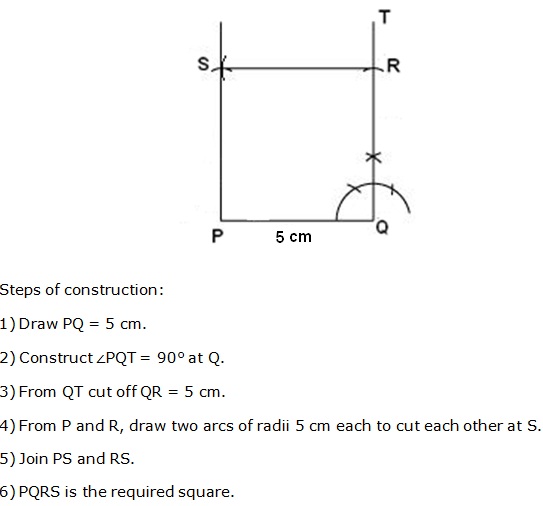
Solution 14(a)
Steps of construction:
- Draw AD = 3.2 cm
- Draw ∠XAD = 90°.
- With D as centre and radius BD = 5.5 cm, draw an arc to cut AX at point B.
- Join BD.
- With B as centre and radius 3.2 cm draw an arc and with D as centre and radius = AB, draw another arc to cut the previous arc at C.
- Join BC and CD.
Thus, ABCD is the required rectangle.
CD = 4.5 cm
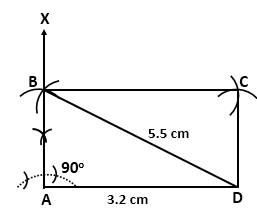
Solution 14(b)
Steps of construction:
- Draw BC = 6.2 cm
- Through B, draw BP such that ∠B = 90°
- From BP, cut BA = 5 cm
- With A and C as centres and radii 6.2 cm and 5 cm respectively, draw arcs cutting each other at D.
- Join AD and CD.
Thus, ABCD is the required triangle.
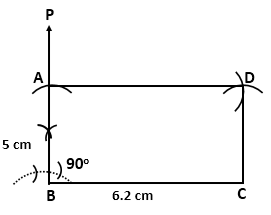
Solution 15


Solution 16
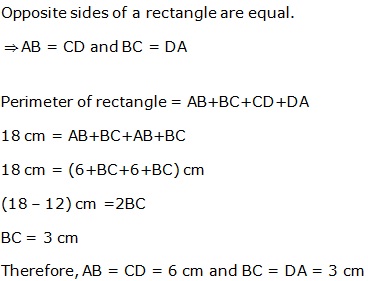
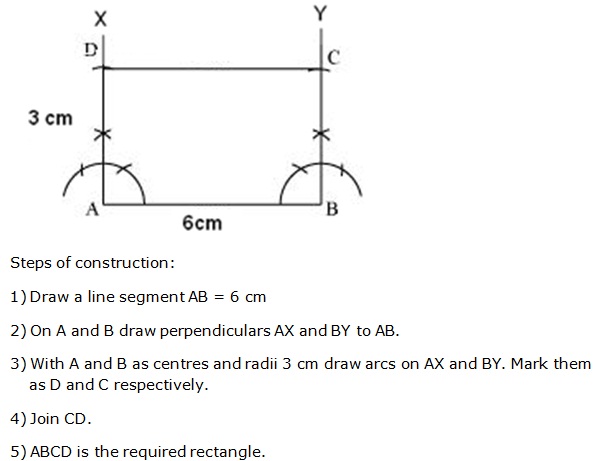
Solution 17
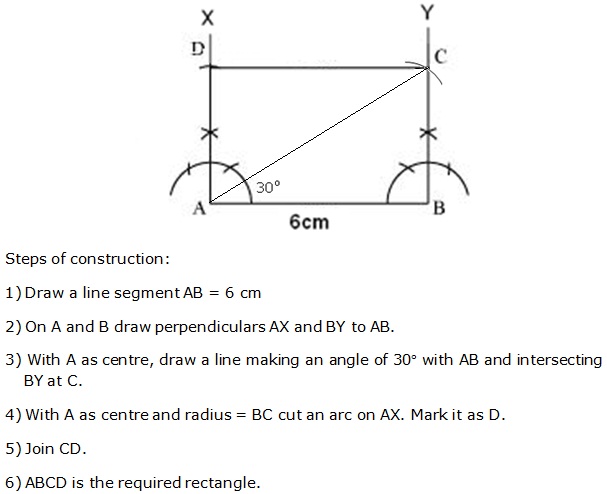
Solution 18(a)
Since area of rectangle = 21 cm2
And, length = 4.2 cm
Breadth = Area ÷ Length = 21 ÷ 4.2 = 5 cm
Steps of construction:
- Draw BC = 5 cm
- Through B, draw BP such that ∠B = 90°
- From BP, cut BA = 4.2 cm
- With A and C as centres and radii 5 cm and 4.2 cm respectively, draw arcs cutting each other at D.
- Join AD and CD.
Thus, ABCD is the required triangle.
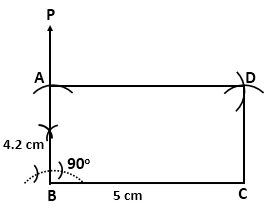
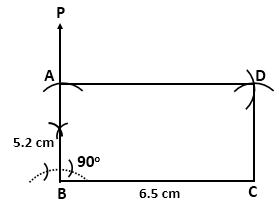
Solution 18(b)
Since area of rectangle = 33.8 cm2
And, breadth = 6.5 cm
Length = Area ÷ Breadth = 33.8 ÷ 6.5 = 5.2 cm
Steps of construction:
- Draw BC = 6.5 cm
- Through B, draw BP such that ∠B = 90°
- From BP, cut BA = 5.2 cm
- With A and C as centres and radii 6.5 cm and 5.2 cm respectively, draw arcs cutting each other at D.
- Join AD and CD.
Thus, ABCD is the required triangle.
Solution 19
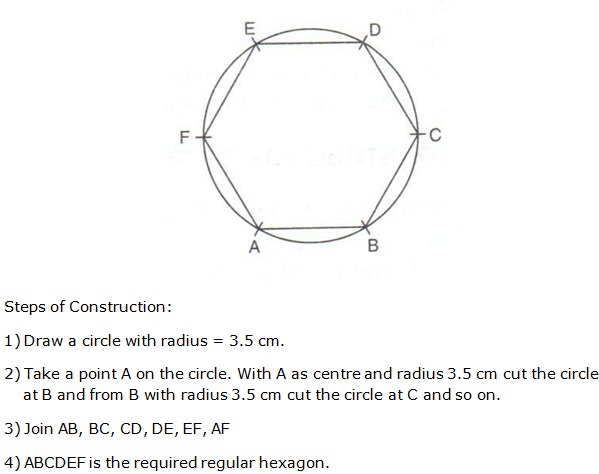
Solution 20

