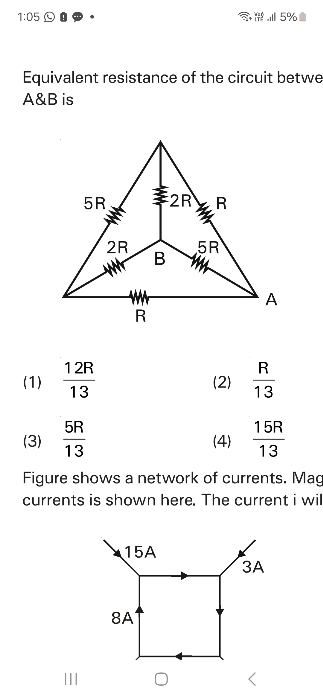
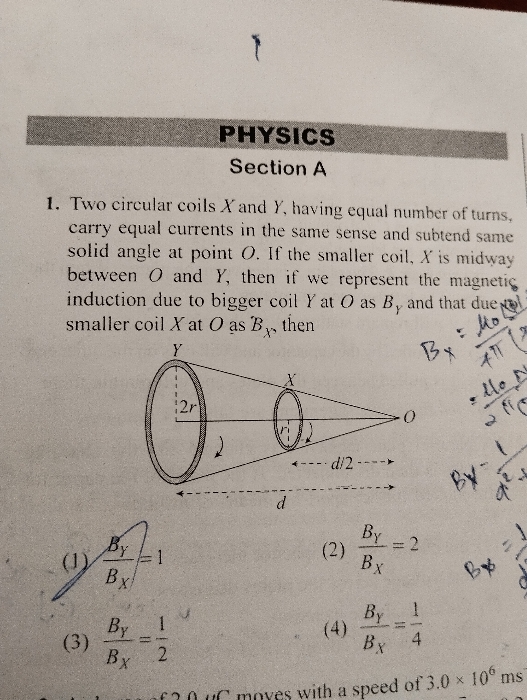
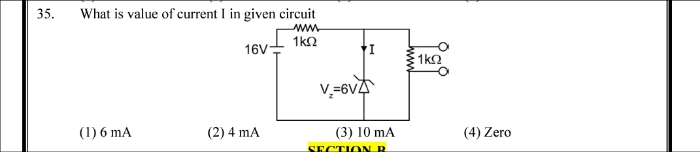
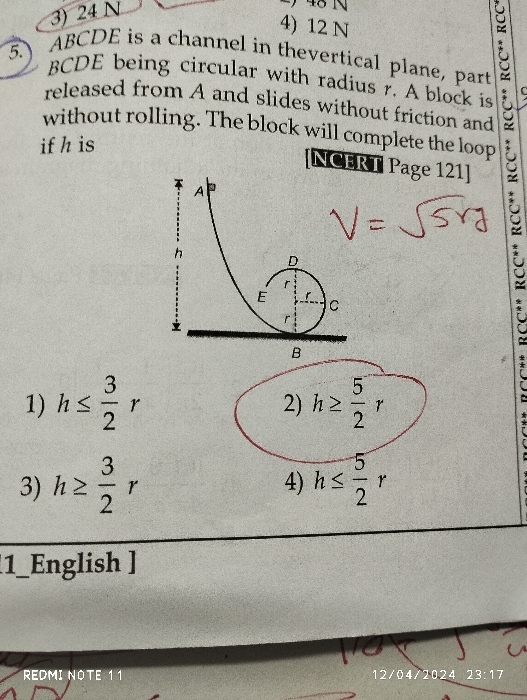NEET Class neet Answered
please answer this
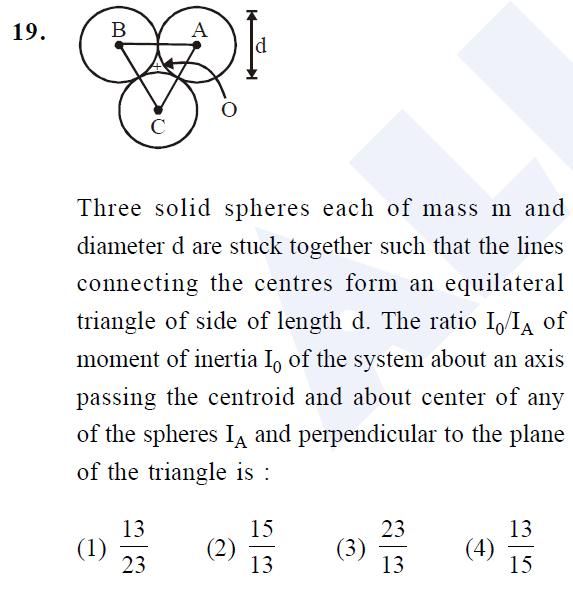
Asked by Prashant DIGHE | 26 Jan, 2020, 09:36: PM
Expert Answer
If point O is centroid of triangle ABC, the distance OA = (2/3) of height of triangle from base BC
Hence OA = (2/3) ×(√3/2) × (2R) = (2/3)(√3R)
where R is radius of sphere
By parallel-axis theorem, Moment of inertia I of each sphere about an axis passing through O is given by,
I= (2/5)MR2 + (4/3)MR2
where M is mass of sphere.
Hence Moment of inertia Io of all three sphers about an axis passing through O is given by,
Io = 3 × [ (2/5)MR2 + (4/3)MR2 ] = (26/5) MR2 ......................(1)
If Axis of rotation is passing through A, then moment of inertia IA of whole system is given by
IA = (2/5)MR2 + [ (2/5)MR2 + 4MR2 ] + [ (2/5)MR2 + 4MR2 ] = (46/5) MR2 .......................(2)
Using eqn.(1) and eqn.(2), ( Io / IA ) = 26/46 = 13/23
Answered by Thiyagarajan K | 27 Jan, 2020, 09:52: AM
Application Videos
-
Growth of Current in L-R Circuit
In this video, the growth of current in RL circuit is explained. It include...
NEET neet - Physics
Asked by ramanjaneyuluoguru | 25 Apr, 2024, 04:18: PM
ANSWERED BY EXPERTNEET neet - Physics
Asked by shatakshibhatt9 | 20 Apr, 2024, 07:52: PM
ANSWERED BY EXPERT
NEET neet - Physics
Asked by praveenpriya000079 | 18 Apr, 2024, 07:24: AM
ANSWERED BY EXPERTNEET neet - Physics
Asked by gouranshi84 | 17 Apr, 2024, 05:23: PM
ANSWERED BY EXPERTNEET neet - Physics
Asked by sojusvi | 17 Apr, 2024, 01:12: PM
ANSWERED BY EXPERT
