what do you mean by equivalence classes? explain with examples.

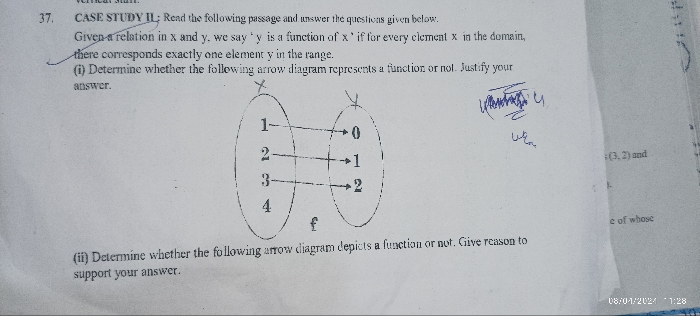
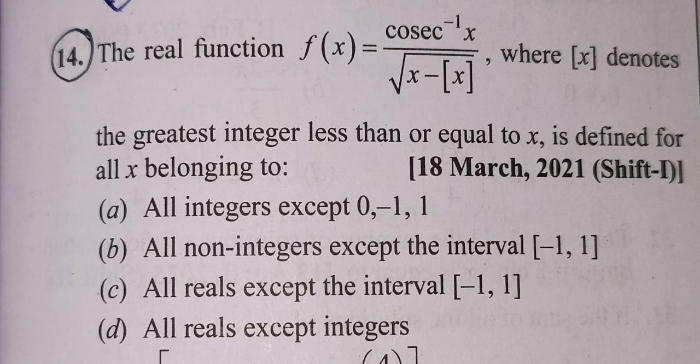
CBSE Class 12-science Answered

what do you mean by equivalence classes? explain with examples.

Asked by anchaka | 02 Apr, 2013, 08:59: PM

Expert Answer

In mathematics, given a set

X

and an equivalence relation

~

X

, the equivalence class of an element

n

X

is the subset of all elements in

X

which are equivalent to

n

. Equivalence classes among elements of a structure are often used to produce a smaller structure whose elements are the classes, distilling a relationship every element of the class shares with at least one other element of another class.

Examples

If $X$ is the set of all cars, and $~$ is the equivalence relation "has the same color as", then one particular equivalence class consists of all green cars. $X /~$ could be naturally identified with the set of all car colors (cardinality of $X /~$ would be the number of all car colors)
Consider the modulo 2 equivalence relation on the set $Z$ of integers: $x ~ y$ if and only if their difference $x ? y$ is an even number. This relation gives rise to exactly two equivalence classes: one class consisting of all even numbers, and the other consisting of all odd numbers. Under this relation $[7]$ , $[9]$ , and $[1]$ all represent the same element of $Z /~$ .
Let $X$ be the set of ordered pairs of integers $(a, b)$ with $b$ not zero, and define an equivalence relation $~$ on $X$ according to which $(a, b) ~ (c, d)$ if and only if $ad = bc$ . Then the equivalence class of the pair $(a, b)$ can be identified with the rational number $a / b$ , and this equivalence relation and its equivalence classes can be used to give a formal definition of the set of rational numbers. The same construction can be generalized to the field of fractions of any integral domain.

Answered by | 03 Apr, 2013, 01:55: AM