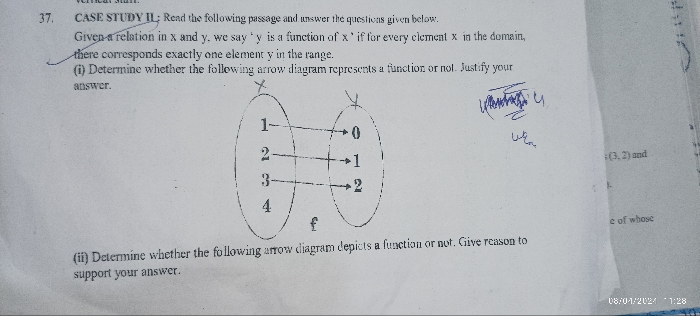
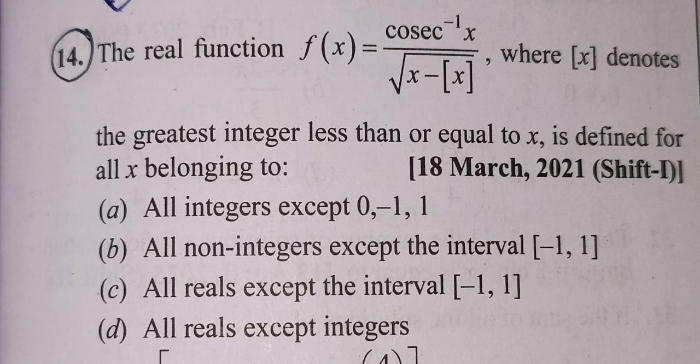
CBSE Class 12-science Answered

A relation R in a set A is said to be an equivalence relation if R is reflexive, symmetric and transitive

A relation R in a set A is called

1. Reflexive, if (a, a) Î R, for every a Î A,
2. Symmetric, if (a₁, a₂) Î R implies that (a₂, a₁) Î R, for all
   a₁, a₂ Î A.
3. Transitive, if (a₁, a₂) Î R and (a₂, a₃) Î R implies that
   (a₁, a₃) Î R, or all a₁, a₂, a₃ Î A.

Example:

1) Relation R in the set A of human beings in a town at a particular time given by R = {(x, y): x is father of y}

(x, x) ? R

As x cannot be the father of himself.

?R is not reflexive.

Now, let (x, y) ?R.

? x is the father of y.

? y cannot be the father of y.

Indeed, y is the son or the daughter of y.

?(y, x) ? R

? R is not symmetric.

Now, let (x, y) ? R and (y, z) ? R.

? x is the father of y and y is the father of z.

? x is not the father of z.

Indeed x is the grandfather of z.

? (x, z) ? R

?R is not transitive.

Hence, R is neither reflexive, nor symmetric, nor transitive. Hence, it is not an equivalence relation.

2) Consider the relation R in the set A of all the books in a library of a college, given by R = {(x, y): x and y have same number of pages}

Set A is the set of all books in the library of a college.

R = {x, y): x and y have the same number of pages}

Now, R is reflexive since (x, x) ? R as x and x has the same number of pages.

Let (x, y) ? R ? x and y have the same number of pages.

? y and x have the same number of pages.

? (y, x) ? R

?R is symmetric.

Now, let (x, y) ?R and (y, z) ? R.

? x and y and have the same number of pages and y and z have the same number of pages.

? x and z have the same number of pages.

? (x, z) ? R

?R is transitive.

Hence, R is an equivalence relation.