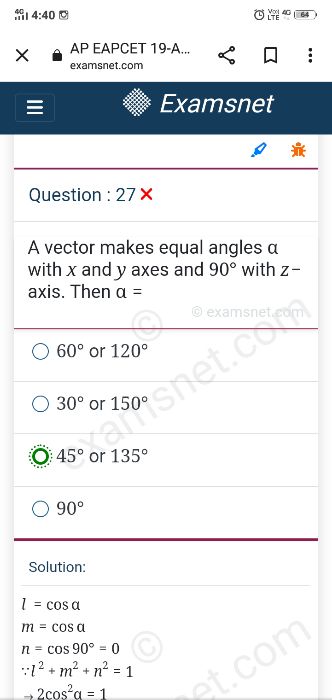
CBSE Class 12-science Answered
If |a+b|=|a-b|,show that a and b are perpendicular vectors
Asked by Snehil Sankalp | 20 Apr, 2012, 12:16: PM
Expert Answer
Given: |a+b|=|a-b|
To Prove: a and b are perpendicular vectors
So, if you square both the sides, then you will get
(|a+b|)^2 = (|a-b|)^2
Since, any |a|^2 can be written as the dot product of a with itself i.e. a.a , so here also (|a+b|)^2 can be represented as (a+b).(a+b). Using the same logic
(a+b).(a+b) = (a-b).(a-b)
On expanding, we will get
a.a +b.b +2a.b = a.a +b.b -2a.b
4a.b=0
4|a|*|b|*cos(theta) = 0
Since, a and b vectors are non-zero vectors, hence
cos(theta) = 0
and hence, theta = 90
So, the angle between a and b vectors is 90.
Thank you
Answered by | 21 Apr, 2012, 10:47: AM
Concept Videos
-
Vector multiplication concepts
Multiplication of a vector by a scalar, vector joining two points. Directio... -
Cross product
This video explains the properties of vector product especially cross produ... -
Introduction to vector algebra
This video explains the definition of vector and its types, direction angle... -
Concepts and application on properties of vector ...
Multiplication of a vector by a scalar, vector joining two points. Directio... -
Concepts on Vector cross product
Vector or cross product. Properties of vector product.
CBSE 12-science - Maths
Asked by sdmbotch1123 | 18 Jan, 2023, 11:01: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
If |𝑎⃗| = 3 and −1 ≤ 𝑘 ≤ 2, then |𝑘𝑎⃗| lies in the interval
(a) [0,6] (b) [−3,6] (c) [3,6] (d) [1,2]
Asked by mohdtayyab682 | 18 Jan, 2023, 10:34: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by durgaprasaddurgaprasad174 | 01 Jul, 2022, 05:05: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sujaychandramouli | 24 May, 2022, 10:46: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by abhimeena1011 | 18 Jan, 2022, 10:43: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by gurupadam3101985 | 11 Apr, 2020, 10:06: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 07 Feb, 2020, 09:49: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by lovemaan5500 | 16 Dec, 2019, 09:34: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by Vivek.d8765 | 29 Oct, 2019, 10:14: PM
ANSWERED BY EXPERT
