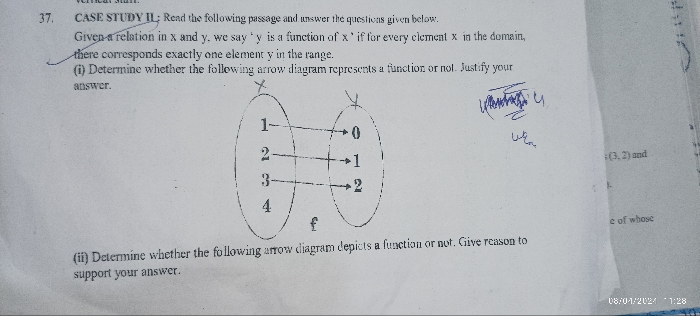
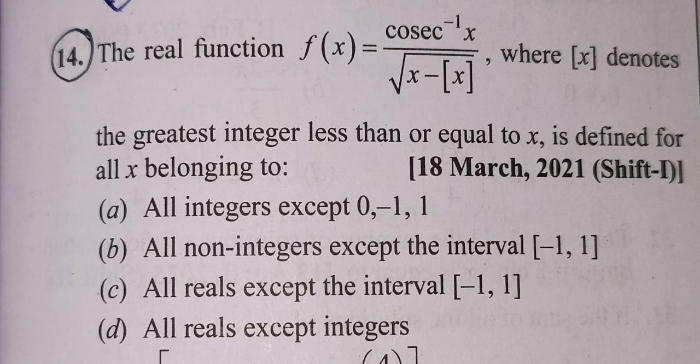
CBSE Class 12-science Answered
how can we prove that a function is onto? please explain that if range = codomain then the function is said to be onto. how?
Asked by sreenanda dhar | 01 Jan, 2014, 12:24: PM
Expert Answer
Consider a function 
The set of all elements of X are called the domain of f and the set of all elements of Y are called the range of f.
Range is also called as image of f.
In general, the image of f is a subset of codomain.
It may coincide with its codomain.
A function f from X to Y is called onto if for all y in Y, there is an x in X, such that 
So to prove that a function is onto, we need to prove that the range of the function is equal to its codomain.
Now let us consider the function, 
This function is a straight line.
Suppose 
If x=1, then we have, f(x) = x + 1 = 2
If x=2, then we have, f(x) = x + 1 = 3
.
.
.
.
Thus, each x value has one unique y=f(x) value.
That is for all elements in the range is having their pre-images in domain.
Thus we have, range = codomain
Answered by Vimala Ramamurthy | 02 Jan, 2014, 09:54: AM
Application Videos
-
ProblemsbasedonIntoFunction-I
Video contains the question based on verification of a function to be Into ... -
ProblemsbasedonIntoFunction-II
Video contains the question based on verification of a function to be Into ...
Concept Videos
-
Introduction to Relations
This video explains the concept of relations and types of relation like Emp... -
Functions and its type
Learn the definition and way of exhibiting functions. Domain, co-domain and... -
Problems on Functions
This video revists the types of functions and solve problems based on them. -
Concepts related to composition of functions
Define composition of functions and establish the condition for a function... -
Problems on Composition
This video covers the concept of composition of functions and invertible fu...
CBSE 12-science - Maths
Asked by 20muskaansharma23 | 08 Apr, 2024, 11:31: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by nainaadityathakur | 12 Mar, 2024, 01:22: PM
ANSWERED BY EXPERT
CBSE 12-science - Maths
Asked by aiswaryaanil0009 | 01 Feb, 2024, 08:36: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by raunakumar9300 | 01 Feb, 2024, 04:23: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by amandwivesh | 25 Oct, 2023, 11:57: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by bornalimalakar3 | 05 Oct, 2023, 11:15: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by rginish12 | 04 Oct, 2023, 11:14: AM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by sdmbotch1123 | 19 Sep, 2023, 01:53: PM
ANSWERED BY EXPERTCBSE 12-science - Maths
Asked by iamujjy | 21 May, 2023, 04:55: PM
ANSWERED BY EXPERT
