Class 9 SELINA Solutions Physics Chapter 3: Laws of Motion
Laws of Motion Exercise Ex 3(A)
Solution A.1
(d) All of the above
A body's dimensions, state of rest or motion, size, shape, and dimensions can all change as a result of forces.
Solution A.2
Frictional force is a contact force.
Solution A.3
Force due to gravity is a non-contact force.
Solution A.4
(b) non-contact force
A ball placed on a table starts rolling down when the table is tilted because of gravitational force. Gravitational force is classified as a non-contact force.
Solution A.5
(d) electrostatic force
A phenomenon known as electrostatic force causes combs to charge up when they are rubbed against dry hair.
An example of a non-contact force is electrostatic force, which is produced when charged particles either attract or repel one another.
Solution A.6
(b) attractive
Gravitational force is always attractive, which pulls objects with mass towards each other.
Solution A.7
(b) decreases, increases
The magnitude of a non-contact force between two bodies is inversely proportional to the distance of separation between them.
i.e., the force decreases as the distance of separation increases and increases as the separation decreases.
Solution A.8
(c) one-fourth
On doubling the separation between two bodies, the non-contact force's magnitude becomes one-fourth (1/4) of its original value. (∵ F ∝ 1/R2)
Solution A.9
(c) restoring elastic force
A spring balance measures forces like weight or tension by restoring elastic force, which is the force a deformed spring uses to return to its original shape.
Solution A.10
(a) pushes, pulls

Solution B.1
The forces which act on bodies when they are in physical contact are called contact forces.
Solution B.2
The forces experienced by bodies even without being physically touched are called non-contact forces.
Solution B.3
Contact force: (a) frictional force (b) normal reaction force (c) force of tension in a string
Non-contact force: (d) gravitational force (e) electric force (f) magnetic force
Solution B.4
a. Force exerted on two bodies during collision.
b. Magnetic force between magnetic poles.
Solution B.5
The magnitude of non-contact force on two bodies depends on the distance of separation between them.
The force decreases as the distance of separation increases.
The force is inversely proportional to the square of the distance of separation.
Solution B.6
The magnitude of gravitational force between two masses will become four times as gravitational force varies inversely as the square of distance of separation.
Solution B.7
i. A fielder on the ground stops a moving ball by applying a force with his hands.
ii. The pull exerted by horse makes a cart moves.
iii. In a cycle pump, when the piston is lowered, the air is compressed to occupy a less volume.
iv. On pressing a piece of rubber, its shape changes.
Solution C.1
a. The forces which act on bodies when they are in physical contact are called contact forces.
Example: frictional force and force exerted on two bodies during collision.
b. The forces experienced by bodies even without being physically touched are called non-contact forces.
Example: Gravitational force and Electrostatic force.
Solution C.2
A force when applied on a non-rigid body changes the inter-spacing between its constituent particles and therefore causes a change in its dimensions and can also produce motion in it.
On the other hand, a force when applied on a rigid body, does not change the inter-spacing between its constituent particles and therefore it does not change the dimensions of the body but causes motion in it
Solution C.3
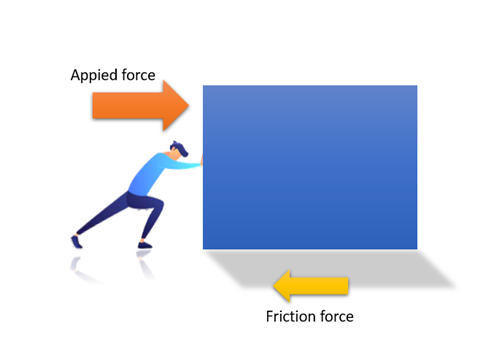
Friction or frictional force is the force that always opposes an object's motion over another object in contact with it.
For instance, when we push a heavy block on the ground, a force called frictional force will exist between the surfaces of the two objects.
Solution C.4
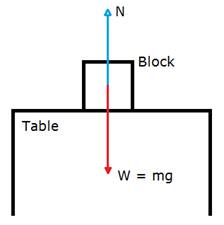
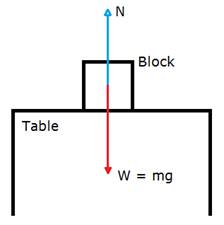
When a body is placed on a surface, it pushes down on the surface with force equal to its weight. The surface, known as the normal reaction force, applies an equal and opposite force to the body, preventing it from moving (or falling).
For example, The forces acting on the block are its weight in the downward direction and the normal reaction force due to the table in the upward direction.
Solution D.1
A.
B.
C.
The forces acting on the block are its weight in the downward direction and the normal reaction force due to the table on the upward direction.
Laws of Motion Exercise Ex 3(B)
Solution A.1
(c) both (a) and (b)
According to Newton's first law of motion, if a body is in a state of rest, it will remain in the state of rest, and if the body is in a state of motion, it will keep moving in the same direction with the same speed unless an external force is applied on it.
It includes the definition of inertia, which is the property of an object to resist changes in its motion, and the definition of force, which is the cause of a change in motion or acceleration of an object.
Solution A.2
(b) vector
Since it has both a magnitude and a direction, force is a vector quantity.
Solution A.3
(b) inertia
The property of an object by virtue of which it tends to retain its state of rest or motion is called inertia.
Inertia is one of the fundamental concepts in Newton's laws of motion, and it describes an object's resistance to changes in its motion.
Solution A.4
A truck
Solution A.5
Change the state of motion or state of rest of the body.
Solution A.6
Less force is required for the tennis ball than for the cricket ball.
Solution A.7
a. table tennis ball, tennis ball, football, cricket ball.
Solution A.8
(d) When a passenger jumps out of a moving train, he falls down.
When a passenger jumps out of a moving train, he falls down, as this is an example of inertia of motion, whereas rest are the examples of inertia at rest.
Solution A.9
(d) both (a) and (c)
Since the athlete's body tends to stay in motion until acted upon by an external force, the athlete's running before a long jump demonstrates Newton's first law and inertia of motion.
Solution A.10
(b) same, same
The inertia of motion is the tendency of an object to continue moving at a constant speed in a straight line unless acted upon by an external force, so the correct answer is (b) same, same.
Solution B.1
Force causes motion in a body.
Solution B.2
Force is not needed to keep a moving body in motion.
Solution B.3
The force of friction between the table and the ball opposes the motion of the ball.
Solution B.4
Galileo's law of inertia states that a body continues to be in its state of rest or of uniform motion unless an external force is applied on it.
Solution B.5
According to Newton's first law of motion, if a body is in a state of rest, it will remain in the state of rest, and if the body is in the state of motion, it will remain moving in the same direction with the same speed unless an external force is applied on it.
Solution B.6
Force is that external cause which can move a stationary object or which can stop a moving object.
Solution B.7
Inertia of a body depends on its mass. Inertia is directly proportional to mass, i.e. greater the mass of a body, greater is its inertia.
Solution B.8
Two kinds of inertia are as listed below:
(i) Inertia of rest.
(ii) Inertia of motion.
Solution B.9
Examples of inertia of rest: A coin placed on top of a card remains in place when the card is slightly and quickly jerked horizontally.
Example of inertia of motion: A ball thrown vertically upwards by a person in a moving train comes back to his hand.
Solution B.10
The net force on the airplane is zero or the upward force is equal to the downward force.
Solution C.1
In absence of any external force, its speed shall remain unchanged.
Solution C.2
The property of an object by virtue of which it neither changes its state nor tends to change the state is called inertia.
Solution C.3
Examples to show that greater the mass, greater is the inertia of the body are as shown below:
(i) If you want to start a car by pushing it, you find that it takes a very large force to overcome its inertia. On the other hand, only a small force is needed to start a child's express wagon. The difference between the car and express wagon is the difference in mass. The car has a large mass, whereas the wagon has a small one.
(ii) A cricket ball is more massive than a tennis ball. The cricket ball acquires a much smaller velocity than a tennis ball when the two balls are pushed with equal force for the same time.
Solution C.4
It is difficult, i.e. a larger force is required to set a loaded trolley (which has more mass) in motion than an unloaded trolley (which has less mass).
Solution C.5
No, the body will not move because the net force acting on it is zero. Hence, it will remain stationary due to inertia of rest.
Solution C.6
The motion remains unaffected because the net force acting on it is zero.
Solution C.7
If a person jumps out of a moving train and tries to stop immediately, he falls due to inertia of motion. This is because his body tends to move forward with the velocity of the train while his feet are stationary.
Solution C.8
The reason is that when the card is flicked, a momentary force acts on the card, so it moves away. However, the coin kept on it does not share the motion at once and it remains stationary at its place due to the inertia of rest. The coin then falls down into the tumbler due to the pull of gravity.
Solution C.9
The reason is that when the ball is thrown, the ball is in motion along with the person and train. Due to the inertia of motion, during the time the ball remains in air, the person and ball move ahead by the same distance. This makes the ball fall back into the thrower's hand.
Solution C.10
When the branches of a tree are shaken, an external force is applied to the fruits attached to the branches. This force overcomes the inertia of the rest of the fruits, which tends to keep them in their initial position. As a result, the fruits fall from the tree, making it easier for people to harvest them.
This phenomenon demonstrates the principle of inertia, where an object tends to remain at rest unless acted upon by an external force.
Solution D.1
Statement of Newton's first law: If a body is in a state of rest, it will remain in the state of rest, and if the body is in the state of motion, it will remain moving in the same direction with the same speed unless an external force is applied on it.
Explanation: Newton's first law can be explained in the following two parts:
(i) Definition of inertia: The 1st part of Newton's first law of motion gives the definition of inertia, according to which an object cannot change its state by itself.
Example: A book lying on a table will remain in its position unless it is displaced.
(ii) Definition of force: The 2nd part of Newton's first law defines force, according to which force is that external cause which can move a stationary object or which can stop a moving object.
Example: A book lying on a table is displaced from its place when it is pushed.
Solution D.2
(a) When a train suddenly starts, the passengers tend to fall backwards. This is because the lower part of the body, which is in contact with the train, begins to move while the upper part of the body tends to maintain its position of rest. As a result, the upper part tends to fall backwards.
(b) The frame of the sliding door being in contact with the floor of the train also comes in motion on start of the train, but the sliding door remains in its position due to inertia. Thus, the frame moves ahead with the train, while the door slides opposite to the direction of motion of the train. Thus, the door may shut or open accordingly.
(c) When people alight from a moving bus, they continue to run alongside the bus to avoid falling. If they were to stop at once, the feet would come to rest suddenly but the upper part of the body would still be in motion and they would tend to fall forward.
(d) The part of the carpet where the stick strikes comes in motion at once, while the dust particles settled on it remain in the state of rest due to inertia of rest. Thus, a part of the carpet moves ahead with the stick leaving behind the dust particles that fall down due to gravity.
(e) When running, the athlete brings his body in the state of motion. When the body is in motion, it becomes easier to take a long jump.
Laws of Motion Exercise Ex 3(C)
Solution A.1
(c) both (a) and (b)
The force needed to stop a moving body in a definite time depends on both the body's mass (option a) and the body's velocity (option b). Therefore, the correct answer is (c) both (a) and (b).
Solution A.2
(c) mv
Solution A.3
(a) N s
Solution A.4
(b) vector quantity
Linear momentum is a vector quantity (b), which has both magnitude and direction.
It is defined as the product of an object's mass and velocity, and its direction is the same as the direction of its velocity.
Solution A.5
(b) qualitatively
Newton's first law of motion gives the qualitative definition of force.
It explains the force as the cause of acceleration only qualitatively, but Newton's second law of motion gives the quantitative value of force. It states force is the product of mass and acceleration. Thus, it relates force to measurable quantities such as acceleration and mass.
Solution A.6
(b) directly proportional to the force applied on it
The mathematical expression of Newton's second law of motion is as shown below:
Force = Mass × Acceleration
i.e., Force ∝ Acceleration.
Solution A.7
(a)
![]()
Solution A.8
(b) F = ma
The mathematical expression of Newton's second law of motion is as shown below:
Force = Mass × Acceleration
Solution A.9
(d):
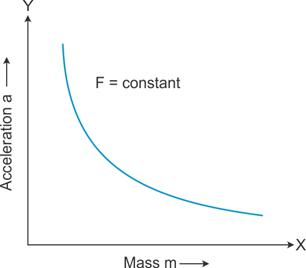
When a force is applied to bodies with varying masses, the acceleration that results is inversely proportional to the masses of the bodies.
i.e., F = ma⇒ m ∝ 1/a
A graph plotted for acceleration (a) against mass (m) is a hyperbola.
Solution A.10
(c) Mass of the body
Solution A.11
(c) Newton
The S.I. unit of force is the newton.
Solution A.12
(d) 105 dyne
1 N = 105 dyne.
Solution A.13
(c) 10 N
Given that,
Mass, m = 2 kg
Acceleration, a =5 m/s2
Now,
Force = m× a = 2× 5 =10 N
Solution A.14
(c) similar to the direction of force applied
Newton's second law of motion states that the rate of momentum change is directly proportional to the force acting on it and that momentum change occurs in the direction of the force.
Solution A.15
(d) both (b) and (c)
Velocities should be much smaller than the speed of light, and mass should remain constant for the equation to be applicable.
Solution A.16
(a) 1:1
For the ball with a mass of 4m:
Momentum (p1) = Mass (m1) × Velocity (v1) = 4m × 2v = 8mv
For the ball with a mass of 8m:
Momentum (p2) = Mass (m2) × Velocity (v2) = 8m × v = 8mv
Now we can find the ratio of their momenta:
p1: p2 = 8mv: 8mv = 1:1
Solution B.1
Linear momentum of a body is the product of its mass and velocity.
Its SI unit is kgms-1.
Solution B.2
(i) When v![]()
![]() c,
c,
![]() p =
p = ![]() (mv) = m
(mv) = m![]() v
v
(ii) When v ![]() c,
c,
![]() p =
p = ![]() (mv)
(mv)
(iii) When v![]()
![]() c but m does not remain constant.
c but m does not remain constant.
![]() p =
p = ![]() (mv)
(mv)
Solution B.3
(i) Mass is the measure of inertia.
Let 'm' be the mass of the two bodies.
Inertia of body A:Inertia of body B :: m:m
Or, Inertia of body A:Inertia of body B :: 1:1
(ii) Momentum of body A = m (v)
Momentum of body B = m (2v) = 2mv
Momentum of body A:Momentum of body B :: mv:2mv
Or, Momentum of body A:Momentum of body B :: 1:2.
Solution B.4
(i) Inertia of body A:Inertia of body B :: m:2m
Or, Inertia of body A:Inertia of body B :: 1:2.
(ii) Momentum of body A = m ( 2v) = 2mv
Momentum of body B = (2m) v = 2mv
Momentum of body A:Momentum of body B :: 2 mv:2mv
Or, Momentum of body A:Momentum of body B :: 1:1.
(iii) According to Newton's 2nd law of motion, rate of change of momentum is directly proportional to the force applied on it. Therefore,
Force needed to stop A:Force needed to stop B :: 1:1.
Solution B.5
The S.I. unit of force is newton.
One newton is the force which acts on a body of mass 1kg and produces an acceleration of 1 m/s2, i.e. 1 N = 1 kg × 1 m/s2.
Solution B.6
The C.G.S. unit of force is dyne.
One dyne is the force which acts on a body of mass 1 gramme and produces an acceleration of 1 cms-2, i.e. 1 dyne = 1 g × 1 cms-2.
Solution B.7
The S.I. unit of force is newton and the C.G.S. unit of force is dyne.
1 N = 105 dyne.
Solution C.1
Force needed to stop a moving body in a given time depends on its mass and velocity.
Solution C.2
Let a force 'F' be applied on a body of mass m for a time 't' due to which its velocity changes from u to v. Then,
Initial momentum of body = mu
Final momentum of body = mv
Change in momentum of the body in 't' seconds = mv ![]() mu = m (v
mu = m (v![]() u)
u)
Rate of change of momentum = Change in momentum/time
= [m (v![]() u)]/t
u)]/t
However, acceleration a = Change in velocity/time = (v![]() u)/t
u)/t
Therefore, rate of change of momentum = ma = mass × acceleration
This relation holds true when the mass of the body remains constant.
Solution C.3
According to Newton's second law of motion, the rate of change of momentum is directly proportional to the force applied on it and the change of momentum takes place in the direction in which the force is applied.
It gives the quantitative value of force, i.e. it relates the force to the measurable quantities such as acceleration and mass.
Solution C.4
Newton's first law of motion gives the qualitative definition of force. It explains the force as the cause of acceleration only qualitatively but Newton's second law of motion gives the quantitative value of force. It states force as the product of mass and acceleration. Thus, it relates force to the measurable quantities such as acceleration and mass.
Solution C.5
Mathematical expression of Newton's second law of motion is as shown below:
Force = Mass × Acceleration
Above relation holds for the following conditions:
(i) When the velocity of the body is much smaller than the velocity of light.
(ii) When the mass remains constant.
Solution C.6
According to Newton's second law of motion, the rate of change of momentum is directly proportional to the force applied on it, and the change of momentum takes place in the direction in which the force is applied.
The relation F=ma holds for the following conditions:
(i) When the velocity of the body is much smaller than the velocity of light.
(ii) When the mass remains constant.
Solution C.7
From Newton's second law of motion, F = ma.
If F = 0, then a = 0.
This means that if no force is applied on the body, its acceleration will be zero. If the body is at rest, then it will remain in the state of rest and if it is moving, then it will remain moving in the same direction with the same speed. Thus, a body not acted upon by an external force, does not change its state of rest or motion. This is the statement of Newton's first law of motion.
Solution C.8
When a glass vessel falls from a height on a hard floor, it comes to rest almost instantaneously, i.e. in a very short time, so the floor exerts a large force on the vessel and it breaks. However, if it falls on a carpet, then the time duration, in which the vessel comes to rest, increases, so the carpet exerts less force on the vessel and it does not break.
Solution C.9
(a) We pull our hands back while catching a fast moving cricket ball, because by doing so, we increase the time of catch, i.e. increase the time to bring about a given change in momentum, and hence, the rate of change of momentum decreases. Thus, a small force is exerted on our hands by the ball.
(b) When an athlete lands from a height on a hard floor, his feet comes to rest instantaneously, so a very large force is exerted by the floor on his feet, but if he lands on sand, his feet push the sand for some distance; therefore, the time duration in which his feet comes to rest increases. As a result, the force exerted on his feet decreases and he is saved from getting hurt.
Solution D.1
Solution D.2
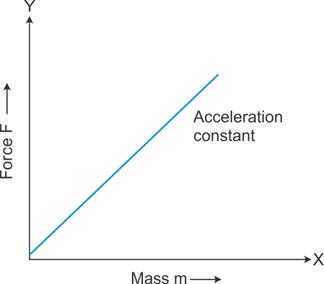
If a given force is applied on bodies of different masses, then the acceleration produced in them is inversely proportional to their masses.
A graph plotted for acceleration (a) against mass (m) is a hyperbola.
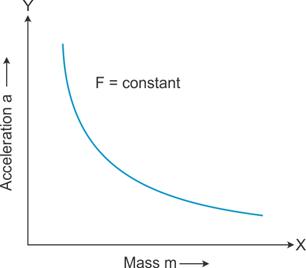
Solution D.3
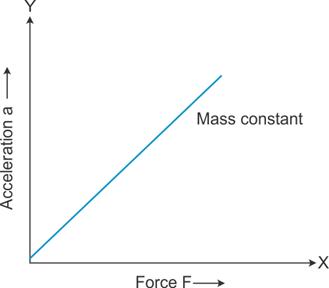
The relationship between force and acceleration for a given body with a constant mass, as described by Newton's second law of motion, can be illustrated with a graph of a straight line passing through the origin with a positive slope, indicating that as the force applied to the body increases, the acceleration of the body also increases proportionally.
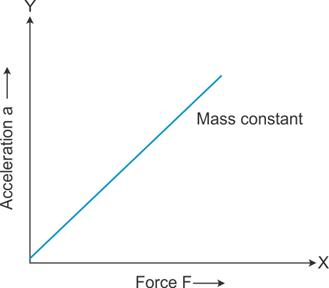
Solution E.1
Mass of the body, m = 5kg
Velocity, v = 2 m/s
Linear momentum = mv = (5)(2) kg m/s
= 10 kg ms-1
Solution E.2
Linear momentum = 0.5 kg m/s
Mass, m = 50 g = 0.05 kg
Velocity = Linear momentum/mass
= 0.5/0.05 m/s
= 10 ms -1
Solution E.3
Force, F = 15 N
Mass, m = 2kg
Acceleration, a = F/m [ From Newton's second law]
Or, a = (15/2) ms-2
Or, a = 7.5 ms-2
Solution E.4
Force, F = 10 N
Mass, m = 5kg
Acceleration, a = F/m [ From Newton's second law]
Or, a = (10/5) ms-2
Or, a = 2 ms-2
Solution E.5
Mass, m = 0.5 kg.
Acceleration, a = 5 ms-2
Force, F = ma [ From Newton's second law]
Or, F = (0.5) (5) N = 2.5 N.
Solution E.6
Force, F = 10 N
Mass, m = 2 kg
Time, t = 3 s
Initial velocity, u = 0 m/s.
(i) Let v be the final velocity acquired.
From Newton's second law,
F = ma.
Or, a = F/m = 10/2 = 5 ms-2.
From the 1st equation of motion,
a = (v![]() u)/t
u)/t
Or, v = at + u.
Or, v = (5)(3) + 0 = 15 ms-1
(ii) Change in momentum = Final momentum ![]() initial momentum
initial momentum
![]() p = mv
p = mv ![]() mu.
mu.
Or, ![]() p = m (v
p = m (v![]() u).
u).
Or, ![]() p = 2 ( 15
p = 2 ( 15 ![]() 0) = 30 kg ms-1
0) = 30 kg ms-1
Solution E.7
Mass, m = 100 kg
Distance moved, s = 100 m
Initial velocity, u = 0
(i) Because the body moves through a distance of 100 m in 5 s,
Velocity of the body = Distance moved / time taken
Velocity = (100/5) = 20 ms-1
(ii) From Newton's third equation of motion,
v2 ![]() u2 = 2as.
u2 = 2as.
Or, a = (v2 ![]() u2) /2s.
u2) /2s.
Or, a = [ (202 ![]() 02)/ 2(100) ] ms-2.
02)/ 2(100) ] ms-2.
Or, a = 2 ms-2.
(iii) Force, F = ma
Or, F = (100) (2) N.
Or, F = 200 N.
Solution E.8
Slope of a velocity-time graph gives the value of acceleration.
Here, slope = 20/5 = 4 m/s2.
Or, acceleration, a = 4 m/s2.
Force = Mass × Acceleration.
Given mass, m = 100 g = 0.1 kg.
Force = (0.1) (4) = 0.4 N.
Solution E.9
Let the force be F.
Force F causes an acceleration, a = 10 m/s2 in a body of mass, m = 500 g or 0.5 kg
Thus, F = ma
Or, F = (0.5) (10) = 5 N
Let a' be the acceleration which force F (=5N) cause on a body of mass, m' = 5 kg.
Then, a' = F/m'.
Or, a' = (5/5) ms-2.
Or, a' = 1 ms-2.
Solution E.10
Mass of cricket ball, m = 150 g = 0.15 kg
Initial velocity of ball, u = 25 m/s
Final velocity, v = 0
Time taken to bring ball to rest = 0.03 s
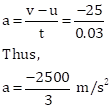
F = ma = 0.15 x (-2500)/3
F = - 125 N
Solution E.11
Mass, m = 2 kg
Initial velocity, u = 0
Final velocity, v = 2 m/s
Time, t = 0.1 s
Acceleration = Change in velocity/time
Or, a = (v![]() u) /t
u) /t
Or, a = (2![]() 0)/ 0.1 = 20 ms-2.
0)/ 0.1 = 20 ms-2.
Force = Mass ![]() Acceleration
Acceleration
Or, F = (2) (20) = 40 N.
Solution E.12

Thus, the force applied to move the body is 1 Newton.
Solution E.13
Mass, m = 480 kg.
Initial velocity, u = 54 km/hr = 15 m/s.
Final velocity, v = 0.
Time, t = 10 s.
Acceleration = Change in velocity/time.
Or, a = (v![]() u)/t.
u)/t.
Or, a = (0![]() 15)/10 = -1.5 ms-2.
15)/10 = -1.5 ms-2.
Here, negative sign indicates retardation.
Now, Force = Mass ![]() Acceleration
Acceleration
Or, F = (480) (1.5) = 720 N.
Solution E.14
Initial velocity, u = 30 m/s
Final velocity, v = 0
Time, t = 2s
Force, F = 1500 N
Here, a = (v ![]() u)/t = (0
u)/t = (0 ![]() 30)/ 2 =
30)/ 2 = ![]() 15 ms-2. Here, negative sign indicates retardation.
15 ms-2. Here, negative sign indicates retardation.
Now, F = ma.
Or, m = F/a = (1500/ 15) = 100 kg.
(a) Change in momentum = Final momentum - Initial momentum
Or, ![]() p = m (v
p = m (v![]() u)
u)
Or, ![]() p = 100 (0
p = 100 (0 ![]() 30)
30)
Or, ![]() p = 3000 kg m/s-1
p = 3000 kg m/s-1
(b) Acceleration, a = (v![]() u)/t.
u)/t.
Or, a = (0 ![]() 30)/ 2 =
30)/ 2 = ![]() 15 ms-2,
15 ms-2,
Here, negative sign indicates retardation.
Thus, retardation = 15 ms-2.
(c) From Newton's second law of motion,
F = ma
Or, m = F/a = (1500/ 15) = 100 kg.
Solution E.15
Mass, m = 50 gm = 0.05 kg.
Initial velocity, u = 100 m/s.
Final velocity, v = 0.
Distance, s = 2cm = 0.02 m.
(i) Initial momentum = mu = (0.05) (100) = 5 kg m/s-1
(ii) Final momentum = mv = (0.05) (0) = 0 kg m/s.
(iii) Acceleration, a = (v2 ![]() u2)/2s.
u2)/2s.
Or, a = (02 ![]() 1002)/ 2(0.02).
1002)/ 2(0.02).
Or, a = ![]() 2.5
2.5 ![]() 105 ms-2.
105 ms-2.
Therefore, retardation is 2.5 ![]() 105 ms-2.
105 ms-2.
(iv) Force, F = ma
Or, F = (0.05 kg) (2.5 ![]() 105 ms-2)
105 ms-2)
Or, F = 12500 N
Laws of Motion Exercise Ex 3(D)
Solution A.1
(c) Explains the way the force acts on a body.
Solution A.2
(a) Newton's third law
Newton's third law explains how a force acts on an object.
Solution A.3
(b) an equal and opposite reaction
According to Newton's third law of motion, there is always an equal and opposite reaction to every action. The action and reaction act simultaneously on two different bodies.
Solution A.4
(b) Different bodies in opposite directions
Solution A.5
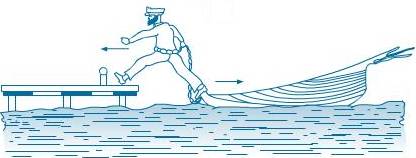
(c) Newton's third law
The motion of a boat moving away from the shore is not directly related to Newton's laws of motion.
Solution A.6
(c) ![]()
In an interaction of two bodies, A and B, the magnitude of action, i.e. the force FAB applied by body B on body A, is equal in magnitude to the reaction, i.e., the force FBA applied by body A on body B, but they are in directions opposite to each other.
Solution A.7
(b) 10 N
According to Newton's third law of motion, to every action there is always an equal and opposite reaction.
Hence the force experienced by the footballer will also be 10 N.
Solution A.8
(b) action and reaction act on different bodies in opposite direction.
According to Newton's third law, the action and reaction forces always occur in pairs and act on different bodies, with the action force and reaction force having equal magnitudes but opposite directions.
Solution A.9
(a) (iii)
The motion of a man on the ground is also an example of Newton's third law of motion. When a person walks or runs on the ground, their feet exert a backward force on the ground, and the ground exerts an equal and opposite reaction force forward, propelling the person forward.
Solution B.1
According to Newton's third law of motion, to every action there is always an equal and opposite reaction. The action and reaction act simultaneously on two different bodies.
Solution B.2
(a) Action: Force exerted on the bullet.
Reaction: Recoil experienced by the gun.
(b) Action: The force exerted by the hammer on the nail.
Reaction: The force applied by the nail on the hammer.
(c) Action: Weight of the book acting downwards.
Reaction: Force acted by the table upwards.
(d) Action: Force exerted by the rocket on the gases backwards.
Reaction: Force exerted by outgoing gases on the rocket in forward direction.
(e) Action: Force exerted by the feet on the ground in backward direction.
Reaction: Force exerted by the ground on feet in forward direction.
(f) Action: Force exerted by a moving train on a stationary train.
Reaction: Force exerted by a stationary train on a moving train.
Solution B.3
Yes, action and reaction act simultaneously.
Solution B.4
Yes, action and reaction are equal in magnitude.
Solution B.5
The given statement is wrong.
Reason: According to Newton's third law of motion, the action and reaction act simultaneously on different bodies. Hence they do not cancel each other.
Solution C.1
Newton's third law explains how a force acts on an object.
Solution C.2
Law of action and reaction: In an interaction of two bodies A and B, the magnitude of action, i.e. the force FAB applied by the body B on the body A, is equal in magnitude to the reaction, i.e., the force FBA applied by the body A on the body B, but they are in directions opposite to each other.
Examples:
(i) When a book is placed on a table, it does not move downwards. It implies that the resultant force on the book is zero, which is possible only if the table exerts an upward force of reaction on the book, equal to the weight of the book.
(ii) While moving on the ground, we exert a force by our feet to push the ground backwards; the ground exerts a force of the same magnitude on our feet forward, which makes it easier for us to move.
Explanation: In the above stated example, there are two objects and two forces. In the first example, the weight of the book acts downwards (action) and the force of the table acts upwards (reaction).
In the second example, our feet exerts a force on the ground (action) and the ground exerts an equal and opposite force (reaction) on our feet.
Solution C.3
When a rocket moves in space, it pushes gases outside, i.e. the rocket applies force on the gases in the backward direction. As a reaction, the gases put equal amount of force on the rocket in the opposite direction and the rocket moves in the forward direction.

Solution C.4
When a man fires a bullet from a gun, a force F is exerted on the bullet (action), and the gun experiences an equal and opposite recoil (reaction) and hence gets recoiled.

Solution C.5
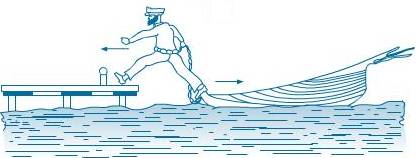
When a man exerts a force (action) on the boat by stepping into it, its force of reaction makes him step out of the boat, and the boat tends to leave the shore due to the force exerted by the man (i.e. action).
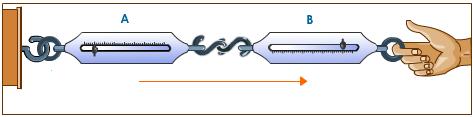
Solution C.6
Couple two spring balances A and B as shown in the figure. When we pull the balance B, both the balances show the same reading indicating that both the action and reaction forces are equal and opposite. In this case, the pull of either of the two spring balances can be regarded as action and that of the other balance as the reaction.
Solution C.7
To move a boat, the boatman pushes (action) the water backwards with his oar. In this response, the water exerts an equal and opposite force (reaction) in the forward direction on the boat due to which the boat moves ahead.
Solution C.8
A person pushing a wall hard (action) by his palm, experiences a force (reaction) exerted by the wall on his palm in the opposite direction; thus, he is liable to fall backwards.
Solution C.9
When a falling ball strikes the ground, it exerts a force on the ground. The ground exerts a force back at the ball in the opposite direction. This is the reason the ball rises upwards.
Solution D.1
The wall exerts an equal force of 10 N on the boy in the opposite direction, i.e. west.
Solution D.2
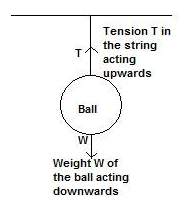
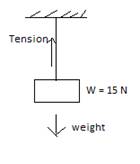
(a) A block exerts 15 N force (weight) on the string downwards.
(b) The string exerts an equal force of 15 N on the block in the opposite direction, i.e. upward direction (tension).
Laws of Motion Exercise Ex 3(E)
Solution A.1
(b) always attractive
Gravitational force is always attractive.
Solution A.2
(b) Always attractive
Solution A.3
(c) Sir Isaac Newton
Newton's law of gravitation: Every particle in the universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them, and the direction of the force is along the line joining the masses.
Solution A.4
(d) 6.7 x 10-11 N
Solution A.5
(d) none of these
G is a constant of proportionality called the universal gravitational constant. It does not depend on any other factors.
Solution A.6
(b) 6.7 x 10-11 N m2 kg-2
Solution A.7
(d) it remains unchanged
G is a constant of proportionality called the universal gravitational constant. It does not depend on any other factors.
Solution A.8
(d) all of the above
The gravitational constant is defined as the force of attraction between two bodies of unit mass separated by a unit distance.
i.e., ![]()
Solution A.9
(d) 6.7 × 10 - 11 N
Plugging in the given values:
m1 = m2 = 1 kg
r = 1 m
G = 6.7 × 10-11 Nm2 kg- 2
Now,
![]()
![]()
F = 6.7 × 10-11 N
Solution A.10
(a) 1.6 × 10 - 9 N
Given that,
m1 = 60 kg
m2 = 40 kg
r = 10 m
G = 6.7 × 10-11 Nm2 kg- 2
Now,
![]()
![]()
F = 1.6 × 10-11 N
Solution A.11
(b) m s -2
S.I unit of gravitational acceleration is m/s2.
Solution A.12
(c)![]()
Acceleration due to gravity (g) is directly proportional to the universal gravitational constant (G).
i.e., ![]()
Solution A.13
(c) both (a) and (b)
Now, if M is the planet's mass and R is its radius, the acceleration due to gravity at its surface will be expressed as.
![]()
Hence both options a and b are correct among the following.
Solution A.14
(c)
![]()
METHOD: Let't' be the time in which the body reaches its maximum height.
Initial velocity = u.
Final velocity (at the highest point) = 0.
Acceleration due to gravity = ![]() g (negative sign indicates the body is moving against gravity).
g (negative sign indicates the body is moving against gravity).
Using the first equation of motion,
v = u + gt.
We get,
0 = u ![]() gt
gt
Or t = u/g
Now total time for which the ball remains in air = Time of ascent + Time of descent
Because time of ascent = Time of descent,
Total time taken = u/g + u/g = 2u/g
Solution A.15
(c) 19.6 m s-1
METHOD: Given, u = 0
g = 9.8 m/s2
Time t = 2s
Let 'v' be the velocity of object on reaching the ground.
Using the first equation of motion,
v = u + gt
We get,
v = 0 + (9.8) (2)
Or, v = 19.6 m/s.
Solution A.16
(c) 40 N
Given:
Mass of the body (m) = 25 kg
Acceleration due to gravity on the moon (g) = 1.6 m/s2
Weight of the body on the moon = 25 × 1.6 = 40 N
Solution A.17
(c) W = mg
Solution A.18
(c) Newton
The S.I. unit of weight is Newton.
Solution A.19
(c) changes when the velocity of the body is close to the velocity of light
According to the theory of special relativity, the mass of a body does change when the velocity of the body is close to the velocity of light.
Solution B.1
Newton's law of gravitation: Every particle in the universe attracts every other particle with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between them, and the direction of the force is along the line joining the masses.
Solution B.2
Gravitational force is always attractive.
Solution B.3
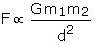
Here G is a constant of proportionality called the universal gravitational constant.
Solution B.4
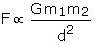
If the distance between the masses becomes half, the force reduces to one-fourth.
Solution B.5
The gravitational constant is defined as the force of attraction between two bodies of unit mass separated by a unit distance.
Solution B.6
The value of G in the S.I. system is 6.67 x 10-11Nm2kg-2.
Solution B.7
The rate at which the velocity of a freely falling body increases is called acceleration due to gravity. Its S.I. unit is m/s2.
Solution B.8
The average value of 'g' on the Earth's surface is 9.8 m/s2.
Solution B.9
Acceleration due to gravity (g) is directly proportional to universal gravitational constant (G).
Solution B.10
Initial velocity, u = 0.
Time taken = t.
Acceleration due to gravity = g.
Let 'h' be the height fallen.
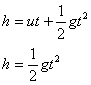
Solution B.11
If a body is thrown vertically up with an initial velocity u to a height h, then there will be retardation (a = ![]() g).
g).
At the highest point, the final velocity v = 0.
Thus, from the third equation,

Solution B.12
The S.I. unit of mass is kg and that of weight is newton.
Solution B.13
W = mg
At the centre of Earth, g = 0.
Therefore, W = 0.
Solution B.14
Mass of a body is always constant.
Solution C.1
The gravitational force of attraction between two masses is inversely proportional to the square of distance between them.
Solution C.2
The gravitational force of attraction is significant to explain the motion of heavenly bodies, e.g. motion of planets around the Sun, motion of the Moon around the Earth etc.
Solution C.3
The force with which the Earth attracts a body towards its centre is called the force due to gravity.
Solution C.4
The force due to gravity on a body of mass m kept on the surface of Earth (mass=M and radius=R) is equal to the force of attraction between the Earth and that body.
![]()
Solution C.5
Let g be the acceleration due to gravity on the Earth's surface (mass = M and radius = R).
Then, ![]()
Solution C.6
Mass: The mass of a body is the quantity of matter it contains.
Weight: The weight of a body is the force with which the Earth attracts it.
Solution C.7
Mass is a scalar quantity, but weight is a vector quantity. Mass is the measure of the quantity of matter contained in a body, but weight is the measure of force with which the Earth attracts the body. Mass of a body is always constant but weight varies from place to place.
Solution C.8
1 kgf = 9.8 N.
One kilogramme force is the force due to gravity on a mass of 1 kilogramme.
Solution D.1
Given the force of attraction between two bodies = 10 N
Now, ![]() .
.
If the new distance R'= R/2, then let F' be the force acting between the bodies. Then:
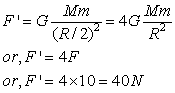
Solution D.2
Weight = mg
W = (5) (9.8) = 50 N.
Assumption: Value of acceleration due to gravity = 9.8 m/s2.
Solution D.3
Mass = 10 kg
(i) Weight (in kgf) = 10 x 1 kgf = 10 kgf
[1 kgf = 9.8 N]
(ii) Weight (in newton) = 10 x 9.8 = 98 N.
Solution D.4
Mass = 5 kg.
g = 9.8 m/s2.
Let F be the force of gravity,
F = mg.
F = (5) (9.8) = 49 N.
Force of gravity always acts downwards.
Solution D.5
Weight, W = 2.0 N
g = 9.8 m/s2
Let 'm' be the mass of the body.
W = mg
Or, m = W/g = (2/9.8) kg = 0.2 kg.
Solution D.6
Weight of the body on Earth = 98 N.
Acceleration due to gravity on Earth = 9.8 m/s2.
Let 'm' be the mass of the body on Earth.
m = W/g
m = (98/9.8) = 10 kg
Thus, the mass of the body is 10 kg, which always remains constant.
(a) Mass on moon = mass on Earth = 10 kg
(b) Let weight on moon is W'.
W' = mass ![]() acceleration due to gravity on the Moon.
acceleration due to gravity on the Moon.
[Given, acceleration due to gravity on the Moon = 1.6 m/s2]
W' = 10 ![]() 1.6 =16 N.
1.6 =16 N.
Solution D.7
Man's weight on Earth = 600 N
Man's weight on the Moon = (1/6) man's weight on Earth;
Because the acceleration due to gravity on the Moon is 1/6th that of Earth and w = mg.
Therefore, man's weight on Moon = (600/6) = 100 N.
Solution D.8
Mass, m = 10.5 kg
G = 10 m/s2
(a) Force, F = mg
F = (10.5) (10) = 105 N
(b) Weight, w = mg
w = (10.5) (10) = 105 N
Solution D.9
Let 'S' be the height.
Time taken, t = 3s; g = 9.8 m/s2
Initial velocity, u = 0 (because the body starts from rest)
(a) Using the second equation of motion,
S = ut + (1/2) gt2
We get,
S = 0 + (1/2) (9.8) (3) (3)
S = 44.1 m
(b) Let 'v' be the velocity with which the ball strikes the ground.
Using the third equation of motion,
v2 ![]() u2 = 2gs
u2 = 2gs
or, v2 - 02 = 2(9.8) (44.1)
or, v2 = 864.36
or, v = 29.4 m/s
Solution D.10
Mass, m = 5kg
Force, F = mg
F = (5) (9.8) = 49 N
Assumption: Value of acceleration due to gravity is 9.8 m/s2.
Solution D.11
Given, maximum height reached, s = 20 m
Acceleration due to gravity, g = 10 m/s2
(i) Let 'u' be the initial velocity.
At the highest point, velocity = 0
Using the third equation of motion,
v2 ![]() u2 = 2gs
u2 = 2gs
or, 0 ![]() u2 = 2 (10) (20) m/s
u2 = 2 (10) (20) m/s
or, u2 = ![]() (400) m/s [Negative sign indicates that the motion is against gravity]
(400) m/s [Negative sign indicates that the motion is against gravity]
or, u = 20 m/s
(b) Let v' be the final velocity of the ball on reaching the ground.
Considering the motion from the highest point to ground,
Velocity at highest point = 0 = Initial velocity for downward journey of the ball.
Distance travelled, s = 20m
Using the third equation of motion,
v2 ![]() u2 = 2gs
u2 = 2gs
or, v2 ![]() 0 = 2 (10) (20) m/s
0 = 2 (10) (20) m/s
or, v2 = 400 m/s
or, v = 20 m/s
(c) Now total time for which the ball remains in air, t = 2u/g.
Or, t = 2 (20)/(10).
Or, t = 4s.
Solution D.12
Initial velocity u = 0
Final velocity = 20 m/s
g = 10 m/s2
Let 'h' be the height of the tower.
Using the third equation of motion,
v2 ![]() u2 = 2gs
u2 = 2gs
or, (20)2 ![]() 0 = 2 (10) h
0 = 2 (10) h
or, h = 20 m
Solution D.13
Total time of journey = 6 s
g = 10 m/s2
(i) Let 'H' be the greatest height.
Time of ascent, t = 6/2 = 3 s,
For ascent, initial velocity, u = 0
Using the second equation of motion,
H = ut + (1/2) gt2
H = 0 + (1/2) (10) (3) 2
H = 45 m
(ii) Let u' be the initial velocity.
Final velocity, v = 0
Using the third equation of motion,
v2 ![]() u2 = 2gH
u2 = 2gH
or, v2 ![]() 0 = 2(10) (45)
0 = 2(10) (45)
or, v2 = 900
or, v = 30 m/s
Solution D.14
Initial velocity, u = 20 m/s
Time, t = 2s
g = 10 m/s2
Maximum height reached in 2s, H = (1/2) gt2
Or, H = (1/2) (10) (2) 2
Or, H = 20 m
Solution D.15
(a) Height, s = 80m
g = 10 m/s2
Using the second equation of motion,
S = ut + (1/2) gt2
Or, 80 = 0+ (1/2) (10) (t) 2
Or, (t) 2 = 16
Or, t = 4s
(b) Let 'v' be the velocity on reaching the ground.
Using the third equation of motion,
v2 ![]() u2 = 2gH
u2 = 2gH
or, v2 ![]() 0 = 2(10) (80)
0 = 2(10) (80)
or, v2 = 1600
or, v = 40 m/s
Solution D.16
Given time t = 2.5, g = 9.8 m/s2
Height, H = (1/2) gt2
Or, H = (1/2) (9.8) (2.5)2
Or, H = 30.6 m
Solution D.17
Initial velocity, u = 49 m/s
g = 9.8 m/s2
(i) Let H be the maximum height attained.
At the highest point, velocity = 0.
Using the third equation of motion,
v2 ![]() u2 = 2gH
u2 = 2gH
or,0 ![]() 492 = 2(
492 = 2(![]() 9.8) (H)
9.8) (H)
or, H = (492)/ 19.6
or, H = 122.5 m
(ii) Total time of flight is given by t = 2u/g
Or, t = 2(49)/ 9.8
Or, t = 10 s
Solution D.18
Initial velocity u = 0
Time t = 4 s
g = 10 m/s2
Let 'H' be the height of the tower.
Using the second equation of motion,
H = ut + (1/2) gt2
Or, H = 0 + (1/2)(10)(4)2
Or, H = 80 m
Solution D.19
(i) Time t =20 s
g = 10 m/s2
Let 'D' be the depth of the well.
Using the second equation of motion,
D = ut + (1/2) gt2
D = 0 + (1/2)(10)(20) 2
D = 2000 m
(ii) Speed of sound = 330 m/s
Depth of well = 2000 m
Time taken to hear the echo after the pebble reaches the water surface = Depth/speed
= (2000/330) s
= 6.1 s
Time taken for pebble to reach the water surface = 20 s.
Therefore, the total time taken to hear the echo after the pebble is dropped = 20 + 6.1 = 21.6 s.
Solution D.20
Let x be the height of the tower.
Let h be the distance from the top of the tower to the highest point as shown in the diagram.
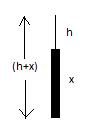
Initial velocity u = 19.6 m/s
g = 9.8 m/s2
At the highest point, velocity = 0
Using the third equation of motion,
v2 ![]() u2 = 2gh
u2 = 2gh
Or, ![]() (19.6) 2 = 2 (
(19.6) 2 = 2 (![]() 9.8) h
9.8) h
Or, h = 19.6 m
If the ball takes time t1 to go to the highest point from the top of building, then for the upward journey from the relation, v = u ![]() gt,
gt,
0 = 19.6 ![]() (9.8) (t1)
(9.8) (t1)
Or, t1 = 2s
(ii) Let us consider the motion for the part (x+h)
Time taken to travel from highest point to the ground = (5 ![]() 2) = 3s
2) = 3s
Using the equation s = ut + (1/2) gt2
We get,
(x + h) = 0 + (1/2) (9.8) (3) 2
Or, (x + 19.6) = 44.1 m
Or, x = 44.1 ![]() 19.6 = 24.5 m
19.6 = 24.5 m
Thus, height of the tower = 24.5 m
(iii) Let v be the velocity of the ball on reaching the ground.
Using the relation, v = u + gt
We get:
v = 0 + (9.8) (3)
Or, v = 29.4 m/s
