Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

Class 9 SELINA Solutions Maths Chapter 22: Trigonometrical Ratios [Sine, Consine, Tangent of an Angle and their Reciprocals]

Trigonometrical Ratios [Sine, Consine, Tangent of an Angle and their Reciprocals] Exercise Ex. 22(A)

Solution 1(a)

Correct option: (i)

 

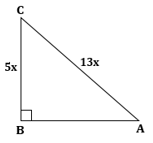
∴ If length of BC = 5x, length of AC = 13x

Now,

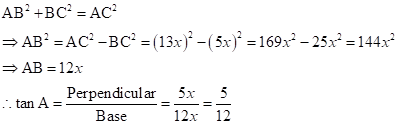
Solution 1(b)

Correct option: (ii) 1

 

 

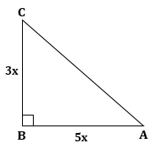
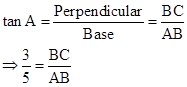
∴ If length of BC = 3x, length of AB = 5x

Now,

So,

 

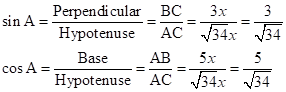
Solution 1(c)

Correct option: (iv) -1

 

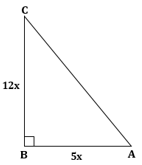
∴ If length of AB = 5x, length of BC = 12x

Now,

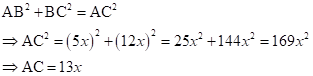
So,

 

Solution 1(d)

Correct option: (iii)  

In right-angled ΔADB,

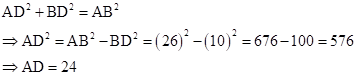
Now,

Solution 1(e)

Correct option: (i) 1

 

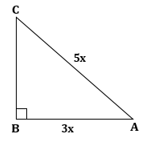
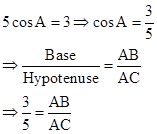
∴ If length of AB = 3x, length of AC = 5x

Now,

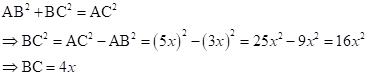
So,

 

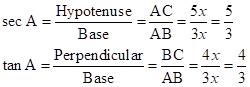
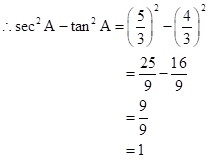
Solution 2

Given angle

 

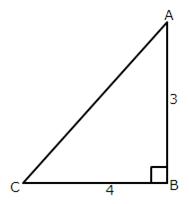
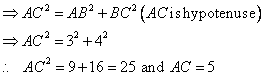
(i)

(ii)

(iii)

(iv)

(v)

(vi)

Solution 3

Given angle

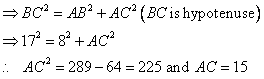
(i)

(ii)

(iii)

(iv)

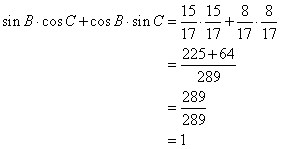
Solution 4

Consider the diagram as

 

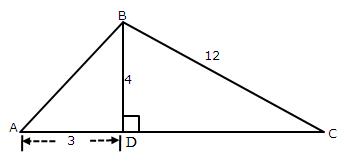
Given angle and

(i)

(ii)

(iii)

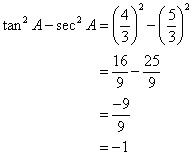
(iv)

(v)

(vi)

Solution 5

Given angle and

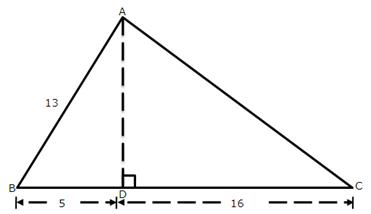
(i)

(ii)

(iii)

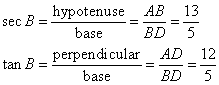
(iv)

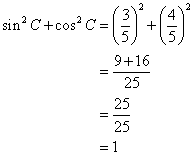
Solution 6

Consider the diagram below:

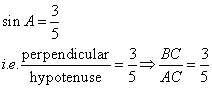
Therefore if length of , length of

Since

Now

(i)

(ii)

Solution 7

Given angle in the figure

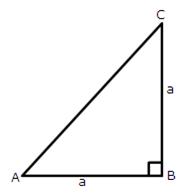
Now

(i)

(ii)

(iii)

 

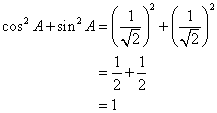
Solution 8

Consider the diagram below:

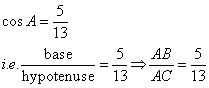
Therefore if length of , length of

Since

Now

(i)

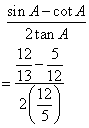
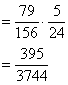
(ii)

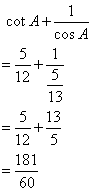
Solution 9

Consider the diagram below:

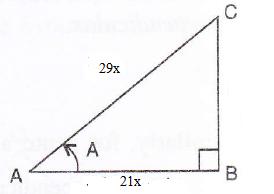
Therefore if length of , length of

Since

Now

Therefore

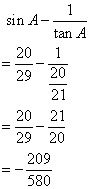
Solution 10

Consider the diagram below:


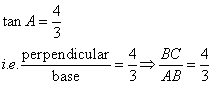
Therefore if length of A B equals 3 x, length of B C equals 4 x

Since


Now


Therefore

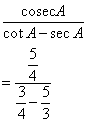
Solution 11

Consider the diagram below:

 


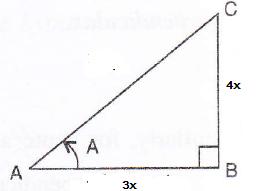
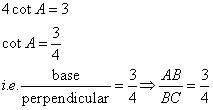
Therefore if length of AB = 3x, length of BC = 4x

Since

(i)

(ii)

(iii)

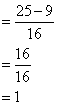
Solution 12

Consider the diagram below:


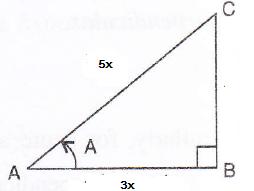
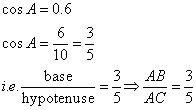
Therefore if length of AB = 3x, length of AC = 5x

Since


Now all other trigonometric ratios are

Solution 13

Consider the diagram below:


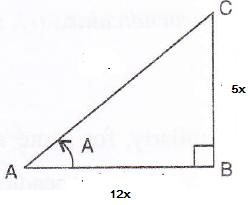
Therefore if length of AB = 12x, length of BC = 5x

Since


(i)

(ii)

(iii)

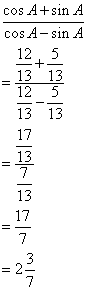
Solution 14

Consider the diagram below:


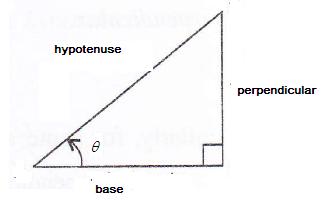
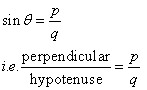
Therefore if length of perpendicular = px, length of hypotenuse = qx


Since


Now


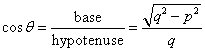
Therefore

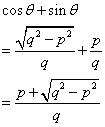
Solution 15

Consider the diagram below:


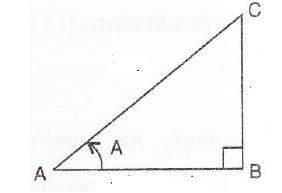
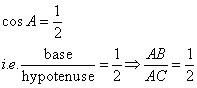
Therefore if length of AB = x, length of AC = 2x

Since


Consider the diagram below:

 


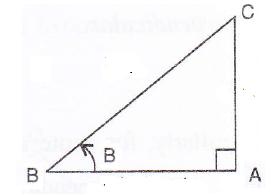
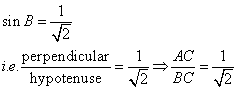
Therefore if length of AC = x, length of B C equals square root of 2 x 

Since


Now


Therefore

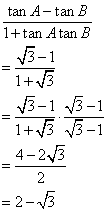
Trigonometrical Ratios [Sine, Consine, Tangent of an Angle and their Reciprocals] Exercise Ex. 22(B)

Solution 1(a)

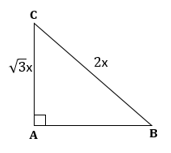
Correct option: (i)

 

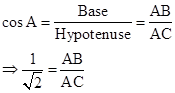
∴ If length of AB = x, length of AC = x

Now,

 

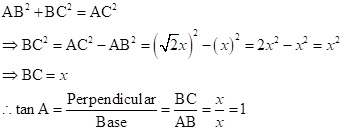
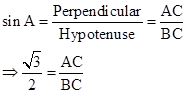
∴ If length of AC = x, length of BC = 2x

Now,

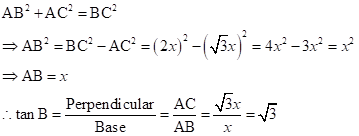
Solution 1(b)

Correct option: (iii)  

 

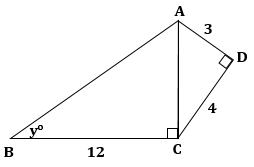
In right-angled ΔADC,

In right-angled ΔACB,

 

Now,

Solution 1(c)

Correct option: (i)  

 

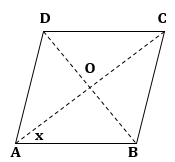
Let the diagonals BD and AC of a rhombus intersect each other at O.

Given, BD = 12 cm, AC = 16 cm.

Diagonals of a rhombus bisect each other at right angles.

⇒ OB = OD = 6 cm, OA = OC = 8 cm and ∠AOB = 90o

In right-angled ΔAOB,

Now,

Solution 1(d)

Correct option: (iv) 6

In right-angled ΔADB,

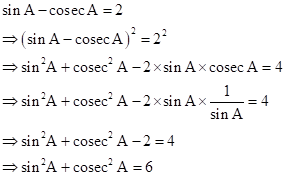
Solution 1(e)

Correct option: (ii)  

 

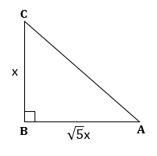
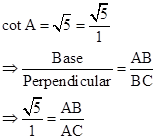
∴ If length of AB = x, length of BC = x

Now,

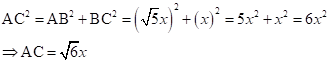
So,

 

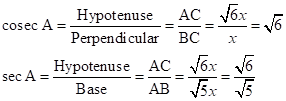
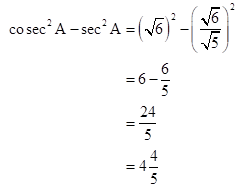
Solution 2

Consider the given figure

 

 

(i)

Since the triangle is a right angled triangle, so using Pythagorean Theorem

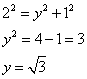
(ii)

(iii)

Therefore

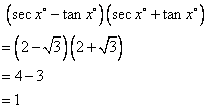
Solution 3

Consider the given figure

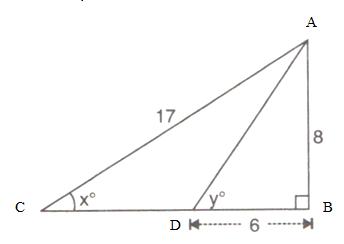
Since the triangle is a right angled triangle, so using Pythagorean Theorem

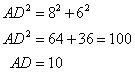
Also

(i)

(ii)

(iii)

Therefore

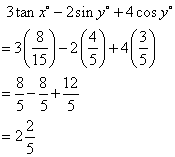
Solution 4

Consider the given figure

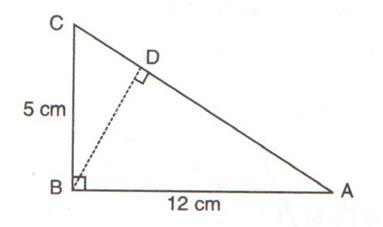
Since the triangle is a right angled triangle, so using Pythagorean Theorem

In and , the is common to both the triangles, so therefore.

Therefore and are similar triangles according to AAA Rule

So

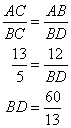
(i)

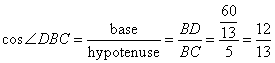
(ii)

Solution 5

Consider the given figure

 

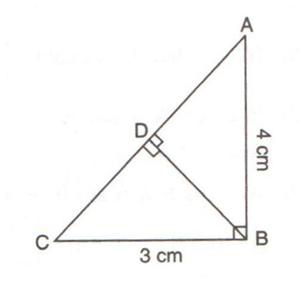
Since the triangle is a right angled triangle, so using Pythagorean Theorem

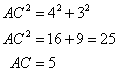
In and , the is common to both the triangles, so therefore.

Therefore and are similar triangles according to AAA Rule

So

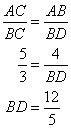
Now using Pythagorean Theorem

Therefore


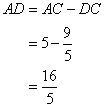
(i) 

 

 

 

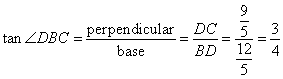
(ii)

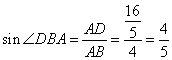
Solution 6

Consider the figure below

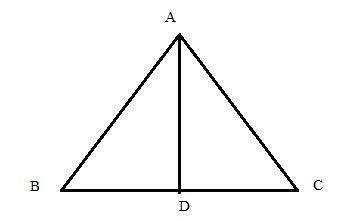
In the isosceles , and the perpendicular drawn from angle to the side divides the side into two equal parts

Solution 7

Consider the figure below

In the isosceles , and the perpendicular drawn from angle to the side divides the side into two equal parts

Since

(i)

(ii)

(iii)

Therefore

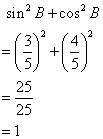
(iv)

Therefore

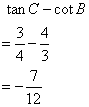
Solution 8

Consider the figure


Therefore if length of base = 4x, length of perpendicular = 3x


Since


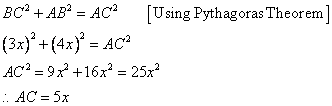
Now


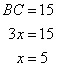
Therefore


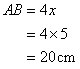
And

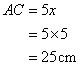
Solution 9

Consider the figure

 

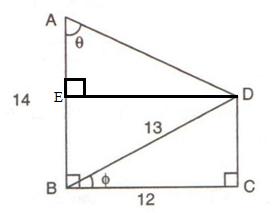
A perpendicular is drawn from D to the side AB at point E which makes BCDE is a rectangle.

Now in right angled triangle BCD using Pythagorean Theorem

Since BCDE is rectangle so ED 12 cm, EB = 5 and AE = 14 - 5 = 9 

(i)

(ii)

Or

Solution 10

Given



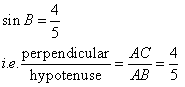
Therefore if length of perpendicular = 4x, length of hypotenuse = 5x


Since

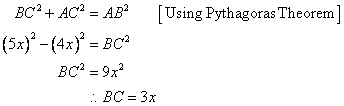
Now

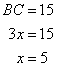
(i)

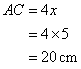
And

(ii)

Given


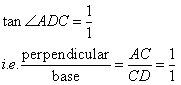
Therefore if length of perpendicular = x, length of hypotenuse = x


Since

Now

So

And


Now


So

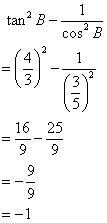
Solution 11

Squaring both sides

Solution 12

Squaring both sides

Solution 13

Consider the diagram below:


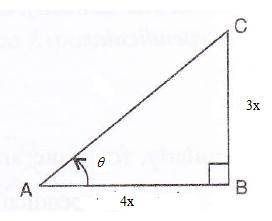
Therefore if length of BC = 3x, length of AB = 4x


Since

(i)

(ii)

(iii)

Therefore

(iv)

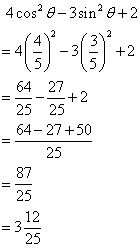
Solution 14

Consider the diagram below:


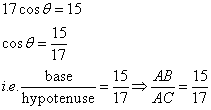
Therefore if length of AB = 15x, length of AC = 17x


Since


Now


Therefore

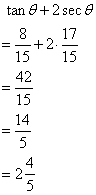
Solution 15

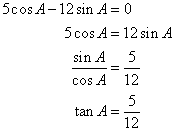
Now

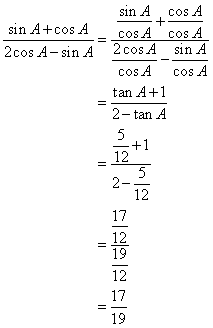
Trigonometrical Ratios [Sine, Consine, Tangent of an Angle and their Reciprocals] Exercise Test Yourself

Solution 1

Consider the diagram below:


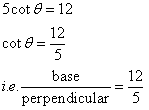
Therefore if length of base = 12x, length of perpendicular = 5x


Since


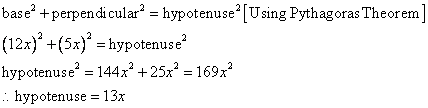
Now


Therefore

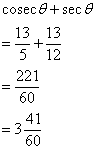
Solution 2

Consider the diagram below:


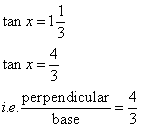
Therefore if length of base = 3x, length of perpendicular = 4x


Since


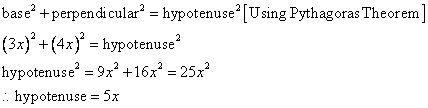
Now


Therefore

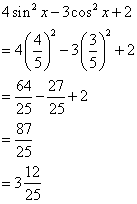
Solution 3

Consider the diagram below:

 


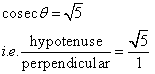
Therefore if length of hypotenuse equals square root of 5 x, length of perpendicular = x

Since


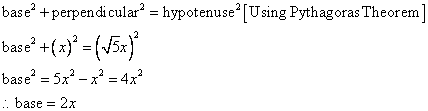
Now

(i)

 

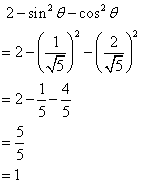
(ii)

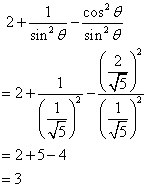
Solution 4

Consider the diagram below:


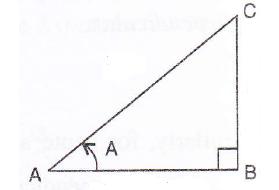
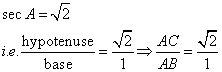
Therefore if length of AB = x, length of A C equals square root of 2 x 

Since


Now


Therefore

Solution 5

Consider the diagram below:


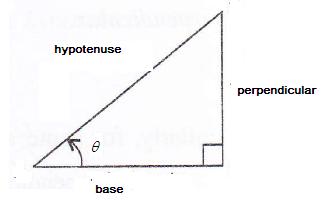
Therefore if length of base = x, length of perpendicular = x


Since


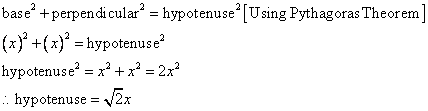
Now


Therefore

Solution 6

Given angle and in the figure


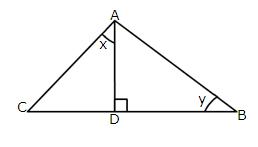
Again


Now

(i)

(ii)


Therefore


(iii)


Therefore

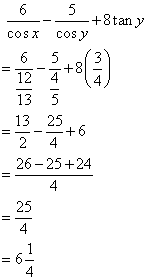
Solution 7

Since is mid-point of so

(i)

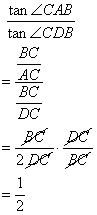
(ii)

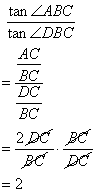
Solution 8

Consider the diagram below:


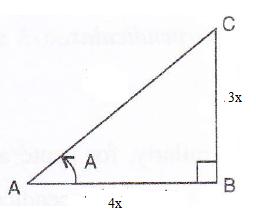
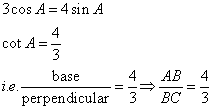
Therefore if length of AB = 4x, length of BC = 3x


Since


(i)


(ii)


Therefore

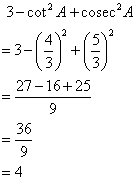
Solution 9

Consider the figure


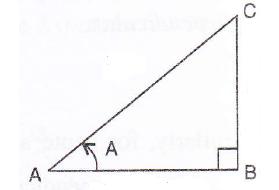
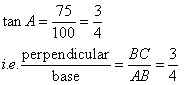
Therefore if length of base = 4x, length of perpendicular = 3x


Since


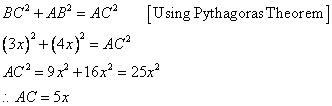
Now


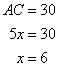
Therefore


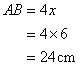
And

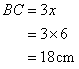
Solution 10

Consider the figure

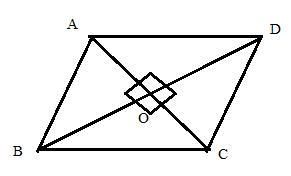
The diagonals of a rhombus bisects each other perpendicularly


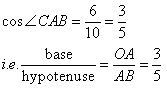
Therefore if length of base = 3x, length of hypotenuse = 5x


Since


Now


Therefore

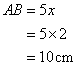
And


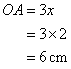
Since the sides of a rhombus are equal so the length of the side of the rhombus


The diagonals are

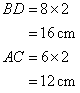
Solution 11

Consider the figure below

In the isosceles , the perpendicular drawn from angle to the side divides the side into two equal parts

Since

(i)

(ii)

(iii)

Therefore

Solution 12

Consider the figure below


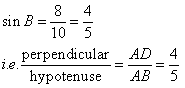
Therefore if length of perpendicular = 4x, length of hypotenuse = 5x


Since


Now


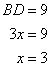
Therefore


And

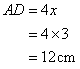
Again


Therefore if length of perpendicular = x, length of base = x


Since


Now


Therefore


And

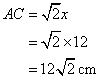
Solution 13

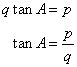
Now

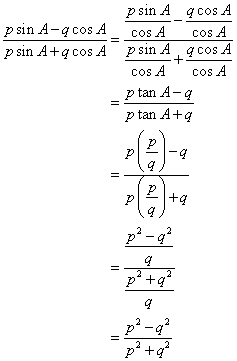
Solution 14

Consider the figure


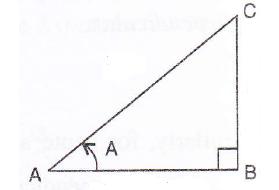
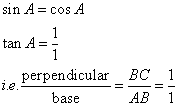
Therefore if length of perpendicular = x, length of base = x


Since


Now


Therefore

Solution 15

Consider the diagram


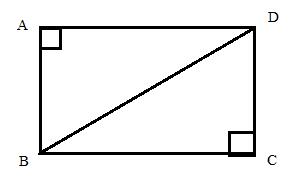
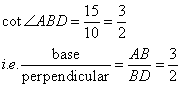
Therefore if length of base = 3x, length of perpendicular = 2x


Since


Now


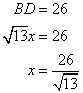
Therefore


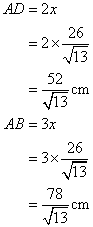
Now

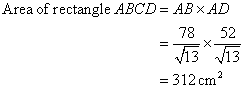
Solution 16

Consider the figure

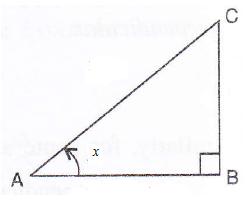
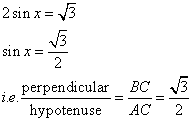
Therefore if length of , length of

Since

Now

(i)

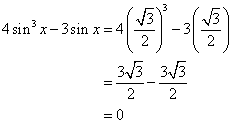
(ii)

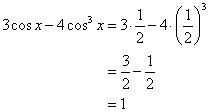
Solution 17

Consider the diagram below:

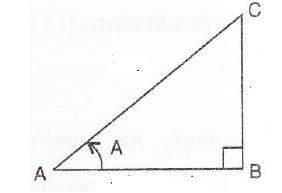
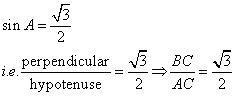
Therefore if length of , length of

Since

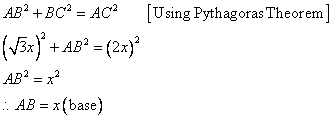
Consider the diagram below:

 

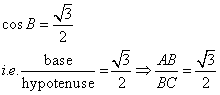
Therefore if length of , length of

Since

Now

Therefore

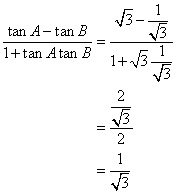
Solution 18

Consider the figure

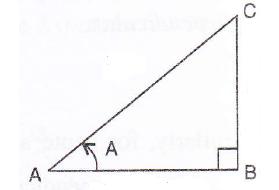
Therefore if length of , length of

Since

Now

Therefore

Solution 19

Now

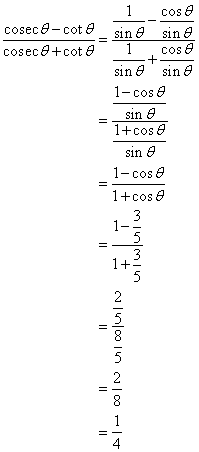
Solution 20

Squaring both sides

Solution 21

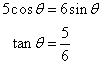
Now

(i)

(ii)

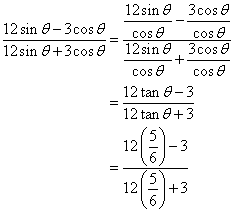
Solution 22

Consider the given diagram as

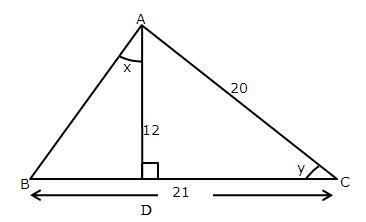
Using Pythagorean Theorem

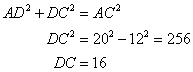
Now

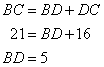
Again using Pythagorean Theorem

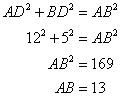
Now

Therefore

My Class Videos Tests ₹ Plans Ask a Doubt