Class 9 SELINA Solutions Maths Chapter 11: Inequalities
Inequalities Exercise Ex. 11
Solution 1(a)
Correct option: (ii) BC > AB
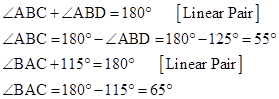
In DABC,
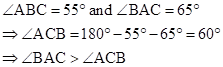
If two angles of a triangle are unequal, the greater angle has the greater side opposite to it.
Solution 1(b)
Correct option: (iii) BD > DC
BD = AD [Given]
In ΔABD,
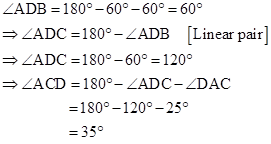
If two angles of a triangle are unequal, the greater angle has the greater side opposite to it.
So, in ΔADC,
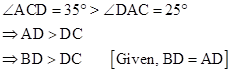
Solution 1(c)
Correct option: (iv) BD > CD
AB = AC [Given]
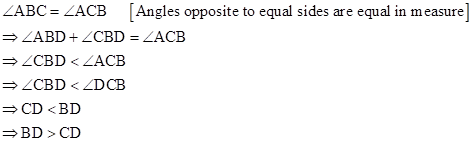
Solution 1(d)
Correct option: (ii) BD < AB
In ΔABC,
Given, 
And,
Now, in ΔABD,
If two angles of a triangle are unequal, the greater angle has the greater side opposite to it.
Here,
Solution 1(e)
Correct option: (ii) AC > AB
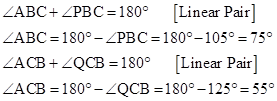
If two angles of a triangle are unequal, the greater angle has the greater side opposite to it.
In ΔABC,
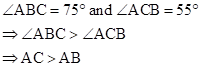
Solution 1(f)
Correct option: (i) AB + BC + CD + DA > AC + BD
Consider quadrilateral ABCD as follows:
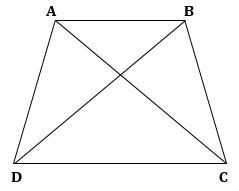
The sum of the lengths of any two sides of a triangle is always greater than the third side.
AB + BC > AC (I)
BC + CD > BD (II)
CD + DA > AC (III)
DA + AB > BD (IV)
Adding equations (I), (II), (III), (IV),
2AB + 2BC + 2CD + 2DA > 2AC + 2BD
⇒ 2(AB + BC + CD + DA) > 2(AC + BD)
⇒ AB + BC + CD + DA > AC + BD
Solution 2
In 
ABC,
AB = AC[Given]
ACB = B[angles opposite to equal sides are equal]
B = 700[Given]
ACB = 700 ……….(i)
Now,
ACB +ACD = 1800[ BCD is a straight line]
700 + ACD = 1800
ACD = 1100 …………(ii)
In ACD,
CAD + ACD + D = 1800
CAD + 1100 + D = 1800 [From (ii)]
CAD + D = 700
But D = 400 [Given]
CAD + 400= 700
CAD = 300 ………………(iii)
In ACD,
ACD = 1100[From (ii)]
CAD = 300[From (iii)]
D = 400 [Given]
[Greater angle has greater side opposite to it]
Also,
AB = AC[Given]
Therefore, AB > CD.
Solution 3
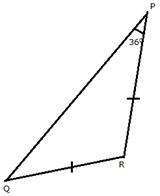
In 
PQR,
QR = PR[Given]
P = Q[angles opposite to equal sides are equal]
P = 360[Given]
Q = 360
In PQR,
P + Q + R = 1800
360 + 360 + R = 1800
R + 720 = 1800
R = 1080
Now,
R = 1080
P = 360
Q = 360
Since R is the greatest, therefore, PQ is the largest side.
Solution 4
The sum of any two sides of the triangle is always greater than third side of the triangle.
Third side < 13
+8 =21 cm.
The difference between any two sides of the triangle is always less than the third side of the triangle.
Third side > 13-8 =5 cm.
Therefore, the length of the third side is between 5 cm and 9 cm, respectively.
The value of a =5 cm and b= 21cm.
Solution 5
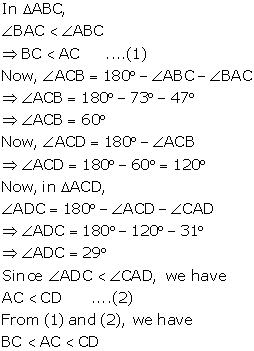
Solution 6
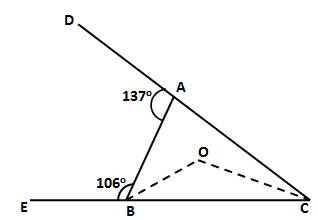
Solution 7
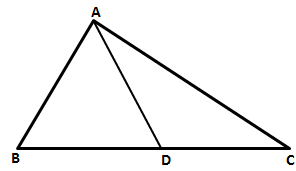
Solution 8
In 
BEC,
B + BEC + BCE = 1800
B = 650 [Given]
BEC = 900[CE is perpendicular to AB]
650 + 900 + BCE = 1800
BCE = 1800 - 1550
BCE = 250 = DCF …………(i)
In CDF,
DCF + FDC + CFD = 1800
DCF = 250 [From (i)]
FDC = 900[AD is perpendicular to BC]
250 + 900 + CFD = 1800
CFD = 1800 - 1150
CFD = 650 …………(ii)
Now, AFC + CFD = 1800[AFD is a straight line]
AFC + 650 = 1800
AFC = 1150 ………(iii)
In ACE,
ACE + CEA + BAC = 1800
BAC = 600 [Given]
CEA = 900[CE is perpendicular to AB]
ACE + 900 + 600 = 1800
ACE = 1800 - 1500
ACE = 300 …………(iv)
In AFC,
AFC + ACF + FAC = 1800
AFC = 1150 [From (iii)]
ACF = 300[From (iv)]
1150 + 300 + FAC = 1800
FAC = 1800 - 1450
FAC = 350 …………(v)
In AFC,
FAC = 350[From (v)]
ACF = 300[From (iv)]
In CDF,
DCF = 250[From (i)]
CFD = 650[From (ii)]
Solution 9
ACB = 740 …..(i)[Given]
ACB + ACD = 1800[BCD is a straight line]
740 + ACD = 1800
ACD = 1060 ……..(ii)
In 
ACD,
ACD + ADC+ CAD = 1800
Given that AC = CD
ADC= CAD
1060 + CAD + CAD = 1800[From (ii)]
2CAD = 740
CAD = 370 =ADC………..(iii)
Now,
BAD = 1100[Given]
BAC + CAD = 1100
BAC + 370 = 1100
BAC = 730 ……..(iv)
In ABC,
B + BAC+ ACB = 1800
B + 730 + 740 = 1800[From (i) and (iv)]
B + 1470 = 1800
B = 330 ………..(v)
Solution 10
(i) 
ADC + ADB = 1800[BDC is a straight line]
ADC = 900[Given]
900 + ADB = 1800
ADB = 900 …………(i)
In 
ADB,
ADB = 900[From (i)]
B + BAD = 900
Therefore, B and BAD are both acute, that is less than 900.
AB > BD …….(ii)[Side opposite 900 angle is greater than
side opposite acute angle]
(ii) In ADC,
ADB = 900
C + DAC = 900
Therefore, C and DAC are both acute, that is less than 900.
AC > CD ……..(iii)[Side opposite 900 angle is greater than
side opposite acute angle]
Adding (ii) and (iii)
AB + AC > BD + CD
AB + AC > BC
Solution 11
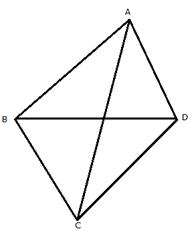
Const: Join AC and BD.
(i) In 
ABC,
AB + BC > AC….(i)[Sum of two sides is greater than the
third side]
In ACD,
AC + CD > DA….(ii)[ Sum of two sides is greater than the
third side]
Adding (i) and (ii)
AB + BC + AC + CD > AC + DA
AB + BC + CD > AC + DA - AC
AB + BC + CD > DA …….(iii)
(ii)In ACD,
CD + DA > AC….(iv)[Sum of two sides is greater than the
third side]
Adding (i) and (iv)
AB + BC + CD + DA > AC + AC
AB + BC + CD + DA > 2AC
(iii) In ABD,
AB + DA > BD….(v)[Sum of two sides is greater than the
third side]
In BCD,
BC + CD > BD….(vi)[Sum of two sides is greater than the
third side]
Adding (v) and (vi)
AB + DA + BC + CD > BD + BD
AB + DA + BC + CD > 2BD
Solution 12
(i) In 
ABC,
AB = BC = CA[ABC is an equilateral triangle]
A = B = C
In ABP,
A = 600
ABP< 600
[Side opposite to greater angle is greater]
(ii) In BPC,
C = 600
CBP< 600
[Side opposite to greater angle is greater]
Solution 13
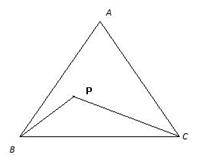
Let 
PBC = x and PCB = y
then,
BPC = 1800 - (x + y) ………(i)
Let ABP = a and ACP = b
then,
BAC = 1800 - (x + a) - (y + b)
BAC = 1800 - (x + y) - (a + b)
BAC =BPC - (a + b)
BPC = BAC + (a + b)
BPC > BAC
Solution 14
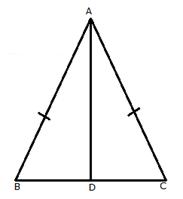
We know that exterior angle of a triangle is always greater than each of the interior opposite angles.
In 
ABD,
ADC > B ……..(i)
In ABC,
AB = AC
B = C …..(ii)
From (i) and (ii)
ADC > C
(i) In ADC,
ADC > C
AC > AD ………(iii) [side opposite to greater angle is greater]
(ii) In ABC,
AB = AC
AB > AD[ From (iii)]
Solution 15
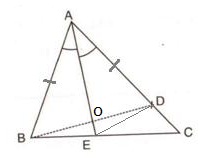
Const: Join ED.
In 
AOB and AOD,
AB = AD[Given]
AO = AO[Common]
BAO = DAO[AO is bisector of A]
[SAS criterion]
Hence,
BO = OD………(i)[cpct]
AOB = AOD .……(ii)[cpct]
ABO = ADO 
ABD = ADB ………(iii)[cpct]
Now,
AOB = DOE[Vertically opposite angles]
AOD = BOE[Vertically opposite angles]
BOE = DOE ……(iv)[From (ii)]
(i) In BOE and DOE,
BO = CD[From (i)]
OE = OE[Common]
BOE = DOE[From (iv)]
[SAS criterion]
Hence, BE = DE[cpct]
(ii) In BCD,
ADB = C + CBD[Ext. angle = sum of opp. int. angles]
ADB > C
ABD > C[From (iii)]
Inequalities Exercise Test Yourself
Solution 1
AB = AC (Given) (I)
⇒ ∠ABC = ∠ACB [Angles opposite to equal sides are equal]
⇒ ∠ABD = ∠ACD (II)
In DABD, exterior ∠ADC = ∠ABD + ∠BAD
⇒ ∠ADC > ∠ABD
⇒ ∠ADC > ∠ACD [From (II)]
Now, in any triangle, the side opposite to the largest angle is the largest.
⇒ AC > AD
⇒ AB > AD [From (I)]
Solution 2
AD = AC (Given)
⇒ ΔADC is an isosceles triangle.
⇒ ∠ADC = ∠ACD = acute angle
Now, ∠ADC + ∠ADB = 180o [Linear pair]
⇒ ∠ADB is an obtuse angle.
Therefore, in ΔABD, ∠ADB is the largest angle.
Now, in any triangle, the side opposite to the largest angle is the largest.
⇒ AB > AD
Solution 3
The sum of the lengths of any two sides of a triangle is always greater than the third side.
In ΔPQS,
PQ + QS >PS (I)
In ΔPSR,
SR + RP >PS (II)
Adding equations (I) and (II),
PQ + QS + SR + RP > PS + PS
⇒ PQ + QR + RP >2PS (proved)
Solution 4
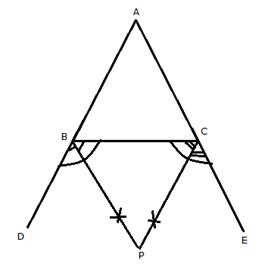
In 
ABC,
AB > AC,
ABC < ACB
1800 -ABC > 1800 -ACB
Solution 5
Since AB is the largest side and BC is the smallest side of the triangle ABC
Solution 6
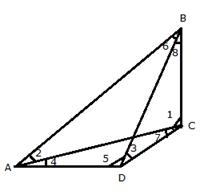
In the quad. ABCD,
Since AB is the longest side and DC is the shortest side.
(i) 
1 > 2[AB > BC]
7 > 4[AD > DC]
1 + 7 > 2 + 4
C > A
(ii) 5 > 6[AB > AD]
3 > 8[BC > CD]
5 + 3 > 6 + 8
D > B
Solution 7
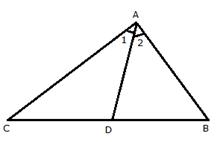
In 
ADC,
ADB = 1 + C.............(i)
In ADB,
ADC = 2 + B.................(ii)
But AC > AB[Given]
B > C
Also given, 2 = 1[AD is bisector of A]
2 + B > 1 + C …….(iii)
From (i), (ii) and (iii)
ADC > ADB
Solution 8
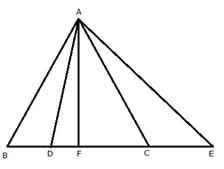
We know that the bisector of the angle at the vertex of an isosceles triangle bisects the base at right angle.
Using Pythagoras theorem in 
AFB,
AB2 = AF2 + BF2…………..(i)
In AFD,
AD2 = AF2 + DF2…………..(ii)
We know ABC is isosceles triangle and AB = AC
AC2 = AF2 + BF2 ……..(iii)[ From (i)]
Subtracting (ii) from (iii)
AC2 - AD2 = AF2 + BF2 - AF2 - DF2
AC2 - AD2 = BF2 - DF2
Let 2DF = BF
AC2 - AD2 = (2DF)2 - DF2
AC2 - AD2 = 4DF2 - DF2
AC2 = AD2 + 3DF2
AC2 > AD2
AC > AD
Similarly, AE > AC and AE > AD.
Solution 9
The sum of any two sides of the triangle is always greater than the third side of the triangle.
Solution 10
