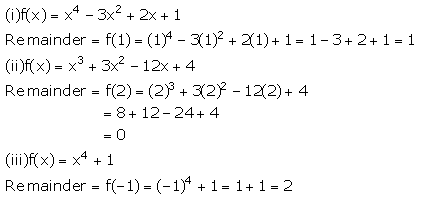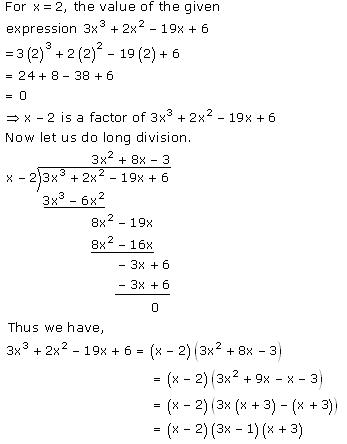Class 10 SELINA Solutions Maths Chapter 8 - Remainder And Factor Theorems
Remainder And Factor Theorems Exercise Ex. 8(A)
Solution 1(a)
Correct option: (i) –1
x – 1 = 0 ⇒ x = 1
Since x – 1 is a factor of 8x2 – 7x + m,
Remainder = 0
⇒ 8(1)2 – 7(1) + m = 0
⇒ 8 – 7 + m = 0
⇒ m = –1
Solution 1(b)
Correct option: (ii) (x – 2)
(x – 2)2 – (x2 – 4)
= (x – 2)2 – (x – 2)(x + 2)
= (x – 2)(x – 2 – x – 2)
= (x – 2)( –4)
Hence, (x – 2) is a factor of a given polynomial.
Solution 1(c)
Correct option: (iii) 6
x – 1 = 0 ⇒ x = 1
Since x – 1 is a factor of x3 – kx2 + 11x – 6,
Remainder = 0
⇒ (1)3 – k(1)2 + 11(1) – 6 = 0
⇒ 1 – k + 11 – 6 = 0
⇒ 6 – k = 0
⇒ k = 6
Solution 1(d)
Correct option: (iv) -5
x – a = 0 ⇒ x = a
Since x – a is a factor of x3 – ax2 + x + 5,
Remainder = 0
⇒ (a)3 – a(a)2 + a + 5 = 0
⇒ a3 – a3 + a + 5 = 0
⇒ a = –5
Solution 1(e)
Correct option: (i) x3 – x2 + x – 6
x – 2 = 0 ⇒ x = 2
Consider polynomial x3 – x2 + x – 6.
When x3 – x2 + x – 6 is divided by (x – 2),
Remainder = (2)3 – (2)2 + 2 – 6
= 8 – 4 + 2 – 6
= 0
Hence, (x – 2) is a factor of x3 – x2 + x – 6.
Solution 2
By remainder theorem we know that when a polynomial f (x) is divided by x - a, then the remainder is f(a).
Solution 3
(x - a) is a factor of a polynomial f(x) if the remainder, when f(x) is divided by (x - a), is 0, i.e., if f(a) = 0.
Solution 4
By remainder theorem we know that when a polynomial f (x) is divided by x - a, then the remainder is f(a).
Let f(x) = 2x3 + 3x2 - 5x - 6
(i) f (-1) = 2(-1)3 + 3(-1)2 - 5(-1) - 6 = -2 + 3 + 5 - 6 = 0
Thus, (x + 1) is a factor of the polynomial f(x).
(ii)

Thus, (2x - 1) is not a factor of the polynomial f(x).
(iii) f (-2) = 2(-2)3 + 3(-2)2 - 5(-2) - 6 = -16 + 12 + 10 - 6 = 0
Thus, (x + 2) is a factor of the polynomial f(x).
Solution 5
(i) 2x + 1 is a factor of f(x) = 2x2 + ax - 3.

(ii) 3x - 4 is a factor of g(x) = 3x2 + 2x - k.
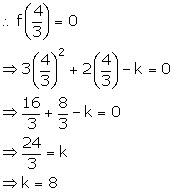
Solution 6
Let f(x) = x3 + ax2 + bx - 12
x - 2 = 0 ![]() x = 2
x = 2
x - 2 is a factor of f(x). So, remainder = 0
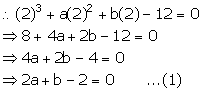
x + 3 = 0 ![]() x = -3
x = -3
x + 3 is a factor of f(x). So, remainder = 0
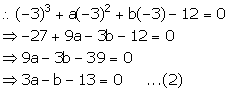
Adding (1) and (2), we get,
5a - 15 = 0
![]() a = 3
a = 3
Putting the value of a in (1), we get,
6 + b - 2 = 0
![]() b = -4
b = -4
Solution 7
Let f(x) = (3k + 2)x3 + (k - 1)
2x + 1 = 0 ![]()
![]()
Since, 2x + 1 is a factor of f(x), remainder is 0.
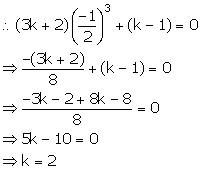
Solution 8
Let f(x) = x3 + (3m + 1) x2 + nx - 18
x - 1 = 0 ![]() x = 1
x = 1
x - 1 is a factor of f(x). So, remainder = 0
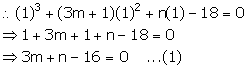
x + 2 = 0 ![]() x = -2
x = -2
x + 2 is a factor of f(x). So, remainder = 0
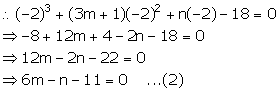
Adding (1) and (2), we get,
9m - 27 = 0
m = 3
Putting the value of m in (1), we get,
3(3) + n - 16 =0
9 + n - 16 = 0
n = 7
Solution 9
Let f(x) = x3 + 2x2 - kx + 4
x - 2 = 0 ![]() x = 2
x = 2
On dividing f(x) by x - 2, it leaves a remainder k.
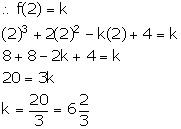
Solution 10
Let f(x) = ax3 + 9x2 + 4x - 10
x + 3 = 0 ![]() x = -3
x = -3
On dividing f(x) by x + 3, it leaves a remainder 5.
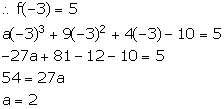
Solution 11
Let f(x) = x3 + ax2 + bx + 6
x - 2 = 0 ![]() x = 2
x = 2
Since, x - 2 is a factor, remainder = 0

x - 3 = 0 ![]() x = 3
x = 3
On dividing f(x) by x - 3, it leaves a remainder 3.
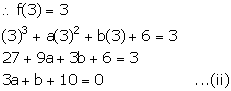
Subtracting (i) from (ii), we get,
a + 3 = 0
a = -3
Substituting the value of a in (i), we get,
-6 + b + 7 = 0
b = -1
Solution 12
Let the number k be added and the resulting polynomial be f(x).
So, f(x) = 3x3 - 5x2 + 6x + k
It is given that when f(x) is divided by (x - 3), the remainder is 8.
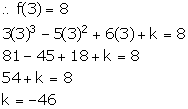
Thus, the required number is -46.
Solution 13
Let the number to be subtracted be k and the resulting polynomial be f(x).
So, f(x) = x3 + 3x2 - 8x + 14 - k
It is given that when f(x) is divided by (x - 2), the remainder is 10.
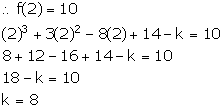
Thus, the required number is 8.
Solution 14
Let f(x) = 2x3 - 7x2 + ax - 6
x - 2 = 0 ![]() x = 2
x = 2
When f(x) is divided by (x - 2), remainder = f(2)
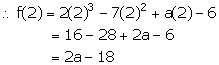
Let g(x) = x3 - 8x2 + (2a + 1)x - 16
When g(x) is divided by (x - 2), remainder = g(2)
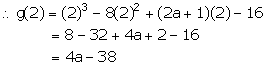
By the given condition, we have:
f(2) = g(2)
2a - 18 = 4a - 38
4a - 2a = 38 - 18
2a = 20
a = 10
Thus, the value of a is 10.
Solution 15
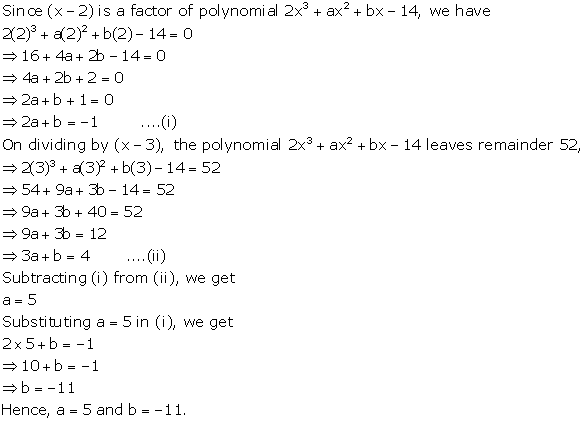
Remainder And Factor Theorems Exercise Ex. 8(B)
Solution 1(a)
Correct option: (iv) 5
The degree of the polynomial is 5.
Hence, the maximum number of linear factors is 5.
Solution 1(b)
Correct option: (ii) 16
f(x) = 3x + 8
f(–x) = 3(–x) + 8 = –3x + 8
f(x) + f(–x) = 3x + 8 + (–3x) + 8 = 16
Solution 1(c)
Correct option: (iii) 0
When x25 + x24 is divided by (x + 1),
Remainder = (–1)25 + (–1)24 = –1 + 1 = 0
Solution 1(d)
Correct option: (ii) x(x – 2)(3x + 4)
3x3 – 2x2 – 8x
= x(3x2 – 2x – 8)
= x(3x2 – 6x + 4x – 8)
= x[3x(x – 2) + 4(x – 2)]
= x[(x – 2)(3x + 4)]
= x(x – 2)(3x + 4)
Solution 1(e)
Correct option: (i) (2 + x)(2 – x)(1 + x)
4 + 4x – x2 – x3
= 4(1 + x) – x2(1 + x)
= (4 – x2)(1 + x)
= (2 + x)(2 – x)(1 + x)
Solution 2
(i) Let f(x) = x3 - 2x2 - 9x + 18
x - 2 = 0 ![]() x = 2
x = 2
![]() Remainder = f(2)
Remainder = f(2)
= (2)3 - 2(2)2 - 9(2) + 18
= 8 - 8 - 18 + 18
= 0
Hence, (x - 2) is a factor of f(x).
Now, we have:
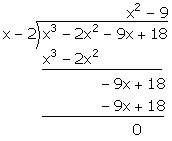
![]() x3 - 2x2 - 9x + 18 = (x - 2) (x2 - 9) = (x - 2) (x + 3) (x - 3)
x3 - 2x2 - 9x + 18 = (x - 2) (x2 - 9) = (x - 2) (x + 3) (x - 3)
(ii) Let f(x) = 2x3 + 5x2 - 28x - 15
x + 5 = 0 ![]() x = -5
x = -5
![]() Remainder = f(-5)
Remainder = f(-5)
= 2(-5)3 + 5(-5)2 - 28(-5) - 15
= -250 + 125 + 140 - 15
= -265 + 265
= 0
Hence, (x + 5) is a factor of f(x).
Now, we have:
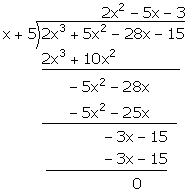
![]() 2x3 + 5x2 - 28x - 15 = (x + 5) (2x2 - 5x - 3)
2x3 + 5x2 - 28x - 15 = (x + 5) (2x2 - 5x - 3)
= (x + 5) [2x2 - 6x + x - 3]
= (x + 5) [2x(x - 3) + 1(x - 3)]
= (x + 5) (2x + 1) (x - 3)
Solution 3
(i)
(ii) Let f(x) = 2x3 + x2 - 13x + 6
For x = 2,
f(x) = f(2) = 2(2)3 + (2)2 - 13(2) + 6 = 16 + 4 - 26 + 6 = 0
Hence, (x - 2) is a factor of f(x).
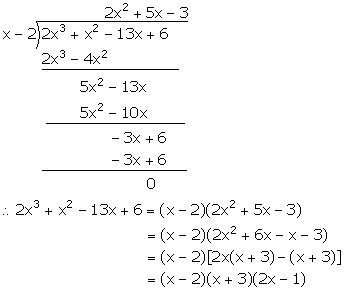
(iii) f(x) = 3x3 + 2x2 - 23x - 30
For x = -2,
f(x) = f(-2) = 3(-2)3 + 2(-2)2 - 23(-2) - 30
= -24 + 8 + 46 - 30 = -54 + 54 = 0
Hence, (x + 2) is a factor of f(x).
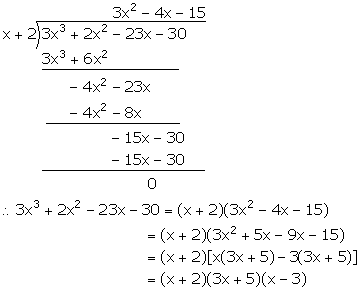
(iv) f(x) = 4x3 + 7x2 - 36x - 63
For x = 3,
f(x) = f(3) = 4(3)3 + 7(3)2 - 36(3) - 63
= 108 + 63 - 108 - 63 = 0
Hence, (x + 3) is a factor of f(x).
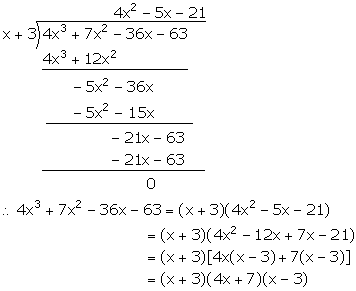
(v) f(x) = x3 + x2 - 4x - 4
For x = -1,
f(x) = f(-1) = (-1)3 + (-1)2 - 4(-1) - 4
= -1 + 1 + 4 - 4 = 0
Hence, (x + 1) is a factor of f(x).
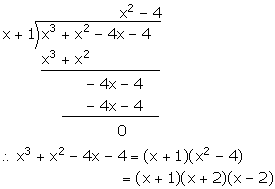
Solution 4
Let f(x) = 3x3 + 10x2 + x - 6
For x = -1,
f(x) = f(-1) = 3(-1)3 + 10(-1)2 + (-1) - 6 = -3 + 10 - 1 - 6 = 0
Hence, (x + 1) is a factor of f(x).
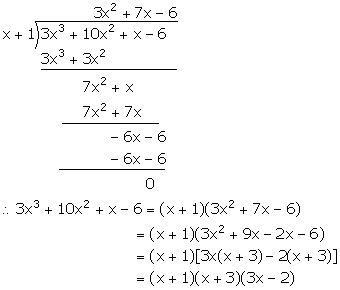
Now, 3x3 + 10x2 + x - 6 = 0
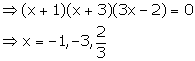
Solution 5
f (x) = 2x3 - 7x2 - 3x + 18
For x = 2,
f(x) = f(2) = 2(2)3 - 7(2)2 - 3(2) + 18
= 16 - 28 - 6 + 18 = 0
Hence, (x - 2) is a factor of f(x).
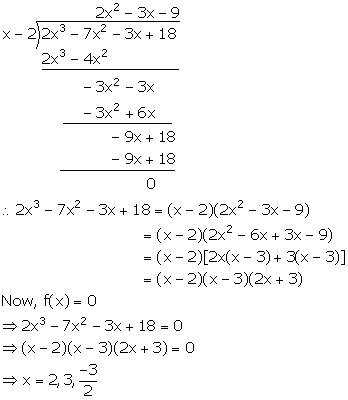
Solution 6
f(x) = x3 + 3x2 + ax + b
Since, (x - 2) is a factor of f(x), f(2) = 0
![]() (2)3 + 3(2)2 + a(2) + b = 0
(2)3 + 3(2)2 + a(2) + b = 0
![]() 8 + 12 + 2a + b = 0
8 + 12 + 2a + b = 0
![]() 2a + b + 20 = 0 ...(i)
2a + b + 20 = 0 ...(i)
Since, (x + 1) is a factor of f(x), f(-1) = 0
![]() (-1)3 + 3(-1)2 + a(-1) + b = 0
(-1)3 + 3(-1)2 + a(-1) + b = 0
![]() -1 + 3 - a + b = 0
-1 + 3 - a + b = 0
![]() -a + b + 2 = 0 ...(ii)
-a + b + 2 = 0 ...(ii)
Subtracting (ii) from (i), we get,
3a + 18 = 0
![]() a = -6
a = -6
Substituting the value of a in (ii), we get,
b = a - 2 = -6 - 2 = -8
![]() f(x) = x3 + 3x2 - 6x - 8
f(x) = x3 + 3x2 - 6x - 8
Now, for x = -1,
f(x) = f(-1) = (-1)3 + 3(-1)2 - 6(-1) - 8 = -1 + 3 + 6 - 8 = 0
Hence, (x + 1) is a factor of f(x).
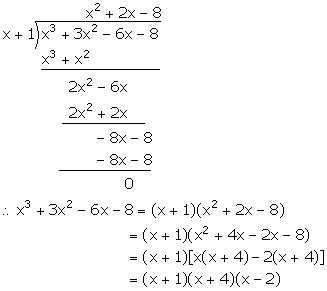
Solution 7
Let f(x) = 4x3 - bx2 + x - c
It is given that when f(x) is divided by (x + 1), the remainder is 0.
![]() f(-1) = 0
f(-1) = 0
4(-1)3 - b(-1)2 + (-1) - c = 0
-4 - b - 1 - c = 0
b + c + 5 = 0 ...(i)
It is given that when f(x) is divided by (2x - 3), the remainder is 30.
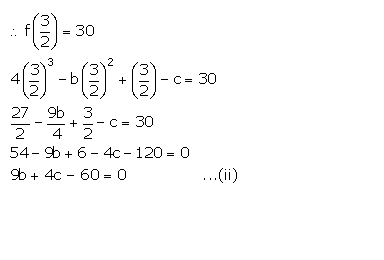
Multiplying (i) by 4 and subtracting it from (ii), we get,
5b + 40 = 0
b = -8
Substituting the value of b in (i), we get,
c = -5 + 8 = 3
Therefore, f(x) = 4x3 + 8x2 + x - 3
Now, for x = -1, we get,
f(x) = f(-1) = 4(-1)3 + 8(-1)2 + (-1) - 3 = -4 + 8 - 1 - 3 = 0
Hence, (x + 1) is a factor of f(x).
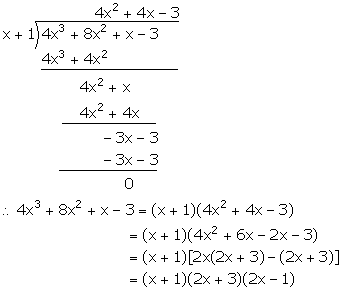
Solution 8
f(x) = x2 + px + q
It is given that (x + a) is a factor of f(x).
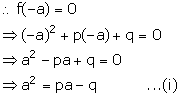
g(x) = x2 + mx + n
It is given that (x + a) is a factor of g(x).
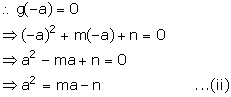
From (i) and (ii), we get,
pa - q = ma - n
n - q = a(m - p)
![]()
Hence, proved.
Remainder And Factor Theorems Exercise TEST YOURSELF
Solution 1(a)
Correct option: (ii) –4
x + 1 = 0 ⇒ x = –1
When x3 – x2 + x – 1 is divided by (x + 1),
Remainder = (–1)3 – (–1)2 + (–1) – 1
= –1 – 1 – 1 – 1
= –4
Solution 1(b)
Correct option: (iv) –8
x – 3 = 0 ⇒ x = 3
Since x – 3 is a factor of x2 + kx + 15,
Remainder = 0
⇒ (3)2 + k(3) + 15 = 0
⇒ 9 + 3k + 15 = 0
⇒ 3k = –24
⇒ k = –8
Solution 1(c)
Correct option: (i) yes
For (x – 2) to be a factor of x3 – 4x2 – 11x + 30, remainder = 0
Now,
(2)3 – 4(2)2 – 11(2) + 30
= 8 – 16 – 22 + 30
= 0
Then, (x – 2) is a factor of given polynomial.
Solution 1(d)
Correct option: (iii) 7
x – 1 = 0 ⇒ x = 1
Now, 4(1)2 – k(1) + 5 = 2
⇒ 4 – k + 5 = 2
⇒ 9 – k = 2
⇒ k = 7
Solution 1(e)
Correct option: (iv) n – 2m = 4
x – 2 = 0 ⇒ x = 2
Since x – 2 is a factor of mx2 – nx + 8,
Remainder = 0
⇒ m(2)2 – n(2) + 8 = 0
⇒ 4m – 2n + 8 = 0
⇒ 2m – n + 4 = 0
⇒ n – 2m = 4
Solution 2
Let f(x) = x3 + 3x2 - mx + 4
According to the given information,
f(2) = m + 3
(2)3 + 3(2)2 - m(2) + 4 = m + 3
8 + 12 - 2m + 4 = m + 3
24 - 3 = m + 2m
3m = 21
m = 7
Solution 3
Let the required number be k.
Let f(x) = 3x3 - 8x2 + 4x - 3 - k
According to the given information,
f (-2) = 0
3(-2)3 - 8(-2)2 + 4(-2) - 3 - k = 0
-24 - 32 - 8 - 3 - k = 0
-67 - k = 0
k = -67
Thus, the required number is -67.
Solution 4
Let f(x) = x3 + (a + 1)x2 - (b - 2)x - 6
Since, (x + 1) is a factor of f(x).
![]() Remainder = f(-1) = 0
Remainder = f(-1) = 0
(-1)3 + (a + 1)(-1)2 - (b - 2) (-1) - 6 = 0
-1 + (a + 1) + (b - 2) - 6 = 0
a + b - 8 = 0 ...(i)
Since, (x - 2) is a factor of f(x).
![]() Remainder = f(2) = 0
Remainder = f(2) = 0
(2)3 + (a + 1) (2)2 - (b - 2) (2) - 6 = 0
8 + 4a + 4 - 2b + 4 - 6 = 0
4a - 2b + 10 = 0
2a - b + 5 = 0 ...(ii)
Adding (i) and (ii), we get,
3a - 3 = 0
a = 1
Substituting the value of a in (i), we get,
1 + b - 8 = 0
b = 7
![]() f(x) = x3 + 2x2 - 5x - 6
f(x) = x3 + 2x2 - 5x - 6
Now, (x + 1) and (x - 2) are factors of f(x). Hence, (x + 1) (x - 2) = x2 - x - 2 is a factor of f(x).
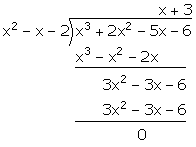
![]() f(x) = x3 + 2x2 - 5x - 6 = (x + 1) (x - 2) (x + 3)
f(x) = x3 + 2x2 - 5x - 6 = (x + 1) (x - 2) (x + 3)
Solution 5
Let f(x) = x2 + ax + b
Since, (x - 2) is a factor of f(x).
![]() Remainder = f(2) = 0
Remainder = f(2) = 0
(2)2 + a(2) + b = 0
4 + 2a + b = 0
2a + b = -4 ...(i)
It is given that:
a + b = 1 ...(ii)
Subtracting (ii) from (i), we get,
a = -5
Substituting the value of a in (ii), we get,
b = 1 - (-5) = 6
Solution 6
Let f(x) = mx3 + 2x2 - 3
g(x) = x2 - mx + 4
It is given that f(x) and g(x) leave the same remainder when divided by (x - 2). Therefore, we have:
f (2) = g (2)
m(2)3 + 2(2)2 - 3 = (2)2 - m(2) + 4
8m + 8 - 3 = 4 - 2m + 4
10m = 3
m = ![]()
Solution 7
Let f(x) = px3 + 4x2 - 3x + q
It is given that f(x) is completely divisible by (x2 - 1) = (x + 1)(x - 1).
Therefore, f(1) = 0 and f(-1) = 0
f(1) = p(1)3 + 4(1)2 - 3(1) + q = 0
p + q + 1 = 0 ...(i)
f(-1) = p(-1)3 + 4(-1)2 - 3(-1) + q = 0
-p + q + 7 = 0 ...(ii)
Adding (i) and (ii), we get,
2q + 8 = 0
q = -4
Substituting the value of q in (i), we get,
p = -q - 1 = 4 - 1 = 3
![]() f(x) = 3x3 + 4x2 - 3x - 4
f(x) = 3x3 + 4x2 - 3x - 4
Given that f(x) is completely divisible by (x2 - 1).
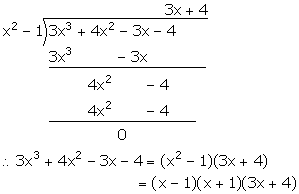
Solution 8
It is given that when the polynomial x3 + 2x2 - 5ax - 7 is divided by (x - 1), the remainder is A.
![]() (1)3 + 2(1)2 - 5a(1) - 7 = A
(1)3 + 2(1)2 - 5a(1) - 7 = A
1 + 2 - 5a - 7 = A
- 5a - 4 = A ...(i)
It is also given that when the polynomial x3 + ax2 - 12x + 16 is divided by (x + 2), the remainder is B.
![]() x3 + ax2 - 12x + 16 = B
x3 + ax2 - 12x + 16 = B
(-2)3 + a(-2)2 - 12(-2) + 16 = B
-8 + 4a + 24 + 16 = B
4a + 32 = B ...(ii)
It is also given that 2A + B = 0
Using (i) and (ii), we get,
2(-5a - 4) + 4a + 32 = 0
-10a - 8 + 4a + 32 = 0
-6a + 24 = 0
6a = 24
a = 4
Solution 9
Let f(x) = (a - 1)x3 + (a + 1)x2 - (2a + 1)x - 15
It is given that (3x + 5) is a factor of f(x).
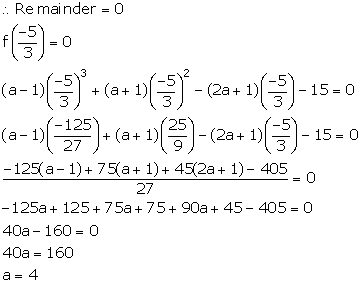
![]() f(x) = (a - 1)x3 + (a + 1)x2 - (2a + 1)x - 15
f(x) = (a - 1)x3 + (a + 1)x2 - (2a + 1)x - 15
= 3x3 + 5x2 - 9x - 15

Solution 10
Let f(x) = 2x3 + 3x2 - kx + 5
Using Remainder Theorem, we have
f(2) = 7
∴ 2(2)3 + 3(2)2 - k(2) + 5 = 7
∴ 16 + 12 - 2k + 5 = 7
∴ 33 - 2k = 7
∴ 2k = 26
∴ k = 13