Class 10 SELINA Solutions Maths Chapter 10 - Arithmetic Progression
Arithmetic Progression Exercise TEST YOURSELF
Solution 1(a)
Correct option: (iv) All are A.P.
A + B = (2 + 3), (4 + 6), (6 + 9), (8 + 12), …… = 5, 10, 15, 20, ….. is an A.P.
A – C = (2 – 0), (4 – 4), (6 – 8), (8 – 12), …. = 2, 0, –2, –4, …. is an A.P.
C – B = (0 – 3), (4 – 6), (8 – 9), (12 – 12), ….. = –3, –2, –1, 0, …. is an A.P.
B – A = (3 – 2), (6 – 4), (9 – 6), (12 – 8), ….. = 1, 2, 3, 4, …. is an A.P.
Solution 1(b)
Correct option: (iv) 5
2k – 7, k + 5 and 3k + 2 are in A.P.
(k + 5) – (2k – 7) = (3k + 2) – (k + 5)
12 – k = 2k – 3
3k = 15
k = 5
Solution 1(c)
Correct option: (ii) 5
l = a + (n – 1)d
36 = –36 + (n – 1)18
72 = (n – 1)18
n – 1 = 4
n = 5
Solution 1(d)
Correct option: (iii) Yes, 97
12, 52, 72, 73 …. = 1, 25, 49, 73 ….
This forms an A.P. with first term 1 and common difference 24.
Therefore, next term = 73 + 24 = 97
Solution 1(e)
Correct option: (ii) 110
First 10 even natural numbers = 2, 4, 6, 8, 10, 12, 14, 16, 18, 20
First term = 2
Common difference = 2

Options (i) and (iv) are same in textbook.
Solution 1(f)
Correct option: (i)
The statement given in assertion is correct.
Hence, assertion A is true.
The difference between the consecutive terms is same, i.e. ![]() .
.
Hence, reason (R) is true.
Solution 2
Let 'a' be the first term and 'd' be the common difference of the given A.P.
Now, t6 = 16 (given)
⇒ a + 5d = 16 ….(i)
And,
t14 = 32 (given)
⇒ a + 13d = 32 ….(ii)
Subtracting (i) from (ii), we get
8d = 16
⇒ d = 2
⇒ a + 5(2) = 16
⇒ a = 6
Hence, 36th term = t36 = a + 35d = 6 + 35(2) = 76
Solution 3
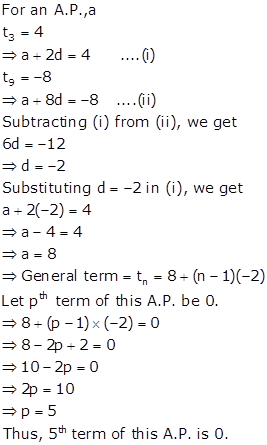
Solution 4
For a given A.P.,
Number of terms, n = 50
3rd term, t3 = 12
⇒ a + 2d = 12 ….(i)
Last term, l = 106
⇒ t50 = 106
⇒ a + 49d = 106 ….(ii)
Subtracting (i) from (ii), we get
47d = 94
⇒ d = 2
⇒ a + 2(2) = 12
⇒ a = 8
Hence, t29 = a + 28d = 8 + 28(2) = 8 + 56 = 64
Solution 5

Solution 6
Here,
First term, a = 3
Last term, l = 57
n = 20
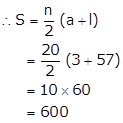
Solution 7
Here, we find that
15 - 18 = 12 - 15 = -3
Thus, the given series is an A.P. with first term 18 and common difference -3.
Let the number of term to be added be 'n'.
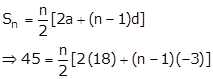
⇒ 90 = n[36 - 3n + 3]
⇒ 90 = n[39 - 3n]
⇒ 90 = 3n[13 - n]
⇒ 30 = 13n - n2
⇒ n2 - 13n + 30 = 0
⇒ n2 - 10n - 3n + 30 = 0
⇒ n(n - 10) - 3(n - 10) = 0
⇒ (n - 10)(n - 3) = 0
⇒ n - 10 = 0 or n - 3 = 0
⇒ n = 10 or n = 3
Thus, required number of term to be added is 3 or 10.
Solution 8
tn = 8 - 5n
Replacing n by (n + 1), we get
tn+1 = 8 - 5(n + 1) = 8 - 5n - 5 = 3 - 5n
Now,
tn+1 - tn = (3 - 5n) - (8 - 5n) = -5
Since, (tn+1 - tn) is independent of n and is therefore a constant.
Hence, the given sequence is an A.P.
Solution 9
The given sequence is 1, -1, -3, …..
Now,
1 - 3 = -1 - 1 = -3 - (-1) = -2
Hence, the given sequence is an A.P. with first term a = 3 and common difference d = -2.
The general term (nth term) of an A.P. is given by
tn = a + (n - 1)d
= 3 + (n - 1)(-2)
= 3 - 2n + 2
= 5 - 2n
Hence, 23rd term = t23 = 5 - 2(23) = 5 - 46 = -41
Solution 10
The given sequence is 11, 8, 5, 2, …..
Now,
8 - 11 = 5 - 8 = 2 - 5 = -3
Hence, the given sequence is an A.P. with first term a = 11 and common difference d = -3.
The general term of an A.P. is given by
tn = a + (n - 1)d
⇒ -150 = 11 + (n - 1)(-5)
⇒ -161 = -5n + 5
⇒ 5n = 166
![]()
The number of terms cannot be a fraction.
So, clearly, -150 is not a term of the given sequence.
Solution 11

Solution 12

Solution 13
Let 'a' be the first term and 'd' be the common difference of the given A.P.
Given,
S14 = 1050
![]()
⇒ 7[2a + 13d] = 1050
⇒ 2a + 13d = 150
⇒ a + 6.5d = 75 ….(i)
And, t14 = 140
⇒ a + 13d = 140 ….(ii)
Subtracting (i) from (ii), we get
6.5d = 65
⇒ d = 10
⇒ a + 13(10) = 140
⇒ a = 10
Thus, 20th term = t20 = 10 + 19d = 10 + 19(10) = 200
Solution 14
nth term of an A.P. is given by tn= a + (n - 1) d.
⇒ t25 = a + (25 - 1)d = a + 24d and
t9 = a + (9 - 1)d = a + 8d
According to the condition in the question, we get
t25 = t9 + 16
⇒ a + 24d = a + 8d + 16
⇒ 16d = 16
⇒ d = 1
Solution 15
Let a and d be the first term and common difference respectively.
⇒(m + n)th term = a + (m + n - 1)d …. (i) and
(m - n)th term = a + (m - n - 1)d …. (ii)
From (i) + (ii), we get
(m + n)th term + (m - n)th term
= a + (m + n - 1)d + a + (m - n - 1)d
= a + md + nd - d + a + md - nd - d
= 2a + 2md - 2d
= 2a + (m - 1)2d
= 2[ a + (m - 1)d]
= 2 × mthterm
Hence proved.
Solution 16
In the first A.P. 58, 60, 62,....
a = 58 and d = 2
tn = a + (n - 1)d
⇒ tn = 58 + (n - 1)2 …. (i)
In the first A.P. -2, 5, 12, ….
a = -2 and d = 7
tn = a + (n - 1)d
⇒ tn= -2 + (n - 1)7 …. (ii)
Given that the nth term of first A.P is equal to the nth term of the second A.P.
⇒58 + (n - 1)2 = -2 + (n - 1)7 … from (i) and (ii)
⇒58 + 2n - 2 = -2 + 7n - 7
⇒ 65 = 5n
⇒ n = 15
Solution 17
Here a = 105 and d = 101 - 105 = -4
Let an be the first negative term.
⇒ an < 0
⇒ a + (n - 1)d < 0
⇒ 105 + (n - 1)(-4)<0
⇒ 105 - 4n + 4 <0
⇒ 109 - 4n < 0
⇒ 109 <4n
⇒ 27.25 < n
The value of n = 28.
Therefore 28th term is the first negative term of the given A.P.
Solution 18
Let the three parts of 216 in A.P be (a - d), a, (a + d).
⇒a - d + a + a + d = 216
⇒ 3a = 216
⇒ a = 72
Given that the product of the two smaller parts is 5040.
⇒a(a - d ) = 5040
⇒ 72(72 - d) = 5040
⇒ 72 - d = 70
⇒ d = 2
∴ a - d = 72 - 2 = 70, a = 72 and a + d = 72 + 2 = 74
Therefore the three parts of 216 are 70, 72 and 74.
Solution 19
We have 2n2 - 7,
Substitute n = 1, 2, 3, … , we get
2(1)2 - 7, 2(2)2 - 7, 2(3)2 - 7, 2(4)2 - 7, ….
-5, 1, 11, ….
Difference between the first and second term = 1 - (-5) = 6
And Difference between the second and third term = 11 - 1 = 10
Here, the common difference is not same.
Therefore the nth term of an A.P can't be 2n2 - 7.
Solution 20
Here a = 14 , d = 7 and tn = 168
tn = a + (n - 1)d
⇒ 168 = 14 + (n - 1)7
⇒ 154 = 7n - 7
⇒ 154 = 7n - 7
⇒ 161 = 7n
⇒ n = 23
We know that,
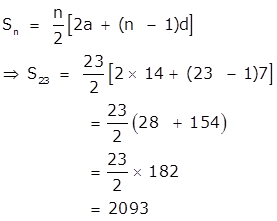
Therefore the sum of the A.P., 14, 21, 28, …, 168 is 2093.
Solution 21
Here a = 20 and S7 = 2100
We know that,
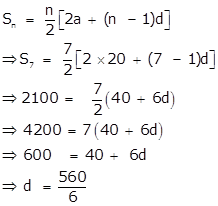
To find: t31 =?
tn = a + (n - 1)d
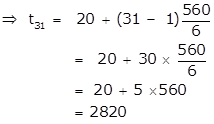
Therefore the 31st term of the given A.P. is 2820.
Solution 22
First we will reverse the given A.P. as we have to find the sum of last 8 terms of the A.P.
58, …., -8, -10, -12.
Here a = 58 , d = -2
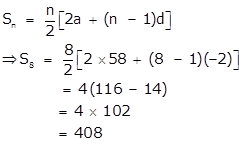
Therefore the sum of last 8 terms of the A.P. -12, -10, -8, ……, 58 is 408.
Arithmetic Progression Exercise Ex. 10(A)
Solution 2
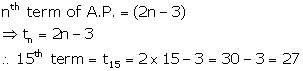
Solution 3

Solution 4

Solution 5

Solution 6

Solution 7

Solution 8

Solution 9
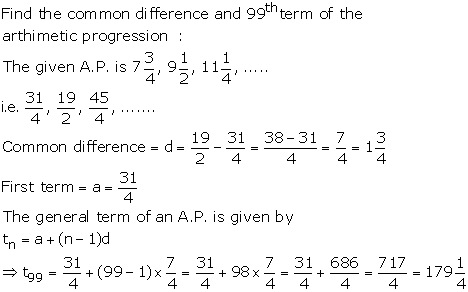
Solution 10

Solution 11
The given A.P. is 1, 4, 7, 10, ……….
Solution 12
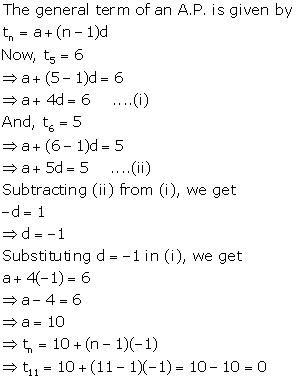
Solution 13

Solution 14

Solution 15

Solution 16
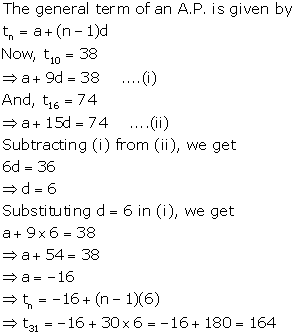
Solution 17

Solution 18
For a given A.P.,
Number of terms, n = 60
First term, a = 7
Last term, l = 125
⇒ t60 = 125
⇒ a + 59d = 125
⇒ 7 + 59d = 125
⇒ 59d = 118
⇒ d = 2
Hence, t31 = a + 30d = 7 + 30(2) = 7 + 60 = 67
Solution 19
Let 'a' be the first term and 'd' be the common difference of the given A.P.
t4 + t8 = 24 (given)
⇒ (a + 3d) + (a + 7d) = 24
⇒ 2a + 10d = 24
⇒ a + 5d = 12 ….(i)
And,
t6 + t10 = 34 (given)
⇒ (a + 5d) + (a + 9d) = 34
⇒ 2a + 14d = 34
⇒ a + 7d = 17 ….(ii)
Subtracting (i) from (ii), we get
2d = 5

Solution 20
Let 'a' be the first term and 'd' be the common difference of the given A.P.
Now, t3 = 5 (given)
⇒ a + 2d = 5 ….(i)
And,
t7 = 9 (given)
⇒ a + 6d = 9 ….(ii)
Subtracting (i) from (ii), we get
4d = 4
⇒ d = 1
⇒ a + 2(1) = 5
⇒ a = 3
Hence, 17th term = t17 = a + 16d = 3 + 16(1) = 19
Solution 1(a)
Correct option: (ii) 8, 3, –2, –7, …..
First term = a = 8
Common difference = d = –5
Then, the A.P. is 8, 8 + (–5), 8 + 2(–5), 8 + 3(–5), ……
That is, 8, 3, –2, –7, …..
Solution 1(b)
Correct option: (ii) yes
The given sequence –8, –8, –8, –8, ….. is an A.P. with the first term –8 and common difference zero.
Solution 1(c)
Correct option: (iv) –39
Given A.P. is 3, 0, –3, –6, …..
First term, a = 3
Common difference, d = 0 – 3 = –3
15th term, t15 = a + 14d
= 3 + 14(–3)
= 3 – 42
= –39
Solution 1(d)
Correct option: (ii) 2
t24 – t19 = 10
(a + 23d) – (a + 18d) = 10
5d = 10
d = 2
Solution 1(e)
Correct option: (ii) 16
Given A.P. is 8, 13, 18, …..
First term, a = 8
Common difference, d = 13 – 8 = 5
nth term, tn = 83
a + (n – 1)d = 83
8 + (n – 1)5 = 83
(n – 1)5 = 75
n – 1 = 15
n = 16
Arithmetic Progression Exercise Ex. 10(B)
Solution 2

Solution 3

Solution 4

Solution 5
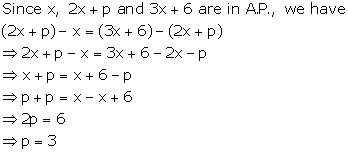
Solution 6

Solution 7

Solution 8
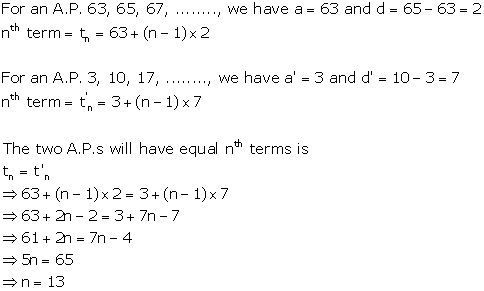
Solution 9

Solution 10
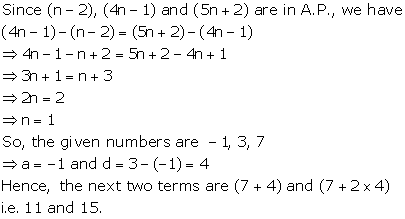
Solution 11
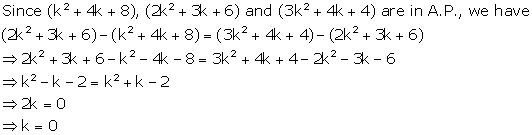
Solution 12

Solution 13
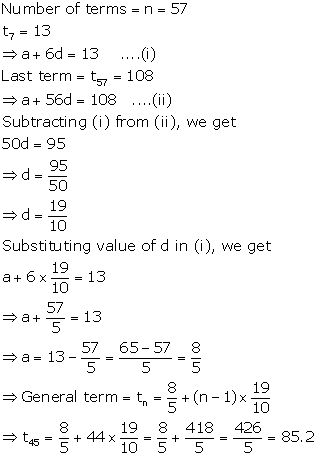
Solution 14

Solution 15

Solution 16
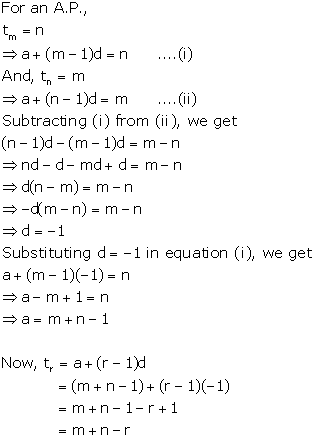
Solution 17

Solution 1(a)
Correct option: (iii) 8
Common difference of two A.P.,s = d
Let the first and nth terms of the first A.P. be a and tn, respectively.
Let the first and nth terms of the second A.P. be A and Tn, respectively.
Then, t25 – T25 = 8
a + 24d – A – 24d = 8
a – A = 8
Now, t50 – T50 = a + 49d – A – 49d = a – A = 8
Solution 1(b)
Correct option: (i) 0
Let the first term and the common difference of an A.P. be a and d, respectively.
Then, 10 × t10 = 20 × (t20)
10 × (a + 9d) = 20 × (a + 19d)
a + 9d = 2(a + 19d)
a + 9d = 2a + 38d
a + 29d = 0
t30 = 0
Solution 1(c)
Correct option: (iv) 7
tn = 7n – 5
Then, t1 = 7(1) – 5 = 2 and t2 = 7(2) – 5 = 9
Therefore, common difference = t2 – t1 = 9 – 2 = 7
Solution 1(d)
Correct option: (iv) 3
t40 – t16 = 72
(a + 39d) – (a + 15d) = 72
24d = 72
d = 3
Solution 1(e)
Correct option: (i) 17
First term = a = 6
Common difference = d = 11 – 6 = 5
tn = 106
a + (n – 1)d = 106
6 + (n – 1)(5) = 106
(n – 1)(5) = 100
n – 1 = 20
n – 1 – 3 = 17
n – 4 = 17
Arithmetic Progression Exercise Ex. 10(C)
Solution 2
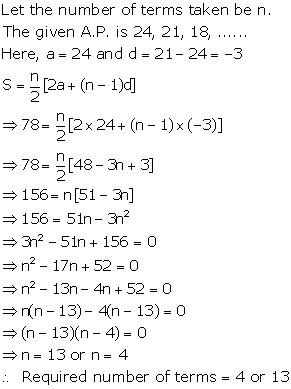
Solution 3
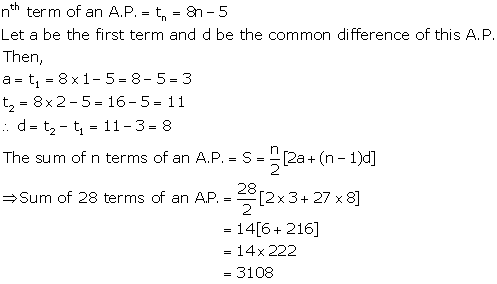
Solution 4(i)

Solution 4(ii)

Solution 5

Solution 6

Solution 7

Solution 8

Solution 9

Solution 10
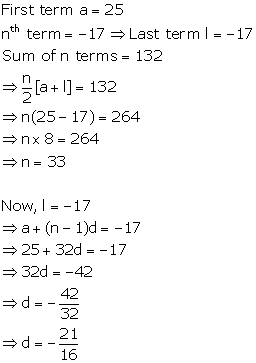
Solution 11

Solution 12
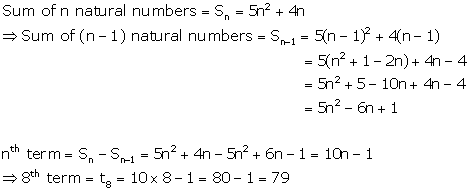
Solution 13

Solution 1(a)
Correct option: (ii) 1640
Total terms = 41
Then, middle term = 21st term = 40
That is, a + 20d = 40
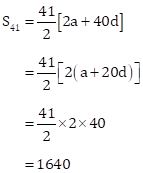
Solution 1(b)
Correct option: (iv) 4905
Two digit numbers are 10, 11, 12, ……99
This forms an A.P. with first term, a = 10, last term, l = 99 and common difference, d = 1
Hence, n = 100 – 10 = 90
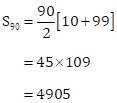
Solution 1(c)
Correct option: (i) 650
First term, a = 4
Common difference, d = 7 – 4 = 3
n = 20
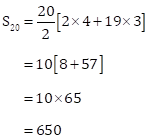
Solution 1(d)
Correct option: (ii) 2620
First term, a = 7
Common difference, d = 10 – 7 = 3
n = 40
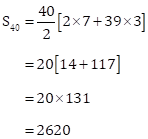
Solution 1(e)
Correct option: (iii) 26
tn = 6n + 4
Then, t1 = 6(1) + 4 = 10 and t2 = 6(2) + 4 = 16
Sum of first two terms = t1 + t2 = 10 + 16 = 26
Arithmetic Progression Exercise Ex. 10(D)
Solution 2
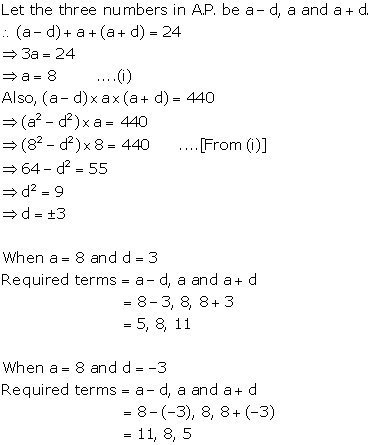
Solution 3

Solution 4

Solution 5
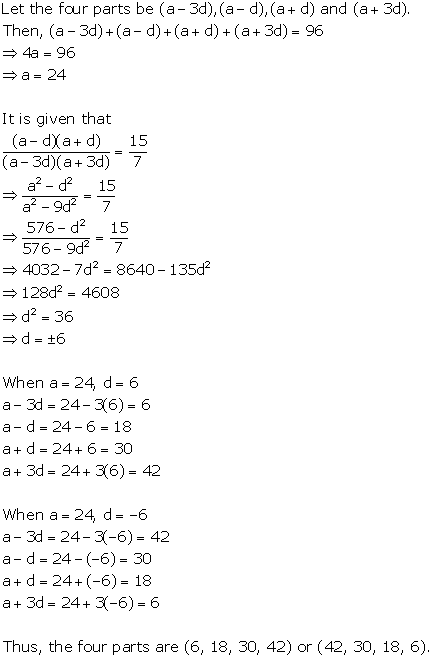
Solution 6
We know that,
Sum of n terms of an A.P = ![]()
Let the first term be 2x and the last term be 3x.
∴ Sum of 5 terms of an A.P = ![]()
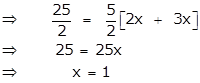
First term = 2x =2 × 1 = 2 and the last term = 3x = 3 × 1 = 3
nth term of an A.P. is given by
tn = a + (n - 1)d
⇒ a5 = 2 + (5 - 1)d
⇒ 3 = 2 + 4d
⇒ 1 = 4d
⇒ d = ![]() = 0.25
= 0.25
Therefore the five numbers in an A.P are 2, 2.25, 2.50, 2.75 and 3.
Solution 7

Solution 8
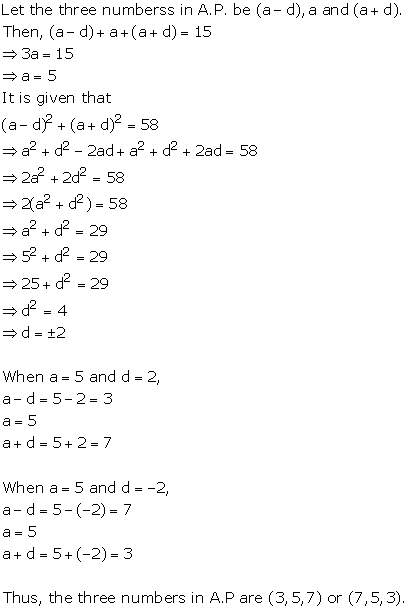
Solution 9
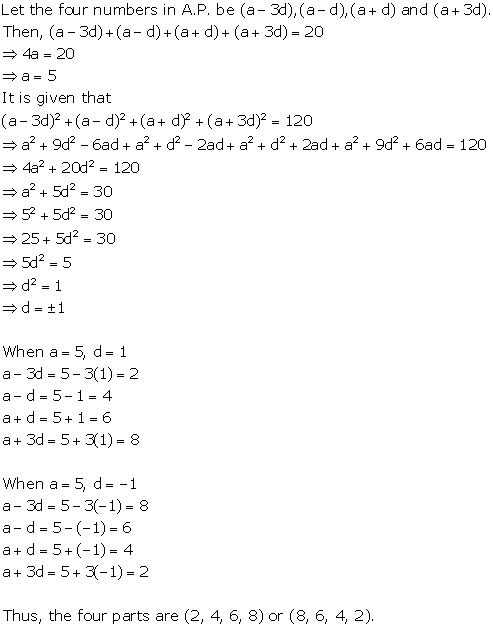
Solution 10
![]()
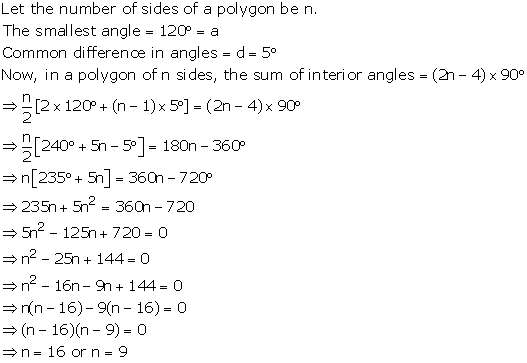
Solution 11
Solution 12
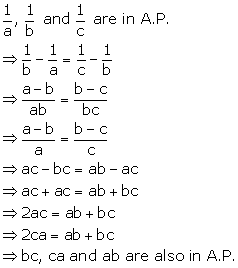
Solution 1(a)
t1 = k + 2, t2 = 2k + 7 and t3 = 4k + 12
t2 – t1 = t3 – t2
(2k + 7) – (k + 2) = (4k + 12) – (2k + 7)
k + 5 = 2k + 5
k = 0
Therefore, first term = 0 + 2 = 2
Solution 1(b)
Correct option: (iii) 9
Sn = 3n2
S1 = 3(1)2 = 3
S2 = 3(2)2 = 12
Second term = S2 – S1= 12 – 3 = 9
Solution 1(c)
Correct option: (ii) 5 × 7, 7 × 7 and 9 × 7
5, 7 and 9 are in A.P.
If each term of an A.P. is multiplied by a non-zero fixed number, the resulting sequence is an A.P.


