NEET Class neet Answered
Which of the following functions represent wave ?
Plzz Explain each option by differential equation of wave...
→d²y/dx²=1/v²(d²y/dt²)

Asked by jhajuhi19 | 05 Mar, 2020, 13:28: PM
if a function y(x,t) is solution to the differential equation of wave motion , 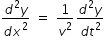 ..........................(1)
..........................(1)
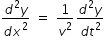 ..........................(1)
..........................(1)where v is speed of wave, then y(x,t) represents wave .
(a) y(x,t) = sin(kx) cos(ωt )
we find, 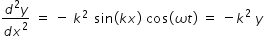 ...........................(2)
...........................(2)
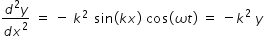 ...........................(2)
...........................(2)we find 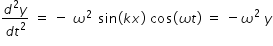 .......................(3)
.......................(3)
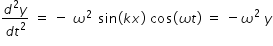 .......................(3)
.......................(3)In above equations ω is angular frequency and k is wave vector .
Speed of the wave v is related to angular frequency ω and wave vector k is , v = ω/k ..................(4)
from eqns.(2) , (3) and (4), we verify the function y(x,t) = sin(kx) cos(ωt) is solution to the differential equation
of wave motion as given by eqn.(1).
hence the function y(x,t) represents wave
---------------------------------------------------------------------------
(b) y(x,t) = k2 x2 - ω2 t2
we find , 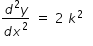 and
and 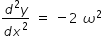
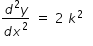 and
and 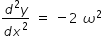
from the above relations we find y(x,t) = k2 x2 - ω2 t2 is not the solution of differential equation (1)
Hence y(x,t) = k2 x2 - ω2 t2 do not represent wave
-------------------------------------------------------
similarly we can verify for other functions
Answered by Thiyagarajan K | 05 Mar, 2020, 19:24: PM
Application Videos
Concept Videos
NEET neet - Physics
Asked by deepakkumawat7886 | 30 Jan, 2025, 13:59: PM
NEET neet - Physics
Asked by sayrapatelsp09 | 04 Dec, 2024, 20:32: PM
NEET neet - Physics
Asked by jhansikethburugu2008 | 04 Oct, 2024, 22:09: PM
NEET neet - Physics
Asked by arfasamreen7766 | 13 Sep, 2024, 16:03: PM
NEET neet - Physics
Asked by mehekfz8738 | 02 Sep, 2024, 19:56: PM
NEET neet - Physics
Asked by muhammadifatimanoori | 22 Aug, 2024, 09:10: AM
NEET neet - Physics
Asked by kumawatranya0812 | 16 Aug, 2024, 16:25: PM
NEET neet - Physics
Asked by nzf1042692 | 10 Aug, 2024, 21:07: PM
NEET neet - Physics
Asked by dropper744 | 08 Aug, 2024, 10:44: AM
NEET neet - Physics
Asked by sajnadevirrc | 06 Aug, 2024, 11:55: AM






