Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

What is the centre of mass of sector of the circle. Explain with diagram
Asked by rushabh1234 | 17 Oct, 2019, 06:05: AM
answered-by-expert Expert Answer
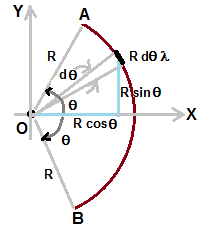
First let us find the centre of mass of thin rod bent in the form of circular arc that subtends angle 2θ at centre.
 
Let us cinsider a small element of length dl that subtends angle dθ at centre.
Let mass is distributed uniformly and λ is mass per unit length of rod .
 
mass moment of this small element along x-axis :-  ( R dθ λ ) ( R cosθ) = R2 λ cosθ dθ
 
mass moment of this small element along y-axis :-  ( R dθ λ ) ( R sinθ) = R2 λ sinθ dθ
 
x-coordinate of centre of mass of ring :- begin mathsize 14px style fraction numerator R squared lambda over denominator M end fraction integral subscript negative theta end subscript superscript theta cos theta apostrophe space d theta apostrophe space equals space 2 fraction numerator R squared lambda over denominator M end fraction sin theta end style ........................... (1)
y-coordinate of centre of mass of ring :- begin mathsize 14px style fraction numerator R squared lambda over denominator M end fraction integral subscript negative theta end subscript superscript theta sin theta apostrophe space d theta apostrophe space equals space 0 end style   ..............................(2)
Where M is mass of whole rod.
 
Mass density λ is defined as, λ =  mass / length = M/( R 2θ )  ..................(3)
 
using the expression for λ in (3), we get x-coordinate and y-coordinate of centre of mass from eqn.(1) and (2) as
 
Centre of mass :-  [ ( R sinθ / θ ) , 0 ] .................................(4)
 
Coordinates are given in above equation, as shown in figure, considering centre of curvature of arc coincides with origin and
line connecting the origin and centre of circular arc coincides with x-axis.
-------------------------------------------------------------------
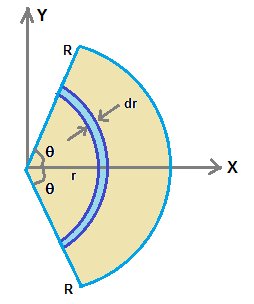
We consider sector of circular disc , angle 2θ at centre , is made up sevaral rings in the shpae of circular arc as shown in figure.
 
Since we calculated coordinates of centre of mass of circular arc, using that formula  for a circular arc ring of thickness dr,
we find the x-coordinate of centre of mass of sector of circular disc as follows
 
mass of circular arc ring = r×2θ×dr×ρ
 
where ρ is density per unit area
 
If M is total mass of sector of circular disc, then x-coordinate of centre of mass of sector of circular disc is given as
 
begin mathsize 14px style 1 over M integral subscript 0 superscript R r space cross times 2 theta cross times d r cross times rho cross times r cross times fraction numerator sin theta over denominator theta end fraction space equals space fraction numerator 2 space sin theta space rho over denominator M end fraction integral subscript 0 superscript R r squared d r space equals space 2 over 3 fraction numerator rho space R cubed over denominator M end fraction sin theta end style...............(5)
density ρ is defined as, ρ = M/ [ (2θ/2π) πR2 ] = M / [ θ R2 ] ...............(6)
 
By substituting ρ in eqn.(5),  x-coordinate of centre of mass of sector of circular disc is given as   (2/3)R (sinθ/θ)
 
x and y coordinate of centre of mass of sector of circular disc is given as  [ (2/3)R (sinθ/θ) , 0 ]
 
Coordinates are given in above equation, as shown in figure, considering centre of curvature of sector of circle coincides with origin and
midline dividing the sector of circle coincides with x-axis.
 
Answered by Thiyagarajan K | 17 Oct, 2019, 22:23: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt