Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

What is the centre of mass of pyramid? Explain briefly with examples
Asked by polyrelation | 21 Oct, 2019, 22:11: PM
answered-by-expert Expert Answer
A regular pyramid has square base and triangular faces as lateral sides. 
Let us have x-y-z coordinate system with origin coincide with centre of square base.
let x-y plane is parallel to square base and z-axis coincides with vertical axis of pyramid.
 
centre of mass of 3-dimensional solid is given as
 
begin mathsize 14px style z subscript c m space end subscript equals space fraction numerator integral subscript z m i n end subscript superscript z m a x end superscript integral subscript y m i n end subscript superscript y m a x end superscript integral subscript x m i n end subscript superscript x m a x end superscript z d x d y d z over denominator integral subscript z m i n end subscript superscript z m a x end superscript integral subscript y m i n end subscript superscript y m a x end superscript integral subscript x m i n end subscript superscript x m a x end superscript d x d y d z end fraction end style
where zmin and zmax are lower and upper limits of integration. For our pyramid, zmin = 0, zmax = h.
 
ymax and ymin are y-limits.   xmax and xmin are x-limits.
 
x and y limits are obtained from the sides of square cross section at a given height as shown below
 
 
 
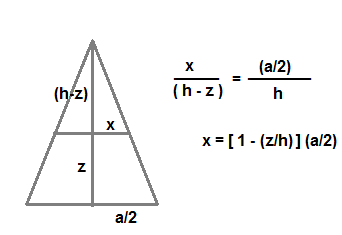
centre of mass of pyramid is obtained  as begin mathsize 14px style z subscript c m space end subscript equals space fraction numerator integral subscript 0 superscript h integral subscript negative left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end subscript superscript left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end superscript integral subscript negative left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end subscript superscript left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end superscript z d x d y d z over denominator integral subscript 0 superscript h integral subscript negative left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end subscript superscript left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end superscript integral subscript negative left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end subscript superscript left square bracket 1 minus left parenthesis z divided by h right parenthesis right square bracket left parenthesis a divided by 2 right parenthesis end superscript d x d y d z end fraction end style
from the above integration, we get zCM = h/4
 
Hence centre of mass of regular pyramid is at axis and at a height h/4 from base, where h is height of pyramid
Answered by Thiyagarajan K | 22 Oct, 2019, 14:31: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt