Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

Urgent... Plz plz solve this numerical attached below. 
question image
Asked by subhrajayanta64 | 25 Aug, 2019, 03:28: AM
answered-by-expert Expert Answer
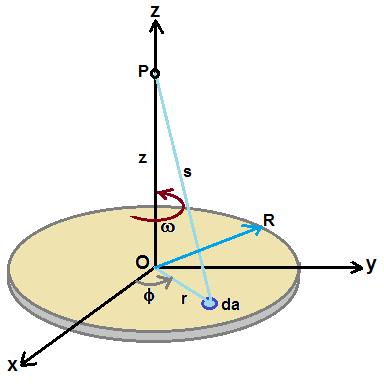
Figure shows a uniformly charged disc of radius R  with uniform charge density σ per unit area.
Let the axis of disc coincide with z-axis of cartesian coordinate system and centre of disc is at origin.
 
If the disc is roatating with angular velocity ω, then we get current flow in circular direction due to
rotational motion of charges in the disc. This creates magnetic field in surrounding region
 
Magnetic vector potential A at a point P on the axis which is at a distance z due to small area element da is given by
 
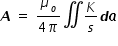begin mathsize 14px style bold italic A space equals space fraction numerator mu subscript o over denominator 4 pi end fraction double integral K over s bold italic d bold italic a end style ...............................(1)
where K is current density vector, K = σ ω r  and area element da = dr rdφ = r dr dφ   
 
By using these substitutions, eqn.(1) is written as 
 
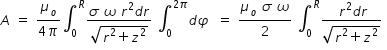begin mathsize 14px style A space equals space fraction numerator mu subscript o over denominator 4 pi end fraction integral subscript 0 superscript R fraction numerator sigma space omega space r squared d r over denominator square root of r squared plus z squared end root end fraction space integral subscript 0 superscript 2 pi end superscript d phi space space equals space fraction numerator mu subscript o space sigma space omega over denominator 2 end fraction space integral subscript 0 superscript R fraction numerator space r squared d r over denominator square root of r squared plus z squared end root end fraction space end style  ......................(2)
 
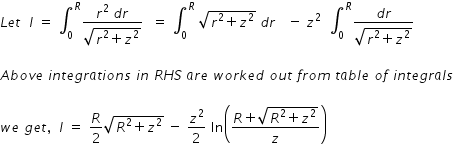
begin mathsize 14px style L e t space space I space equals space integral subscript 0 superscript R fraction numerator r squared space d r over denominator square root of r squared plus z squared end root end fraction space space equals space integral subscript 0 superscript R space square root of r squared plus z squared end root space d r space space space minus space z squared space space integral subscript 0 superscript R fraction numerator d r over denominator square root of r squared plus z squared end root end fraction A b o v e space i n t e g r a t i o n s space i n space R H S space a r e space w o r k e d space o u t space f r o m space t a b l e space o f space i n t e g r a l s w e space g e t comma space space I space equals space R over 2 square root of R squared plus z squared end root space minus space z squared over 2 space ln open parentheses fraction numerator R plus square root of R squared plus z squared end root over denominator z end fraction close parentheses end style
 
By substituting the above integration results in eqn.(2), we get Vector potential A as
 
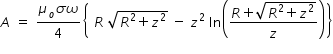
begin mathsize 14px style A space equals space fraction numerator mu subscript o sigma omega over denominator 4 end fraction open curly brackets space R space square root of R squared plus z squared end root space minus space z squared space ln open parentheses fraction numerator R plus square root of R squared plus z squared end root over denominator z end fraction close parentheses close curly brackets end style
Direction of vector potential is along +ve z-axis , as per eqn.(1)  [  da is vector area , direction of da is along z-axis ]
Answered by Thiyagarajan K | 25 Aug, 2019, 16:25: PM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
What is circular motion
Asked by jwjwwj | 16 Jan, 2025, 13:26: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is difference between drift and jerk
Asked by mishradarshan03 | 22 Dec, 2024, 11:45: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt