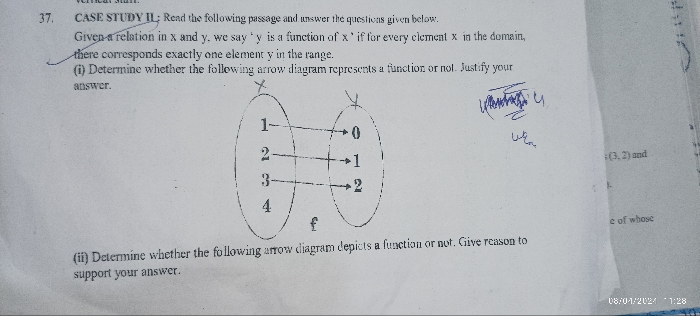
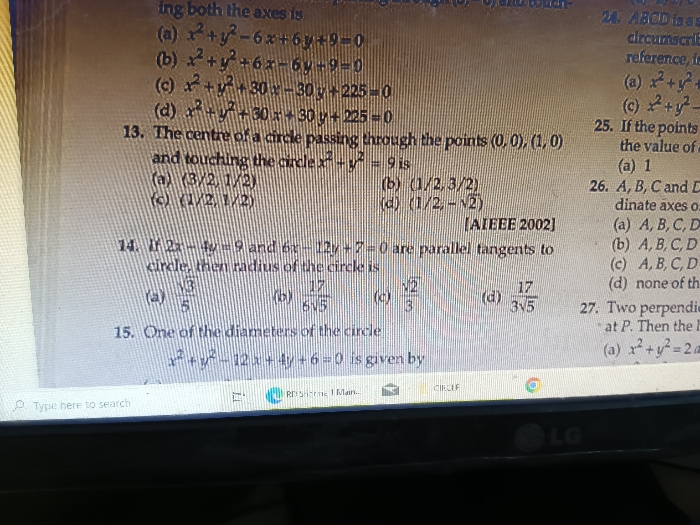
CBSE Class 12-science Answered

Since the we are talking about the triangle inscribed in a GIVEN CIRCLE, the radius r is constant.

A(x) = (r+x)(r²-x²)^1/2 = uv where u = (r+x), v = (r²-x²)^1/2

dA(x)/dx = d(uv)/dx = udv/dx + vdu/dx ..................using the product rule.

du/dx = 1, and dv/dx = (1/2)(r²-x²)^-1/2(-2x)....................using the differentiation rules..chain rule.

Substituting these, the first derivative is,

dA(x)/dx = (r+x)(1/2)(r²-x²)^-1/2(-2x) + (r²-x²)^1/2

dA(x)/dx = (r+x)(r²-x²)^-1/2(-x) + (r²-x²)^1/2 = U/v + v .....where U = -x(r+x)

Similarly the second derivative can be evaluated, using the quotient rule of differentiation,

d²A(x)/dx² = (vdU/dx - Udv/dx)/v²  + dv/dx

Now to find the value of x that maximizes the area, we set first derivative equal to zero,

(r+x)(r²-x²)^-1/2 (-x) + (r²-x²)^1/2 = 0 

rx + x² - r² + x² = 0,

x = (- r ± 3r)/4, x = r/2,

So the base is r+x =3r/2, so we can similarly draw perpendicular to another side, and following same arguments, we find it's also 3r/2 and again for the third.

It shows that it's an equilateral triangle.

Regards,

Team,

TopperLearning.