Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

If a heavy spring of uniform density and mass m is hung vertically so that it is stretched by gravity under its own weight, where is the center of mass?
Asked by rushabhjain.av | 20 Nov, 2019, 22:00: PM
answered-by-expert Expert Answer
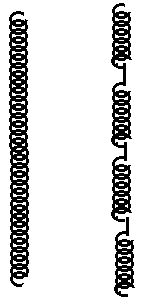
A heavy spring of mass M and length L can be considered as N number of springs connected in series,
each having length l and amss m so that
 
M = N × m   .....................(1)
 
L = N × l .....................(2)
 
If each small spring has spring constant k, then the spring constant of heavy spring is K , so that K = k/N .
 
when the heavy spring is suspended vertically, extension of the spring due to gravity and its own mass is different for lower and upper parts of spring.
 
Upper part of spring due to heavy mass haging down below it , has more extension than the Lower part of spring.
 
Let us consider the extension of individual small spring :-  F = m'g = k Δl'  ...............(3)
 
where m' is the mass hanging below the spring and Δl' is the extension
 
Let Δl be the extension of each spring due to its own mass m.
 
Then we have , mg = k Δl  or  Δl = (mg)/k   .........................(4)
 
Let us consider spring-1 is the top most small spring, spring-2 is next spring below it , so on.
Each spring's extension depends on the total mass of springs hanging below.
Let us consider the distance of centre of mass of each spring from top most hanging point.
 
Extended length of each small spring and distance of centre of mass of each extended spring is tabulated and it is given below
 
Spring No. n Extended Length
Distance yn of centre of mass
of spring
1 l + NΔl (1/2) [ l + N Δl ]
2 l + (N-1)Δl [ l + N Δl ] + [ l + (N-1)Δl ] /2 = (3/2) [ l + N Δl ] - (Δl/2)  
3 l + (N-2)Δl [ l + N Δl ] + [ l + (N-1)Δl ] + [ l + (N-2)Δl ] /2 = (5/2) [ l + N Δl ] - 2(Δl/2)
4 l + (N-3)Δl l + N Δl ] + [ l + (N-1)Δl ] + [ l + (N-2)Δl ] + [ l + (N-3)Δl ] /2 = (7/2) [ l + N Δl ] - 3(Δl/2)
-------- ------------- --------------
-------------- ------------- ----------------
-------------- ---------------- ------------------
N-1 l + 2 Δl  ( (2N-3)/2 ) [ l + N Δl ] - (N-2)( Δl / 2 )
N l + Δl ( (2N-1)/2 ) [ l + N Δl ] - (N-1)( Δl / 2 )
Let Y be the cenetre of mass when the heavy spring is suspended vertically and it extends due to its own mass.
 
Then by defineition of centre of mass,  
Above summation is wroked out using values of tabulated yn as
 
M × Y = m N2 [ l + N Δl ]/2 + m [ (N-1)(N)/2 ] ( Δl / 2 )
 
when N -> ∞ ,  N(N-1) ≈ N2
 
Hence M × Y = m N2 [ (l/2) + ( N + (1/2)) Δl ]
 
since M = m × N , we get , Y = N [ (l/2) + ( N + (1/2)) (Δl/2) ]
 
we use N × l = L , where L is length of spring in the above equation.
 
Also we use the approximation when N -> ∞ ,  N [ N +(1/2) ] ≈ N2
 
Y = (L/2) + N2(Δl/2)  = (L/2) + N2 (mg)/(2k) = (L/2) + (M g )/ ( 2 K )
 
( We have used, ( N m ) = M and  (k/N) = K in the above equation )
Answered by Thiyagarajan K | 26 Nov, 2019, 11:24: AM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Physics
a marble block of mass 2 kg lying on ice when a given velocity of 6 ms–1 is stopped by friction in 10 seconds then co efficient of friction is
Asked by sumalathamadarapu9 | 23 Oct, 2024, 22:06: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
show that the frequency of oscillations remain unchanged whether a mass attached to a spring is made to oscillate in the vertical or in the horizontal direction
Asked by py309649 | 13 Oct, 2024, 13:39: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
Square of the time period of revolution of a planet is proportional to the cube of the mean distance between sun and planet. If mean distance is increased by 2% then percentage change in its time period is
Asked by coolskrish | 13 Oct, 2024, 12:50: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two particles of mass m and 4m are initially actress the sad moving towards each other due to mutual gravitational force at an instantly of m is vî then at this instant the velocity of mass 4m is
Asked by midnightmoon3355 | 09 Oct, 2024, 09:09: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the second law of thermodynamics
Asked by rambabunaidu4455 | 03 Oct, 2024, 16:03: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
what is the relation between drift velocity and mobility
Asked by ratchanavalli07 | 17 Sep, 2024, 07:46: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
A ball of mass m approaches a moving wall of infinite mass with speed v along the normal to the wall. The speed of the ball is u towards the ball. The speed of the ball after elastic collision with the wall is
Asked by yayashvadutta45 | 15 Sep, 2024, 19:47: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
a current of 2a is flowing through a conductor of length 22m bent in arc radius 5cm
Asked by adithireddy999 | 03 Sep, 2024, 09:35: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
two vectors vector a and vector B lie in one plane vector c lie in the different plane then vector a + vector b + vector c is
Asked by vaishalinirmal739 | 29 Aug, 2024, 18:07: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Physics
What is impulse?
Asked by vradhysyam | 26 Aug, 2024, 17:17: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt