Ask a Doubt
Request a call back

Join NOW to get access to exclusive study material for best results

JEE Class main Answered

Find the value of a and b such that the function is continuous at x=0. f(x) =  x+sin x/ sin((a+1)x)     if -π0  
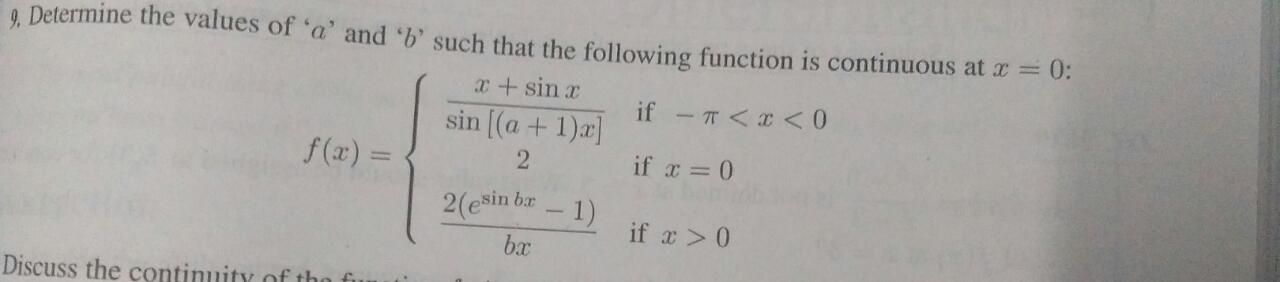
question image
Asked by saikatsukai514 | 22 Apr, 2020, 21:49: PM
answered-by-expert Expert Answer
f(x) is continuous at x = 0
Therefore, LHL = RHL = f(0)
LHL = f(0)
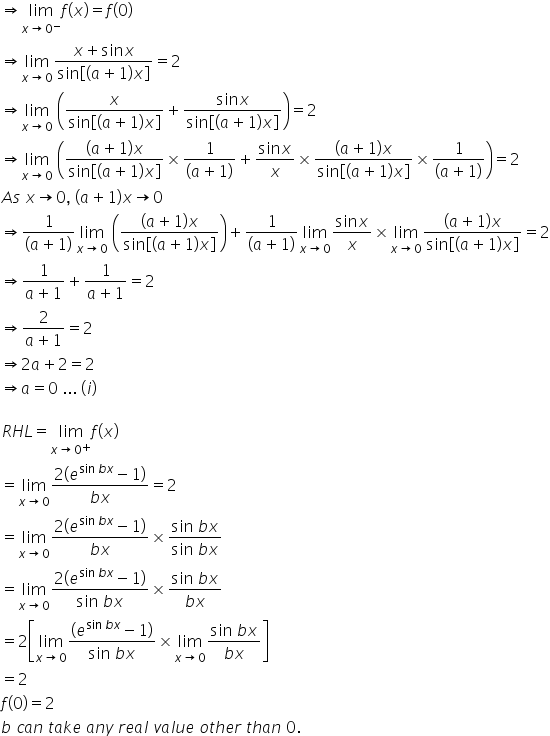
rightwards double arrow limit as x rightwards arrow 0 to the power of minus of f left parenthesis x right parenthesis equals f left parenthesis 0 right parenthesis rightwards double arrow limit as x rightwards arrow 0 of fraction numerator x plus sin x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction equals 2 rightwards double arrow limit as x rightwards arrow 0 of space open parentheses fraction numerator x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction plus fraction numerator sin x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction close parentheses equals 2 rightwards double arrow limit as x rightwards arrow 0 of space open parentheses fraction numerator left parenthesis a plus 1 right parenthesis x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction cross times fraction numerator 1 over denominator left parenthesis a plus 1 right parenthesis end fraction plus fraction numerator sin x over denominator x end fraction cross times fraction numerator left parenthesis a plus 1 right parenthesis x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction cross times fraction numerator 1 over denominator left parenthesis a plus 1 right parenthesis end fraction close parentheses equals 2 A s space x rightwards arrow 0 comma space left parenthesis a plus 1 right parenthesis x rightwards arrow 0 rightwards double arrow fraction numerator 1 over denominator left parenthesis a plus 1 right parenthesis end fraction limit as x rightwards arrow 0 of space open parentheses fraction numerator left parenthesis a plus 1 right parenthesis x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction close parentheses plus fraction numerator 1 over denominator left parenthesis a plus 1 right parenthesis end fraction limit as x rightwards arrow 0 of fraction numerator sin x over denominator x end fraction cross times limit as x rightwards arrow 0 of fraction numerator left parenthesis a plus 1 right parenthesis x over denominator sin open square brackets open parentheses a plus 1 close parentheses x close square brackets end fraction equals 2 rightwards double arrow fraction numerator 1 over denominator a plus 1 end fraction plus fraction numerator 1 over denominator a plus 1 end fraction equals 2 rightwards double arrow fraction numerator 2 over denominator a plus 1 end fraction equals 2 rightwards double arrow 2 a plus 2 equals 2 rightwards double arrow a equals 0 space... space left parenthesis i right parenthesis R H L equals limit as x rightwards arrow 0 to the power of plus of f left parenthesis x right parenthesis equals limit as x rightwards arrow 0 of fraction numerator 2 open parentheses e to the power of sin space b x end exponent minus 1 close parentheses over denominator b x end fraction equals 2 equals limit as x rightwards arrow 0 of fraction numerator 2 open parentheses e to the power of sin space b x end exponent minus 1 close parentheses over denominator b x end fraction cross times fraction numerator sin space b x over denominator sin space b x end fraction equals limit as x rightwards arrow 0 of fraction numerator 2 open parentheses e to the power of sin space b x end exponent minus 1 close parentheses over denominator sin space b x end fraction cross times fraction numerator sin space b x over denominator b x end fraction equals 2 open square brackets limit as x rightwards arrow 0 of fraction numerator open parentheses e to the power of sin space b x end exponent minus 1 close parentheses over denominator sin space b x end fraction cross times limit as x rightwards arrow 0 of fraction numerator sin space b x over denominator b x end fraction close square brackets equals 2 f left parenthesis 0 right parenthesis equals 2 b space c a n space t a k e space a n y space r e a l space v a l u e space o t h e r space t h a n space 0.
Answered by Renu Varma | 23 Apr, 2020, 11:28: AM

Application Videos

Concept Videos

Multiple Choice Questions Practice Test
Answered Unanswered My Questions My Answers
JEE main - Maths
kho kho me kitne team khel sakte hain? options 1 6 2 4
Asked by amitraj39524 | 15 Feb, 2025, 05:15: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
find the equation of line passing through the point (3,2) and having slop 2
Asked by thosarrohit705 | 07 Feb, 2025, 18:08: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
If a number is completely divided by another number, can the quotient be an irrational number?
Asked by rathore6375 | 09 Jan, 2025, 10:52: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
in how many ways a team of 2 boys and 4 girls selected from 5 boys and 6 girls
Asked by pallavibirangal87 | 08 Dec, 2024, 10:18: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
if a chord of length 18 cm is 12 cm away from the centre then find the distance of 24 cm long chord from the centre
Asked by pritisindra6 | 06 Dec, 2024, 21:04: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
The number of ways in which at least one coin can be selected from 3 coins of one rupee, 4 coins of fifty paise, 5 coins of twenty paise and 6 coins of ten paise is-
Asked by kanishthkumawat | 29 Nov, 2024, 00:23: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
what is range of tan^-1 x
Asked by pawandhayal125 | 06 Oct, 2024, 10:03: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
what is 2+2
Asked by srishtipra2008 | 28 Sep, 2024, 10:33: AM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
n arithmetic means are inserted between the numbers 7 and 49. if the sum of these means is 364 then the sum of their squares is
Asked by yogeshsoni575 | 23 Sep, 2024, 23:01: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
JEE main - Maths
consider a dodecagon then number of ways of selecting two triangles which can be made using the vertices of dodecagon so that they do not have a common side.
Asked by sumit.parsudih | 17 Sep, 2024, 22:22: PM
ANSWERED BY EXPERT ANSWERED BY EXPERT
My Class Videos Tests ₹ Plans Ask a Doubt