JEE Class main Answered
A cylindrical block of mass m and height h floats vertically in a liquid which is twice as dense as the block, as shown in the figure. A uniform rod of mass m/2 and length I is hinged to axis of the cylinder at A and to the wall of the vessel at B. The rod can rotate freely about point B. It is observed that the rod is horizontal in its equilibrium position. Angular frequency of small vertical oscillations of the cylinder will be
m/2
B
12g V 7h
Asked by u_m67 | 09 Apr, 2022, 08:43: AM
Expert Answer
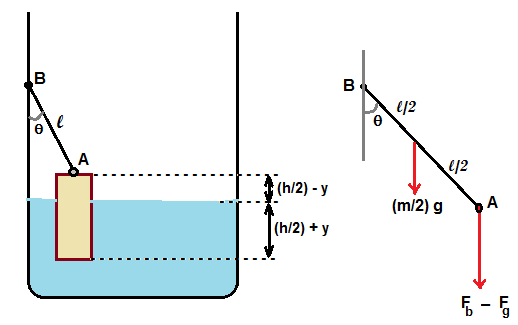
Initially cylinder of mass m and height h floats in the liquid at a depth h/2 . Let it be pressed to an additional depth y and be released .
The total torque acting on the rod is shown in figure
Total torque τ = [ ( Fb - Fg ) l sinθ ]- [ (m/2) g (l/2) sinθ ] .................................. (1)
where Fb is bouyant force from liquid , Fg is force due to gravity ( weight ) .
Force in upward direction is consdered as positive . Downward force is considered as negative .
( Fb - Fg ) = { ρl A [ (h/2) + y ] g } - [ ρc A h g ] .......................(2)
where ρ is density , subscript l denotes liquid and subscript c denotes cylinder. A is cross section area of cylinder and g is accelkeration due to gravity.
It is given that ρl = 2 ρc .............................(3)
Using eqn.(3) , we simplify eqn.(2) as
( Fb - Fg ) = 2 ρc y A g = 2 ( ρc h A ) (y/h) g = 2 m (y/h) g
Total torque from eqn.(1) is written as
Total torque τ = { [ 2 m (y/h) g l ] - [ (m/2) g (l/2) ]} sinθ = [ 2 (y/h) - (1/4) ] mgl sinθ
By Newton's law , Total torque τ = I α , where I = (1/3) m l2 is moment of inertia of rod about its end and α is angular acceleration .
τ = [ 2 (y/h) - (1/4) ] mgl sinθ = (1/3) m l2 ( d2θ / dt2 )
Above expression is written as
( d2θ / dt2 ) = - { ( 3/ l ) [ (1/4) - 2 (y/h) ] g } sinθ
Angular frequeny for small amlitude of oscillation can be written as
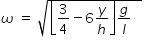
Answered by Thiyagarajan K | 10 Apr, 2022, 02:55: PM
JEE main - Physics
Asked by Machinenineha | 27 Mar, 2024, 05:28: PM
ANSWERED BY EXPERT
JEE main - Physics
Asked by tripathiji9876good | 10 Jan, 2024, 04:22: PM
ANSWERED BY EXPERTJEE main - Physics
Asked by meenu0000156 | 05 Jan, 2024, 12:05: PM
ANSWERED BY EXPERT
JEE main - Physics
Asked by joanmaria916 | 17 Jul, 2023, 11:24: AM
ANSWERED BY EXPERTJEE main - Physics
Asked by theavengers0203 | 22 May, 2023, 11:54: PM
ANSWERED BY EXPERT
JEE main - Physics
Asked by khushpatel9579 | 16 Feb, 2023, 07:50: PM
ANSWERED BY EXPERTJEE main - Physics
Asked by yadavashishkryadav | 13 Jan, 2023, 08:38: AM
ANSWERED BY EXPERT
