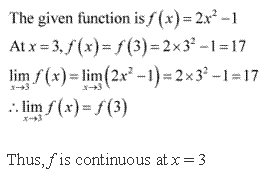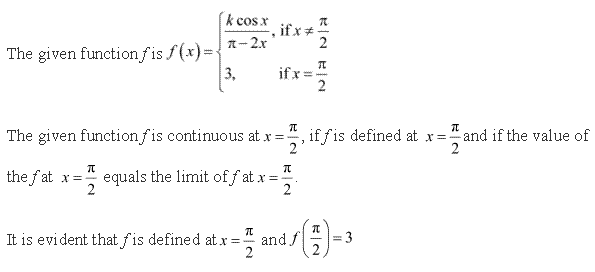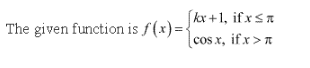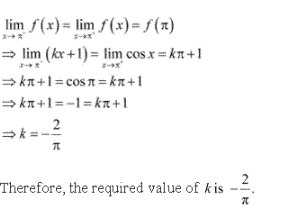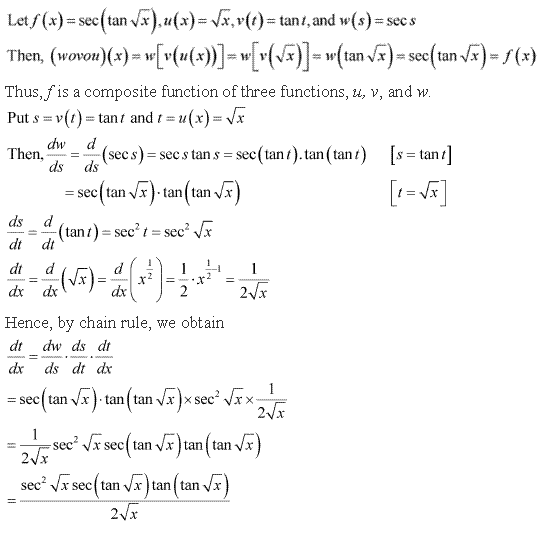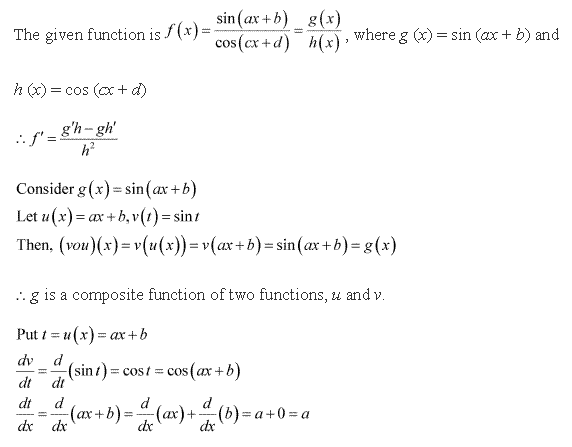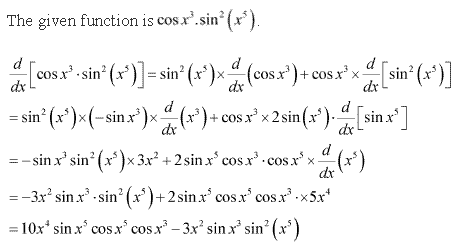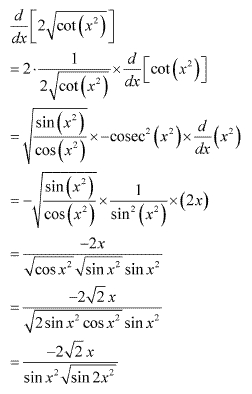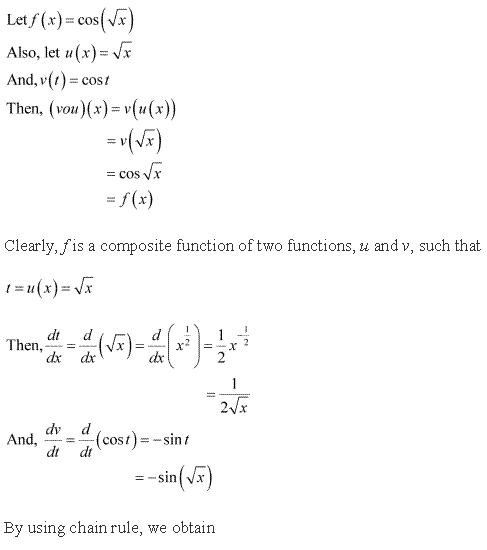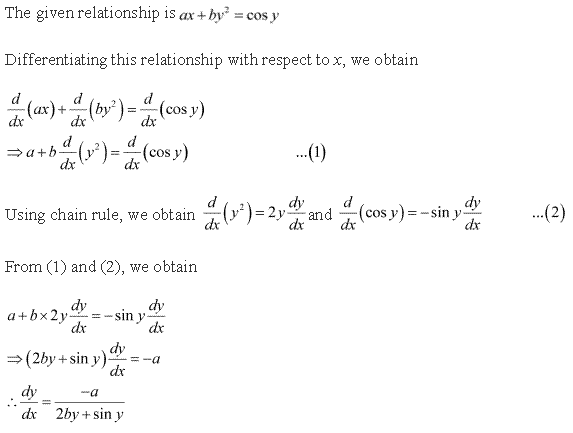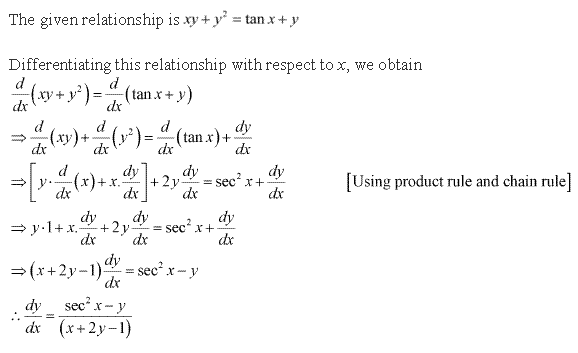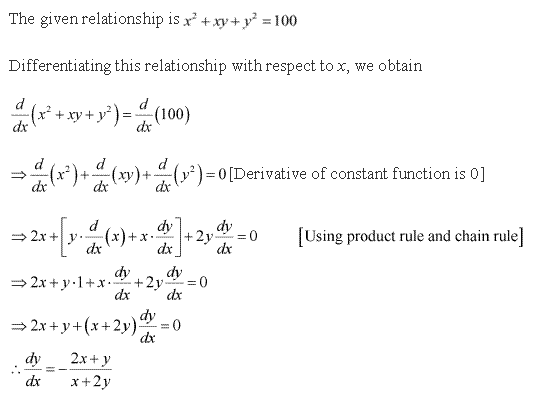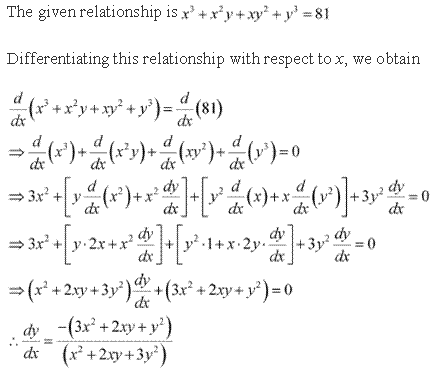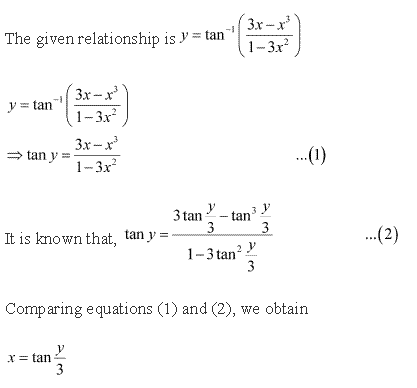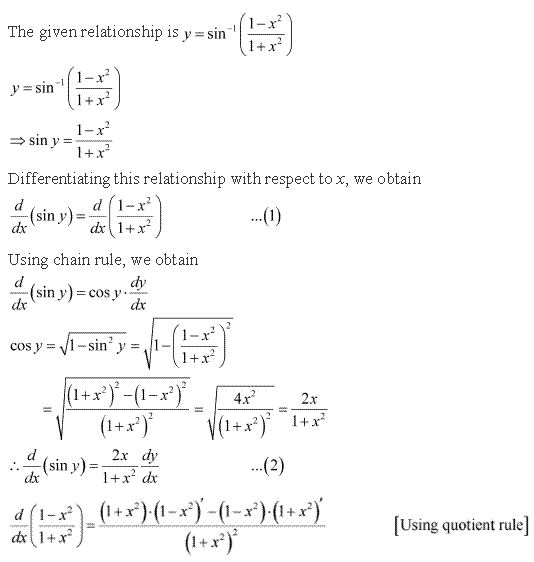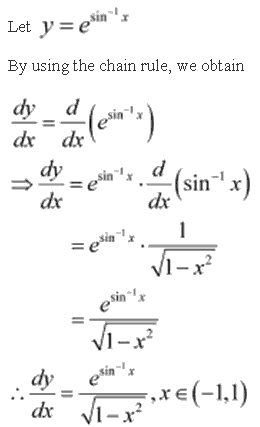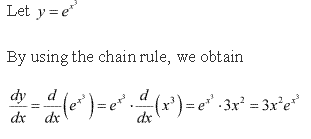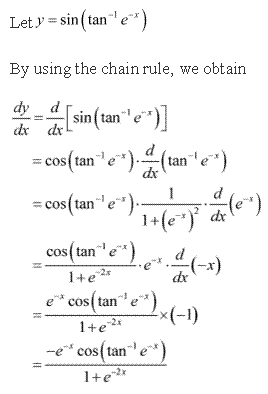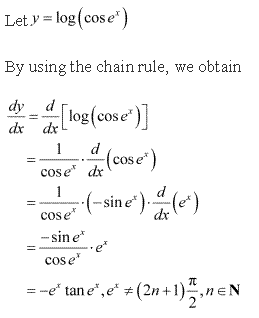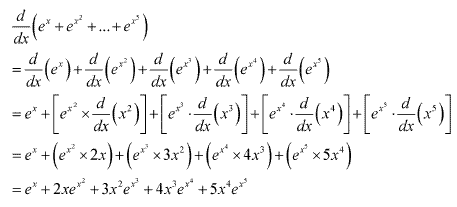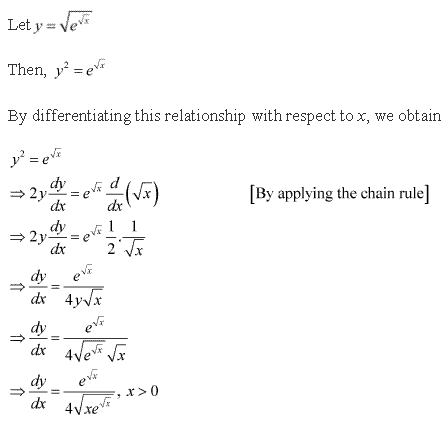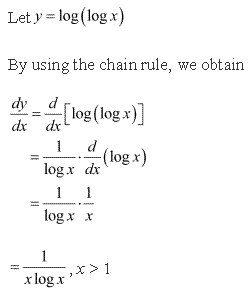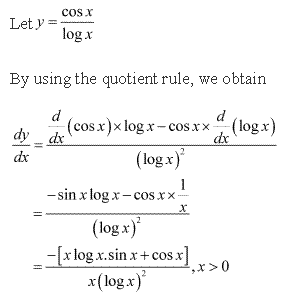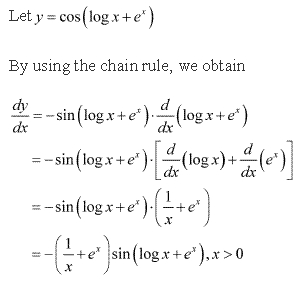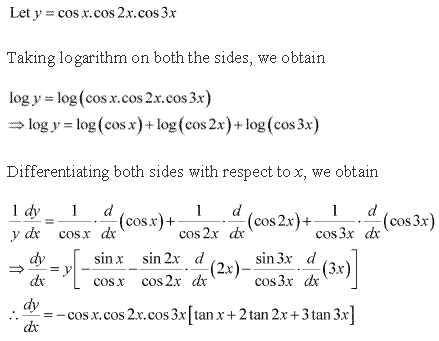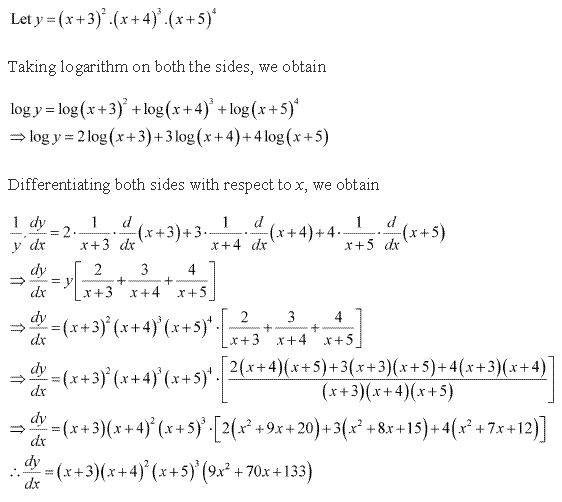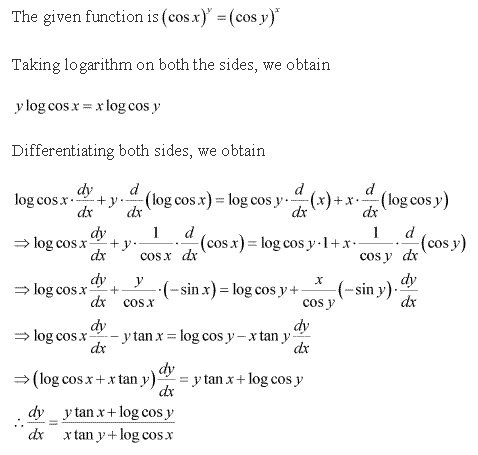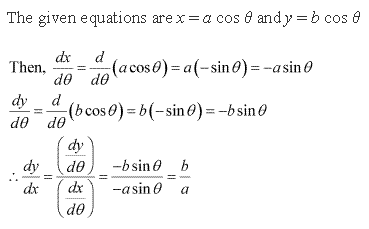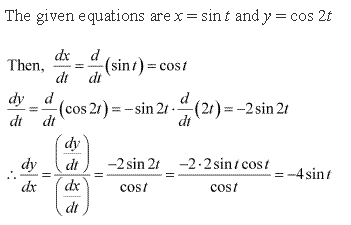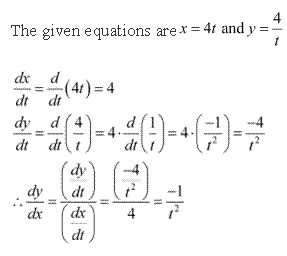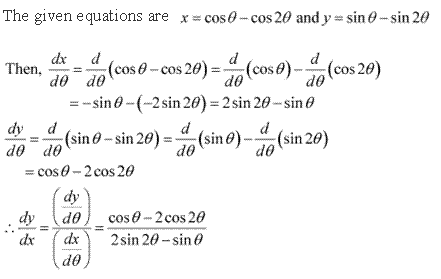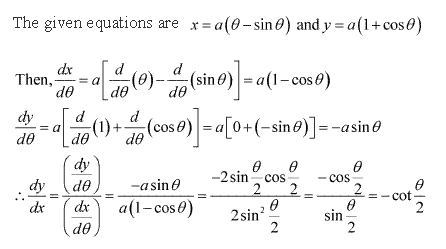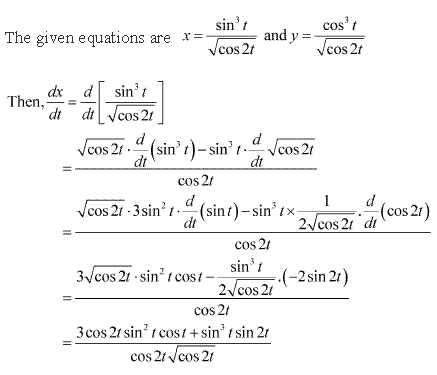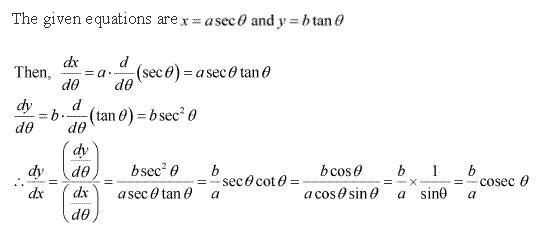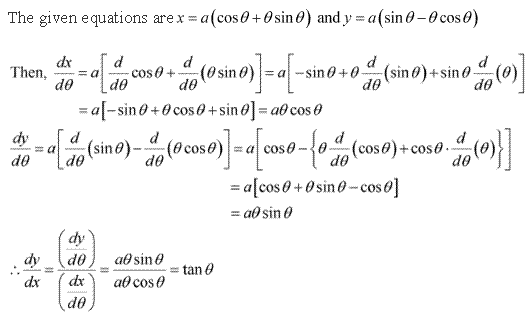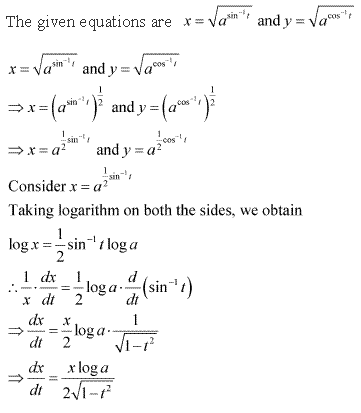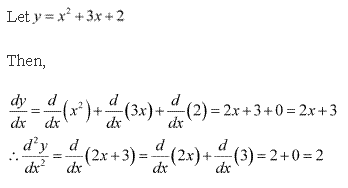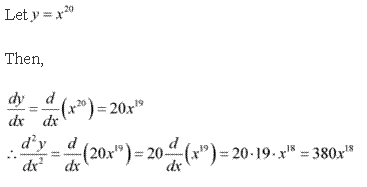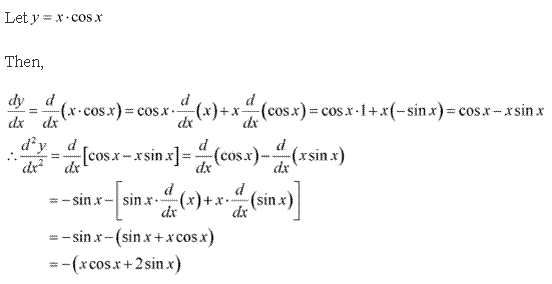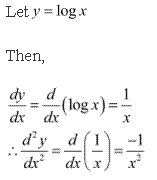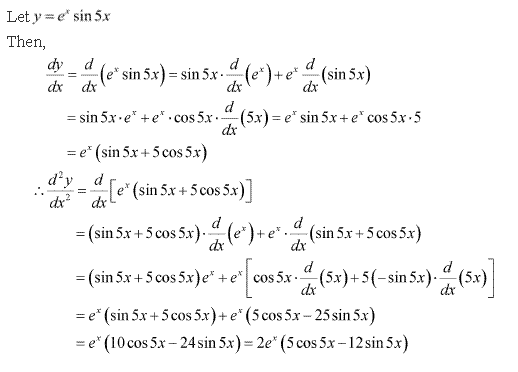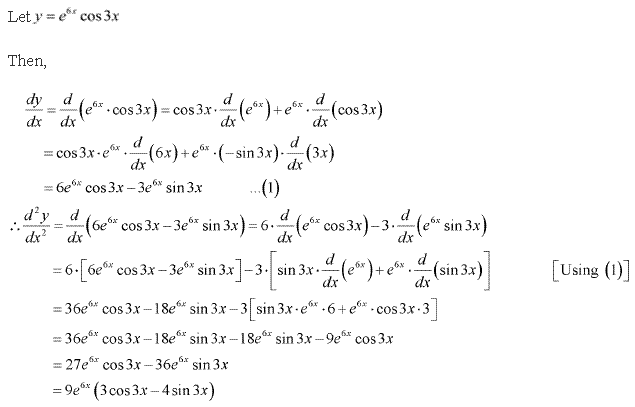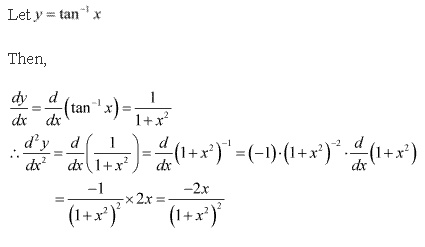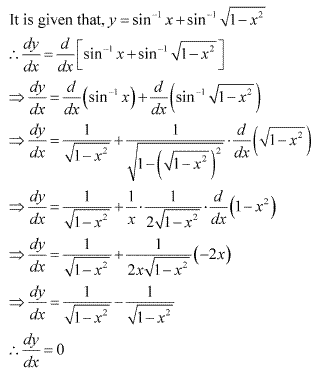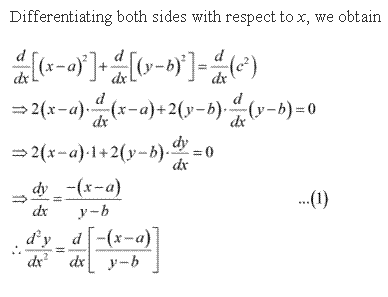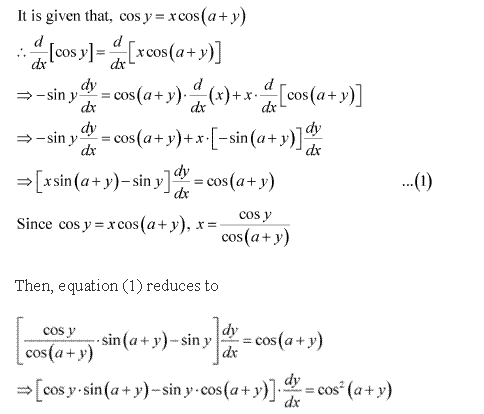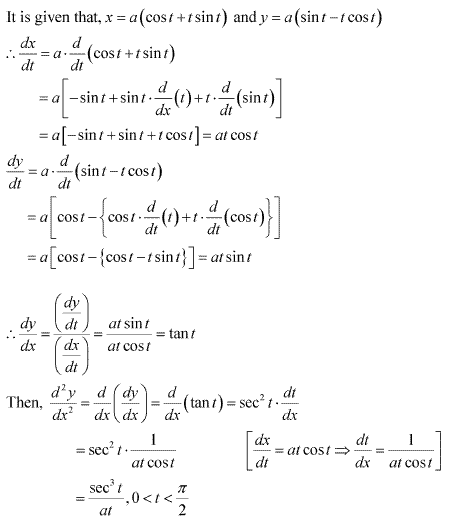Class 12-commerce NCERT Solutions Maths Chapter 5: Continuity and Differentiability
Continuity and Differentiability Exercise Ex. 5.1
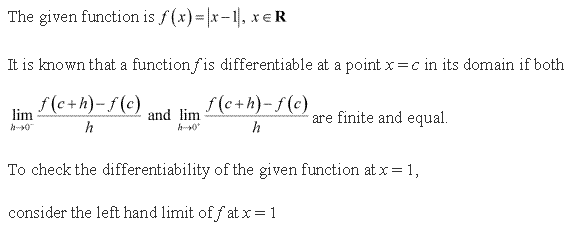
Solution 1
The given function is f(x) = 5x - 3
At x = 0, f(0) = 5 × 0 - 3 = -3
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
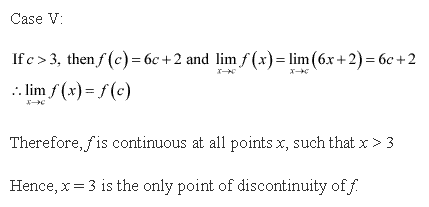
Solution 8
Solution 9
Solution 10
Solution 11
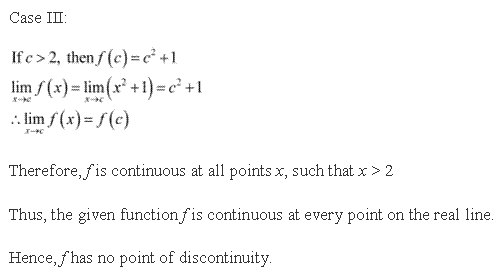
Solution 12
Solution 13
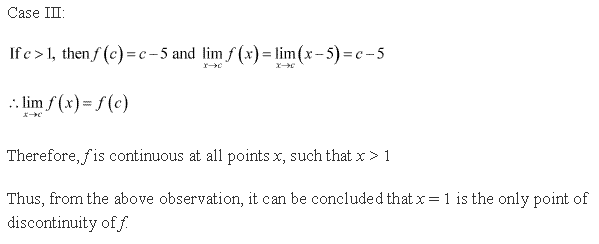
Solution 14
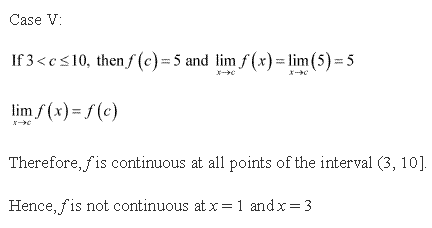
Solution 15
Solution 16
Solution 17
Solution 18
Solution 19
Solution 20
The given function is f(x) = x2 - sinx + 5
It is evident that f is defined at x = ∏
Solution 21
Solution 22
Z
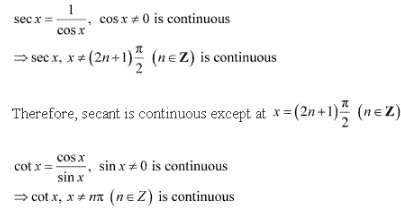
ZSolution 23
Solution 24
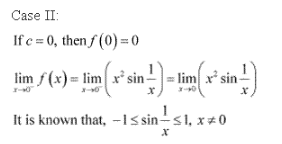
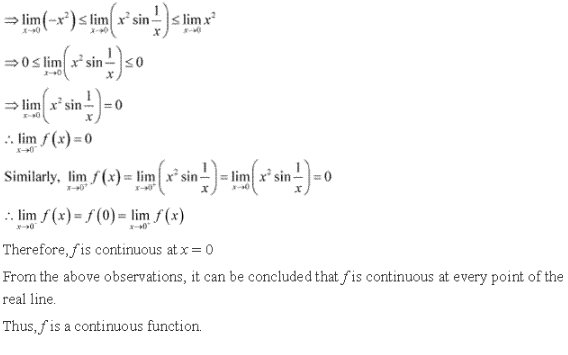
Solution 25
Solution 26
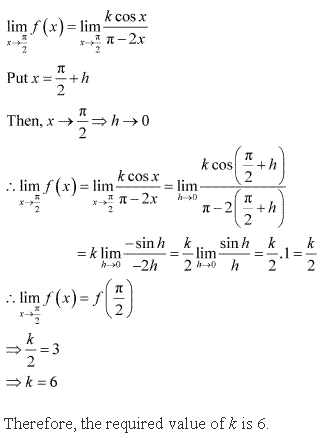
Solution 27
Solution 28
The given function f is continuous at x = 
, if f is defined at x = and if the value
of f at x = equals the limit of f at x =
Solution 29
Solution 30
Solution 31
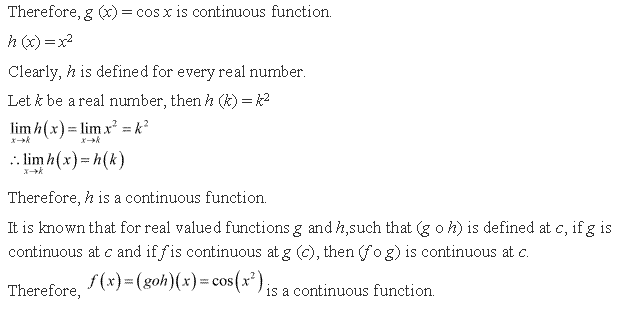
Solution 32
Solution 33
Solution 34
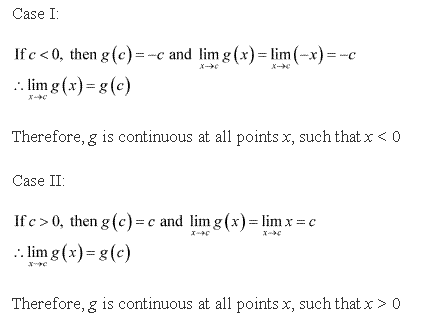
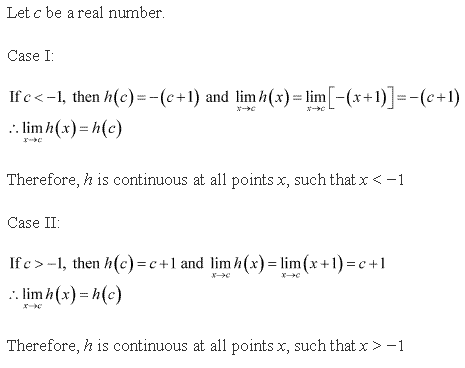
Continuity and Differentiability Exercise Ex. 5.2
Solution 1
Then, (v ο u)(x) = v(u(x)) = v(x2 + 5) = sin (x2 + 5) = f(x)
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
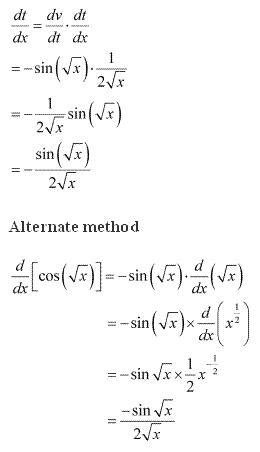
Solution 9
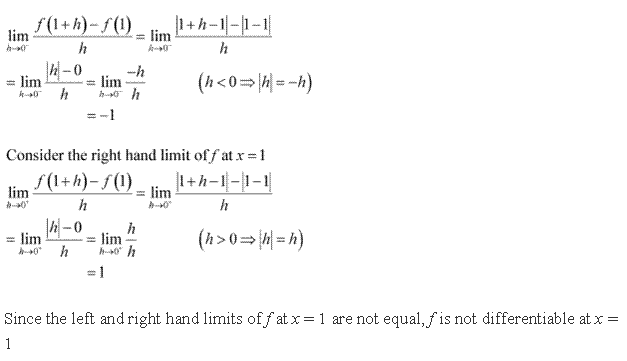
Solution 10
Continuity and Differentiability Exercise Ex. 5.3
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
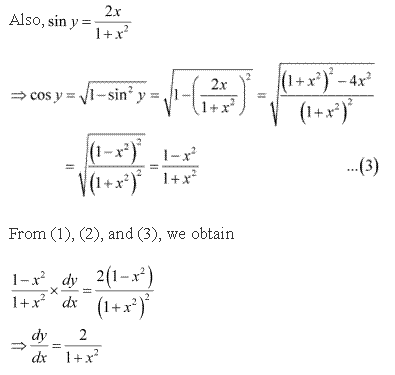
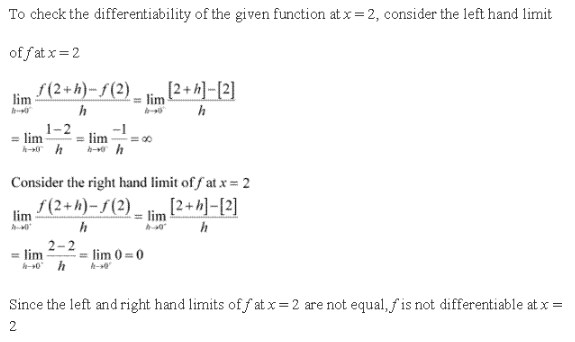
Solution 10
Solution 11
Solution 12
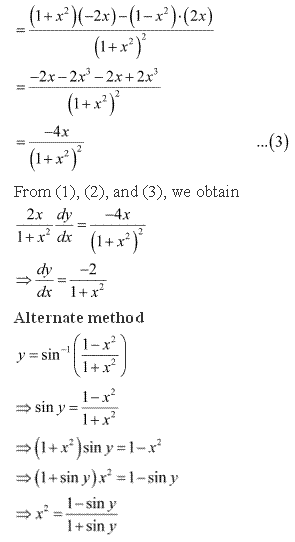
Solution 13
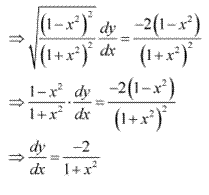
Solution 14
Solution 15
Continuity and Differentiability Exercise Ex. 5.4
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Continuity and Differentiability Exercise Ex. 5.5
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
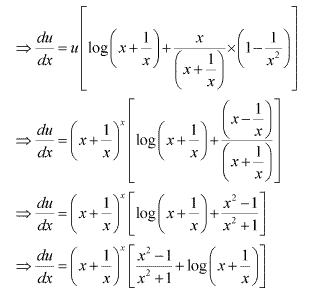
Solution 7
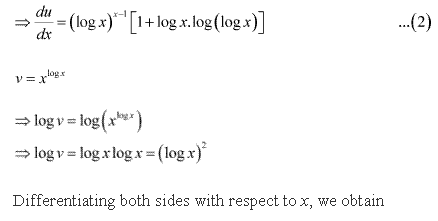
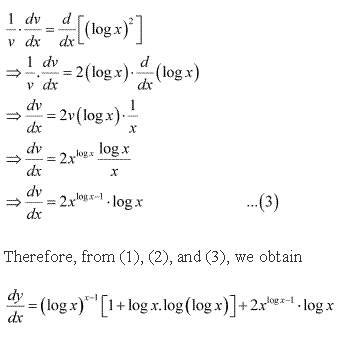
Solution 8
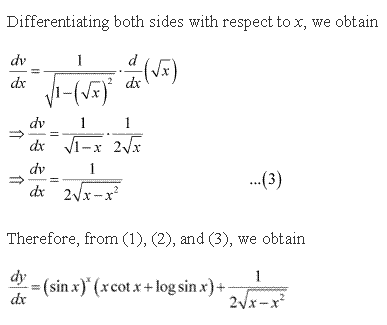
Solution 9
Solution 10
Solution 11
Solution 12
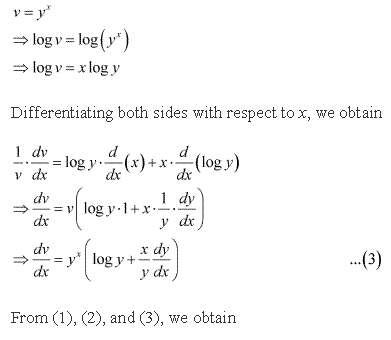
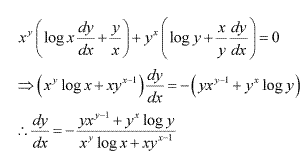
Solution 13
Solution 14
Solution 15
Solution 16
Solution 17
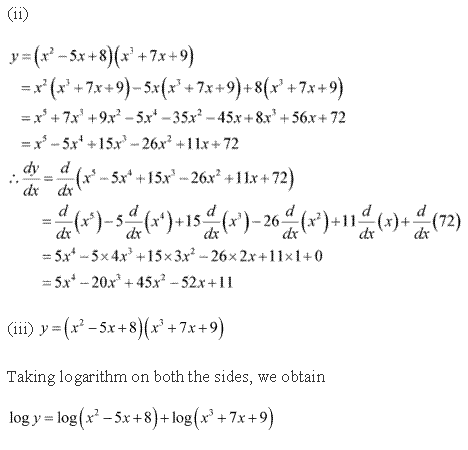
Solution 18
Continuity and Differentiability Exercise Ex. 5.6
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
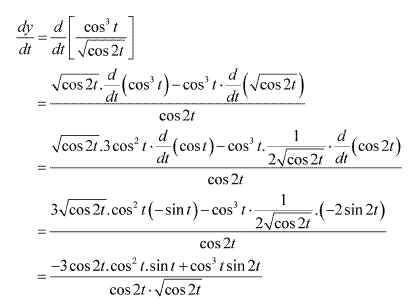
Solution 8
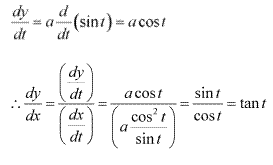
Solution 9
Solution 10
Solution 11
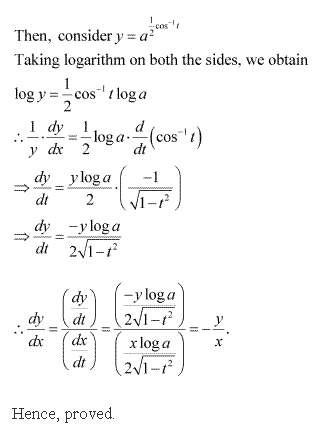
Continuity and Differentiability Exercise Ex. 5.7
Solution 1
Solution 2
Solution 3
Solution 4
Solution 5
Solution 6
Solution 7
Solution 8
Solution 9
Solution 10
Solution 11
Solution 12
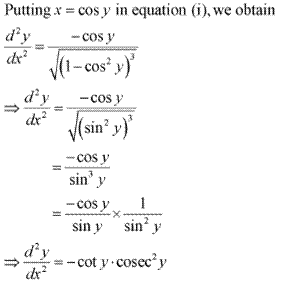
Solution 13
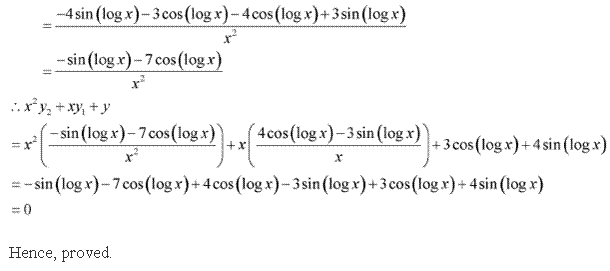
Solution 14
Solution 15
Solution 16
Solution 17
Continuity and Differentiability Exercise Misc. Ex.
Solution 1
Solution 2
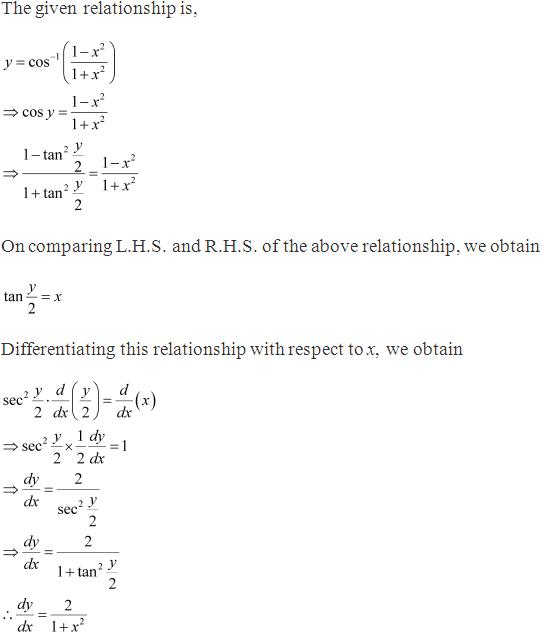
Solution 3
Solution 4
Solution 5
Solution 6
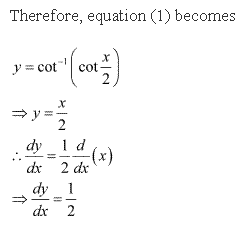
Solution 7
Solution 8
Solution 9
where sin x > cosx
Solution 10
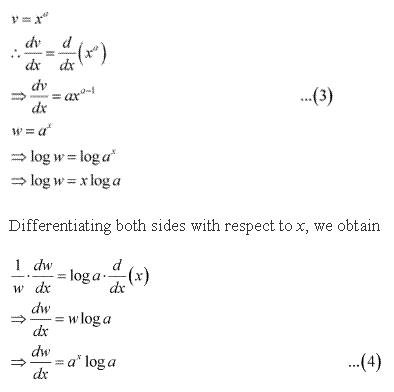
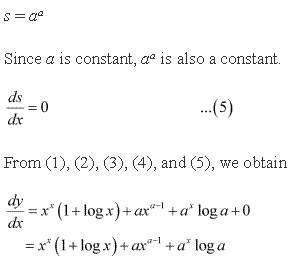
Solution 11
Solution 12
Solution 13
Solution 14
Solution 15
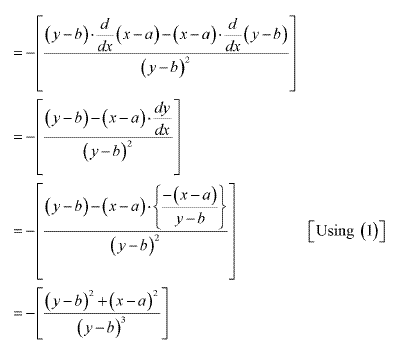
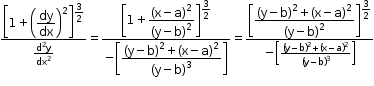
Solution 16
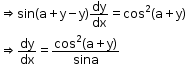
Solution 17
Solution 18
Solution 19
Solution 20
Yes.
Consider the function f(x)=|x-1|+|x-2|
Since we know that the modulus function is continuous everywhere, so there sum is also continuous
Therefore, function f is continuous everywhere
Now, let us check the differentiability of f(x) at x=1,2
At x=1
LHD = 
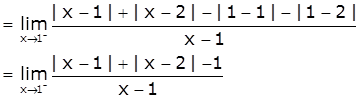
[Take x=1-h, h>0 such that h→0 as x→1-]
Now,
RHD = 
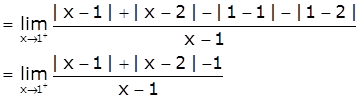
[Take x=1+h, h>0 such that h→0 as x→1+]
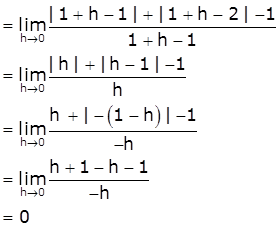
≠ LHD
Therefore, f is not differentiable at x=1.
At x=2
LHD = 
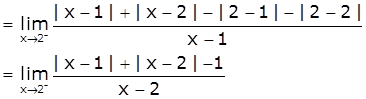
[Take x=2-h, h>0 such that h→0 as x→2-]
Now,
RHD = 
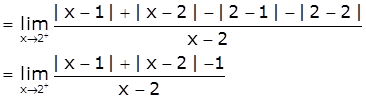
[Take x=2+h, h>0 such that h→0 as x→2+]
≠ LHD
Therefore, f is not differentiable at x=2.
Hence, f is not differentiable at exactly two points.
Solution 21
Solution 22
