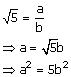Class 10 NCERT Solutions Maths Chapter 1 - Real Numbers

(i) 135 and 225

Step 1:  Since 225 > 135, apply Euclid's division lemma, to a =225 and b=135 to find q and r   such that 225 = 135q+r, 0  r
On dividing 225 by 135 we get quotient as 1 and remainder as 90
i.e 225 = 135 x 1 + 90

Step 2: Remainder r which is 90  0, we apply Euclid's division lemma to b =135 and r = 90 to find whole numbers q and r such that
135 = 90 x q + r,  0 r<90
On dividing 135 by 90 we get quotient as 1 and remainder as 45
i.e 135 = 90 x 1 + 45

Step 3:  Again remainder r = 45 0 so we apply Euclid's division lemma to b =90 and r = 45 to find q and r   such that
90 = 90 x q + r,    0 r<45
On dividing 90 by 45 we get quotient as 2 and remainder as 0
i.e 90 = 2 x 45 + 0

Step 4: Since the remainder is zero, the divisor at this stage will be HCF of (135, 225).  

Since the divisor at this stage is 45, therefore, the HCF of 135 and 225 is 45.

(ii)    196 and 38220
Step 1:  Since 38220 > 196, apply Euclid's division lemma
to a =38220 and b=196  to find  whole numbers q and r   such that
 38220 = 196 q + r, 0 r < 196
On dividing 38220 we get quotient as 195 and remainder r as 0
i.e 38220 = 196 x 195 + 0
Since the remainder is zero, divisor at this stage will be HCF
Since divisor at this stage is 196 , therefore, HCF of 196 and 38220 is 196.

NOTE: HCF( a,b) = a if a is a factor of  b. Here, 196 is a factor of  38220 so HCF is 196.
(iii)    867 and 255

Step 1: Since 867 > 255, apply Euclid's division lemma, to a =867 and b=255 to find q and r   such that 867 = 255q + r, 0 r<255
On dividing 867 by 255 we get quotient as 3 and remainder as 102
i.e 867 = 255 x 3 + 102

Step 2: Since remainder 102  0, we apply the division lemma to a=255 and b= 102 to find whole numbers q and r such that
255 = 102q + r where 0 r<102
On dividing 255 by 102 we get quotient as 2 and remainder as 51
i.e 255 = 102 x 2 + 51

Step 3: Again remainder 51 is non zero, so we apply the division lemma to a=102 and b= 51  to find whole numbers q and r such that
102 = 51 q + r where 0  r < 51
 
On dividing 102 by 51 quotient is 2 and remainder is 0
i.e 102 = 51 x 2 + 0
Since the remainder is zero, the divisor at this stage is the HCF
Since the divisor at this stage is 51,therefore, HCF of 867 and 255 is 51.

Concept Insight: To crack such problem remember to apply the Euclid's division Lemma which states that "Given positive integers a and b, there exists unique integers q and r satisfying a = bq + r, where 0 r < b" in the correct order.

Here, a > b.

Euclid's algorithm works since Dividing 'a' by 'b', replacing 'b' by 'r' and 'a' by 'b' and repeating the process of division till remainder 0 is reached, gives  a number which divides a and b exactly.

i.e    HCF(a,b) =HCF(b,r)

Note that do not find the HCF using prime factorisation in this question when the method is specified and do not skip steps.

Let a be any odd positive integer we need to prove that a is of the form 6q + 1 , or  6q + 3 , or 6q + 5 , where q is some integer.

Since a is an integer consider b = 6 another integer applying Euclid's division lemma  we get
a = 6q + r  for some integer q  0, and r = 0, 1, 2, 3, 4, 5  since
0  r < 6.
Therefore, a = 6q or 6q + 1 or 6q + 2 or 6q + 3 or 6q + 4 or 6q + 5
However since a is odd so a cannot take the values 6q, 6q+2 and 6q+4
(since all these are divisible by 2)

Also, 6q + 1 = 2 x 3q + 1 = 2k1 + 1, where k1 is a positive integer
6q + 3 = (6q + 2) + 1 = 2 (3q + 1) + 1 = 2k2 + 1, where k2 is an integer
6q + 5 = (6q + 4) + 1 = 2 (3q + 2) + 1 = 2k3 +  1, where k3 is an integer
Clearly, 6q + 1, 6q + 3, 6q + 5 are of the form 2k + 1, where k is an integer.
Therefore, 6q + 1, 6q + 3, 6q + 5 are odd numbers.

Therefore, any odd integer can be expressed is of the form
6q + 1, or 6q + 3, or 6q + 5 where q is some integer

Concept Insight:  In order to solve such problems  Euclid's division lemma is applied to two integers a and b the integer b must be taken in accordance with what is to be proved, for example here the integer b was taken 6 because a must  be of the form 6q + 1, 6q + 3, 6q + 5.

Basic definition of even and odd numbers and the fact that addition and , multiplication of  integers is always an integer are applicable here.

Maximum number of columns in which the Army contingent and the band can march will be given by HCF (616, 32)

We can use Euclid's algorithm to find the HCF.

Step 1: since 616 > 32 so applying Euclid's division lemma to a= 616 and b= 32 we get integers q and r as 32 and 19
i.e 616 = 32 x 19 + 8

Step 2: since remainder r =8  0 so again applying Euclid's  lemma to 32 and 8 we get  integers 4 and 0 as the quotient and remainder    
i.e 32 = 8 x 4 + 0
Step 3: Since remainder is zero so divisor at this stage will be the HCF

The HCF (616, 32) is 8.
Therefore, they can march in 8 columns each.

Concept Insight:  In order to solve the word problems first step is to interpret the problem and identify what is to be determined. The key word "Maximum" means we need to find the HCF.Do not forget to write the unit in the answer.

Let a be any positive integer we need to prove that a² is of the form 3m or 3m + 1 for some integer m.

Let b = 3 be  the other integer so applying Euclid's division lemma  to a and b=3
We get a = 3q + r for some integer q  0and r = 0, 1, 2
Therefore, a = 3q or 3q + 1 or 3q + 2
Now Consider a_²


Where k₁ = 3q_², k₂ =3q_²+2q   and k₃ = 3q_²+4q+1  since q ,2,3,1 etc are all integers so is their sum and product.
So k₁ k₂ k₃ are all integers.
Hence, it can be said that the square of any positive integer is either of the form 3m or 3m + 1 for any integer m.

Concept Insight: In order to solve such problems  Euclid's division lemma is applied to two integers a and b the integer b must be taken in accordance with what is to be proved, for example here the integer b was taken 3 because a must  be of the form 3m or 3m + 1. Do not forget to take a². Note that variable is just a notation and not the absolute value.

Let a be any positive integer and b = 3
a = 3q + r, where q 0 and 0 r < 3


Therefore, every number can be represented as these three forms. There are three cases.

Case 1:    When a = 3q,

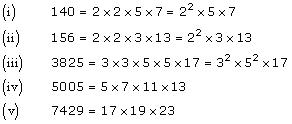
Concept Insight:  Since the given number needs to be expressed as the product of prime factors so in order to solve this problem knowing prime numbers is required. Do not forget to put the exponent in case a prime number is repeating.

Concept Insight: HCF is the product of common prime factors raised to least power, while LCM is product of prime factors raised to highest power. HCF is always a factor of the LCM.

Do not skip verification product of two numbers = HCF x LCM as it can help in cross checking the answer.
 

Concept Insight: HCF is the product of common prime factors of all three numbers  raised to least power, while LCM is product of prime factors of all here  raised to highest power.  Use the fact that HCF is always a factor of the LCM to verify the answer. Note HCF of (a,b,c)  can also be calculated by taking two numbers at a time i.e HCF (a,b) and then HCF (b,c) .

Concept Insight: This problem must be solved using product of two numbers = HCF x LCM rather then prime factorisation

If any number ends with the digit 0, it should be divisible by 10 or in other words its prime factorisation must include primes 2 and  5 both
Prime factorisation of 6^_n = (2 x 3)^_n

By Fundamental Theorem of Arithmetic Prime factorisation of a number is unique. So 5 is not a prime factor of 6^_n.
Hence, for any value of n, 6n will not be divisible by 5.
Therefore, 6^_n cannot end with the digit 0 for any natural number n.

Concept Insight: In order solve such problems the concept used is if a number is to end with zero then it must be divisible by 10 and the prime factorisation of a number is unique.

Numbers are of two types - prime and composite. Prime numbers has only two factors namely 1 and the number itself  whereas composite numbers have factors other than 1 and itself.

It can be observed that
7 x 11 x 13 + 13 = 13 x (7 x 11 + 1) = 13 x (77 + 1)
= 13 x 78
= 13 x 13 x 6

The given expression has 6 and 13  as its factors. Therefore, it is a composite number.
7 x 6 x 5 x 4 x 3 x 2 x 1 + 5 = 5 x (7 x 6 x  4 x 3 x 2 x 1 + 1)
 = 5 x (1008 + 1)
 = 5 x 1009

1009 cannot be factorised further. Therefore, the given expression has 5 and 1009 as its factors. Hence, it is a composite number.

Concept Insight: Definition of prime numbers and composite numbers is used. Do not miss the reasoning.

It can be observed that Ravi and Sonia does not take same amount of time Ravi takes lesser time than Sonia for completing 1 round of the circular path.

As they are going in the same direction, they will meet again at the same time when Ravi will have completed 1 round of that circular path with respect to Sonia.
i.e When  Sonia completes one round then ravi completes 1.5 rounds.   So they will meet first time at the time which is a common multiple of the time taken by them to complete 1 round

i.e LCM of 18 minutes and 12 minutes.
Now
18 = 2 x 3 x 3 = 2 x 3^₂
And, 12 = 2 x 2 x 3 = 2^₂ x 3

LCM of 12 and 18 = product of factors raised to highest exponent =
2² x 3² = 36  

Therefore, Ravi and Sonia will meet together at the starting point after 36 minutes.

Concept Insight: In order to solve the word problems first step is to interpret the problem and identify what is to be determined. The problem asks for simultaneous reoccurrence of events so we need to find LCM. The key word for simultaneous reoccurrence of events is LCM. Do not forget to write the final answer.

Let us assume, on the contrary that  is a rational number.

Therefore, we can find two integers a,b (b # 0) such that  = 

Where a and b are co-prime integers.

Therefore, a^₂ is divisible by 5 then a is also divisible by 5.

So a = 5k, for some  integer k.




This means that b² is divisible by 5 and hence, b is divisible by 5.
This implies that a and b have 5 as a common factor.
And this is a contradiction to the fact that a and b are co-prime.



Concept Insight: There are various ways of proving in mathematics proof by contradiction is one of them. In this approach we assume something which is contrary to what needs to be proved and arrive at a fact which contradicts something which is true in general. Key result used here is "If P is a prime number and it divides a^₂ then it divides a as well".

Therefore, we can find two integers a, b (b  0) such that




Concept Insight: This problem is solved using proof by contradiction. The key concept used is if p is prime number then  is irrational. Do not prove this question by assuming sum of rational and irrational is irrational.

Concept Insight: This problem is solved using proof by contradiction. The key concept used is if p is prime number then is irrational.Do not prove this question by assuming sum or product of rational and irrational is irrational.

3125 = 5^₅
The denominator is of the form 5^m.


8 = 2^₃
The denominator is of the form 2^m.


455 = 5 x 7 x 13
Since the denominator is not in the form 2^_m x 5^_n, and it also contains 7 and 13 as its factors, its decimal expansion will be non-terminating repeating.

The denominator is of the form 5^_n.

Hence the decimal expansion of  is terminating.

(ix)50 = 2 x 5 x 5

The denominator is of the form 2^m  5ⁿ.

30 = 2 x 3 x 5
Since the denominator is not of the form 2^m 5ⁿ, and it also has 3 as its factors,


Concept Insight: The  concept used in this problem is that

The decimal expansion of rational number  where p and q are coprime numbers,

terminates if and only if the prime factorisation of q is of the form 2ⁿ5^m, where n and m are non negative integers. Do not forget that 0 is also a non negative integer so n or m can take value 0.
Generally mistake is committed in identifying terminating decimals  when either of the two prime numbers  2 or 5 is appearing in the prime factorisation.

 

Concept Insight: This is  based on performing the long division and expressing the rational number in the decimal form learnt in lower classes.

(i)    43.123456789

Since this number has a terminating decimal expansion, it is a rational number of the form  and q is of the form 2^m x 5ⁿ,

i.e., the prime factors of q will be either 2 or 5 or both.

(ii)    0.120120012000120000...
The decimal expansion is neither terminating nor recurring. Therefore, the given number is an irrational number.




Since the decimal expansion is non-terminating recurring, the given number is a rational number of the form and q is not of the form 2_^m x 5_ⁿ  i.e., the prime factors of q will also have a

factor other than 2 or 5.


Concept Insight: The  concept used in this problem is that, 

If the decimal expansion of rational number  , [where p and q are coprime numbers] terminates, then  prime factorization of q is of the form 2ⁿ5^m, where n and m are non negative integers.