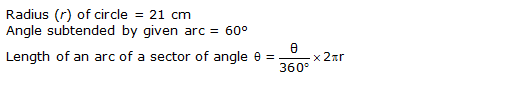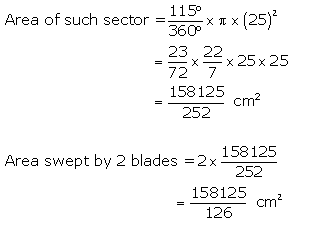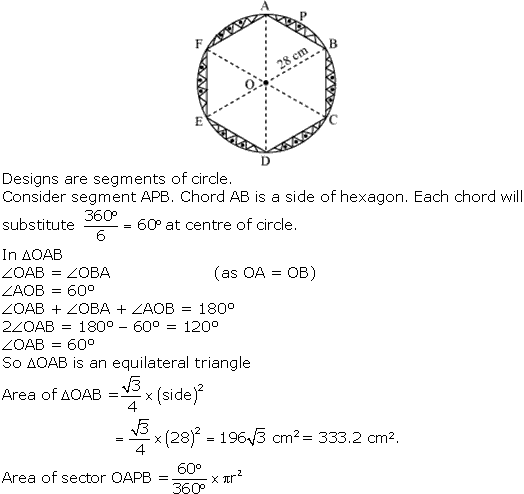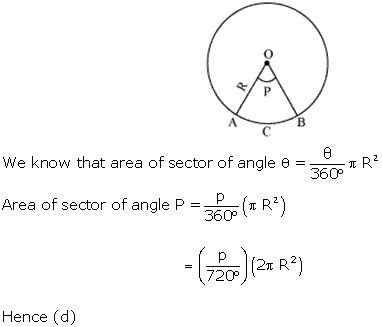Class 10 NCERT Solutions Maths Chapter 11 - Areas Related to Circles
Areas Related to Circles Exercise Ex. 11.1
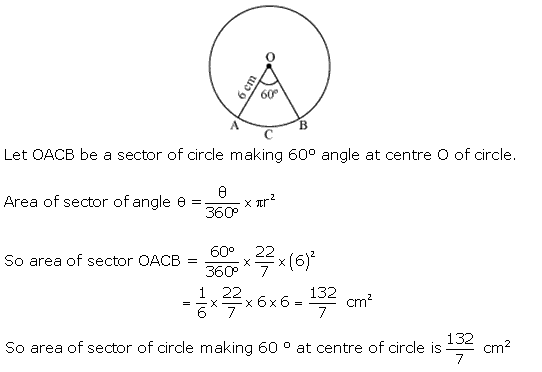
Solution 1
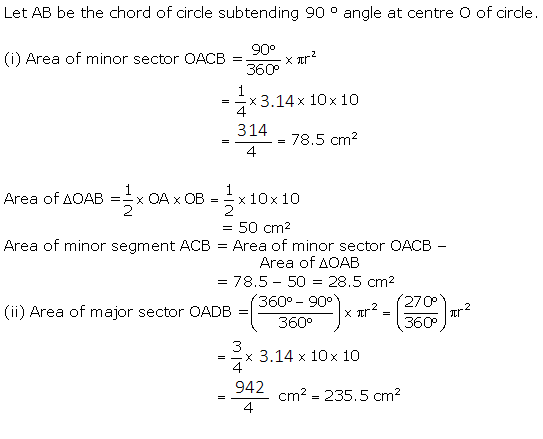
Solution 2
Solution 3
Solution 4
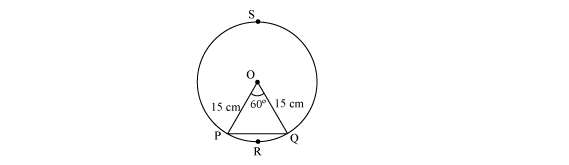
Solution 5
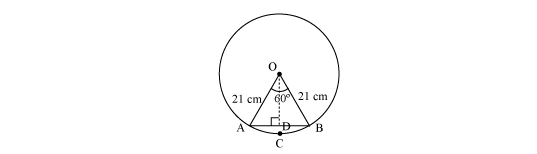
Solution 6
Radius (r) of the circle = 15
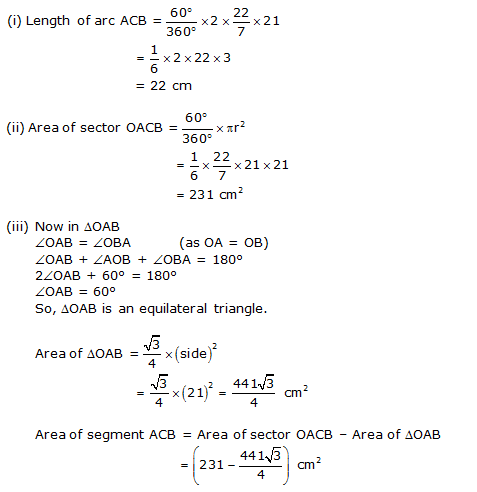
Area of sector OPRQ =![]()
=![]()
=![]()
= 117.75 cm2
In ∆OPQ
![]() .... (Since OP = OQ)
.... (Since OP = OQ)
![]()
\![]()
![]()
Area of ∆OPQ =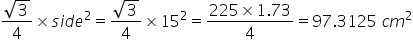
Area of segment PRQ = Area of sector OPRQ –Area of ∆OPQ
= 117.75 – 97.3125
= 20.4375 cm2
Area of major segment PSQ
= Area of circle – Area of segment PRQ
= 152p – 20.4375
= 3.14 × 225 – 20.4375
= 686.0625 cm2Solution 7
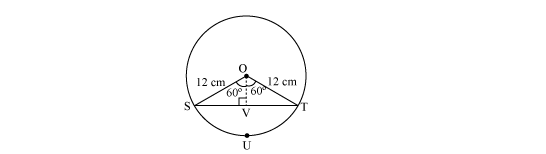
Draw a perpendicular OV on chord ST. It will bisect the chord ST.
SV = VT
In ∆OVS
![]()
![]()
OV = 6

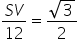
![]()
ST = 2SV = ![]()
Area of ∆OST = ![]()
= ![]()
= ![]()
= 36 × 1.73
= 62.28
Area of sector OSUT = ![]()
= 150.72
Area of segment SUT = Area of sector OSUT
= 150.72 – 62.28
= 88.44 cm2
Solution 8
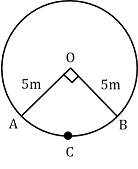
The horse can graze a sector of 90° in a circle of 5 m radius.
i. So area that can be grazed by horse = area of sector OACB
= ![]()
= ![]()
= 19.63 m2
ii. Area that can be grazed by the horse when the length of rope is 10 m long = ![]()
= ![]()
= 78.5
Change in grazing area = 78.5 – 19.63 = 58.87 cm2
Solution 9
Solution 10
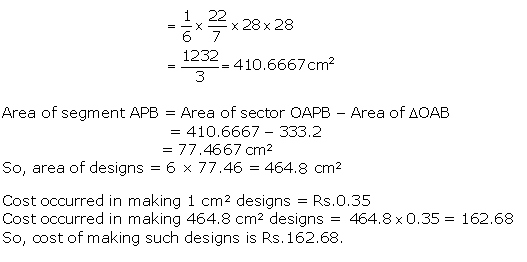
Solution 11
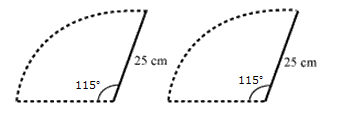
The figure shows that each blade of the wiper will sweep an area of a sector of 115° in a circle of 25 cm radius.