Class 9 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 5 - Quadrilaterals
Quadrilaterals Exercise 5.1
Solution 1
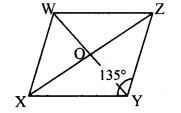
∠XYZ = 135°
and WXYZ is a parallelogram.
∠XWZ = ∠XYZ
∴ ∠XWZ = 135° …..(i)
∠YZW + ∠XYZ = 180° [Adjacent angles of a parallelogram are supplementary]
∴ ∠YZW + 135°= 180° [From (i)]
∴ ∠YZW = 180°- 135°
∴ ∠YZW = 45°
l(OY) = 5 cm [Given]
l(OY) =![]() ×
l(WY) [Diagonals of a parallelogram bisect each other]
×
l(WY) [Diagonals of a parallelogram bisect each other]
∴ l(WY) = 2 × l(OY)
= 2 × 5
∴ l(WY) = 10 cm
∴∠XWZ = 135°, ∠YZW = 45°, l(WY) = 10 cm
Solution 2
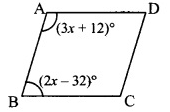
ABCD is a parallelogram. [Given]
∴ ∠A + ∠B = 180° [Adjacent angles of a parallelogram are supplementary],
∴ (3x + 12)° + (2x-32)° = 180°
∴ 3x + 12° + 2x - 32° = 180°
∴ 5x - 20° = 180°
∴ 5x= 180° + 20°
∴ 5x = 200°
![]()
∴ x = 40°
∠A = (3x + 12)°
= [3(40) + 12]°
=(120 +12)°= 132°
∠B = (2x - 32)°
= [2(40) - 32]°
= (80 - 32)° = 48°
∴ ∠C = ∠A = 132°
∠D = ∠B = 48° [Opposite angles of a parallelogram]
∴ The value of x is 40, and the measures of ∠C and ∠D are 132° and 48° respectively.
Solution 3
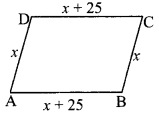
Let, ABCD be the parallelogram and the length of AD be x cm.
One side is greater than the other by 25 cm.
∴ AB = x + 25 cm
AD = BC = x cm
AB = DC = (x + 25) cm [Opposite angles of a parallelogram]
Perimeter of ABCD = 150 cm [Given]
∴ AB + BC + DC + AD = 150
∴ (x + 25) +x + (x + 25) + x - 150
∴ 4x + 50 = 150
∴ 4x = 150 - 50
∴ 4x = 100
![]()
∴ x = 25
AD = BC = x = 25 cm
AB = DC = x + 25 = 25 + 25 = 50 cm
∴ The lengths of the sides of the parallelogram are 25 cm, 50 cm, 25 cm and 50 cm.
Solution 4
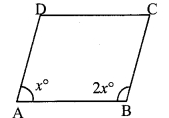
Let ABCD be the parallelogram.
The ratio of measures of two adjacent angles of a parallelogram is 1 : 2.
Let the common multiple be x.
∴ ∠A = x and ∠B = 2x
∠A + ∠B = 180° [Adjacent angles of a parallelogram are supplementary]
∴ x + 2x = 180°
∴ 3x = 180°
![]()
∴ x = 60°
∠A = x° = 60°
∠B = 2x° = 2 x 60° = 120°
∠A = ∠C = 60°
∠B = ∠D= 120° [Opposite angles of a parallelogram]
∴ The measures of the angles of the parallelogram are 60°, 120°, 60° and 120°.
Solution 5
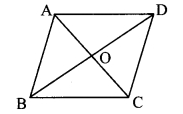
Given: AO = 5, BO = 12 and AB = 13.
To prove: □ABCD is a rhombus.
Solution:
Proof:
AO = 5, BO = 12, AB = 13 [Given]
AO² + BO² = 5² + 12²
= 25 + 144
∴ AO² + BO² = 169 …..(i)
AB² = 13² = 169 ….(ii)
∴ AB2 = AO² + BO² [From (i) and (ii)]
∴ ∆AOB is a right-angled triangle. [Converse of Pythagoras theorem]
∴ ∠AOB = 90°
∴ seg AC ⊥ seg BD …..(iii) [A-O-C]
∴ In parallelogram ABCD,
∴ seg AC ⊥ seg BD [From (iii)]
∴ ABCD is a rhombus. [A parallelogram is a rhombus perpendicular to each other]
Solution 6
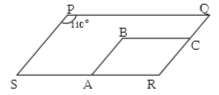
PQRS is a parallelogram. [Given]
∴ ∠R = ∠P [Opposite angles of a parallelogram]
∴ ∠R = 110° …..(iii)
ABCR is a parallelogram. [Given]
∴ ∠A + ∠R= 180° [Adjacent angles of a parallelogram are supplementary]
∴ ∠A+ 110°= 180° [From (i)]
∴ ∠A= 180°- 110°
∴ ∠A = 70°
∴ ∠C = ∠A = 70°
∴ ∠B = ∠R= 110° [Opposite angles of a parallelogram]
∴ ∠A = 70°, ∠B = 110°,
∴ ∠C = 70°, ∠R = 110°
Solution 7
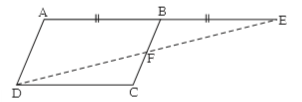
Given: ABCD is a parallelogram.
BE = AB
To prove: Line ED bisects seg BC at point F i.e. FC = FB
Solution:
Proof:
ABCD is a parallelogram. [Given]
∴ seg AB ≅ seg DC …….(i) [Opposite angles of a parallelogram]
seg AB ≅ seg BE ……..(ii) [Given]
seg DC ≅ seg BE ……..(iii) [From (i) and (ii)]
side DC || side AB [Opposite sides of a parallelogram]
i.e. side DC || seg AE and seg DE is their transversal. [A-B-E]
∴ ∠CDE ≅ ∠AED
∴ ∠CDF ≅ ∠BEF …..(iv) [D-F-E, A-B-E]
In ∆DFC and ∆EFB,
seg DC = seg EB [From (iii)]
∠CDF ≅ ∠BEF [From (iv)]
∠DFC ≅ ∠EFB [Vertically opposite angles]
∴ ∆DFC ≅ ∆EFB [SAA test]
∴ FC ≅ FB [c.s.c.t]
∴ Line ED bisects seg BC at point F.
Quadrilaterals Exercise 5.2
Solution 1

Given: ABCD is a parallelogram. P and Q are the midpoints of sides AB and DC respectively.
To prove: APCQ is a parallelogram.
Solution:
Proof:
AP =![]() AB
…..(i) [P is the midpoint of side AB]
AB
…..(i) [P is the midpoint of side AB]
QC =![]() DC
….(ii) [Q is the midpoint of side CD]
DC
….(ii) [Q is the midpoint of side CD]
ABCD is a parallelogram. [Given]
∴ AB = DC [Opposite sides of a parallelogram]
∴![]() AB
=
AB
=![]() DC [Multiplying both
sides by 12]
DC [Multiplying both
sides by 12]
∴ AP = QC ….(iii) [From (i) and (ii)]
Also, AB || DC [Opposite angles of a parallelogram]
i.e. AP || QC ….(iv) [A - P - B, D - Q - C]
From (iii) and (iv),
APCQ is a parallelogram. [A quadrilateral is a parallelogram if its opposite sides is parallel and congruent]
Solution 2
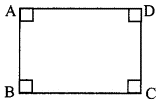
Given:
ABCD is a rectangle.
To prove: Rectangle ABCD is a parallelogram.
Solution:
Proof:
ABCD is a rectangle.
∴ ∠A ≅ ∠C = 90° [Given]
∠B ≅ ∠D = 90° [Angles of a rectangle]
∴ Rectangle ABCD is a parallelogram. [A quadrilateral is a parallelogram, if pairs of its opposite angles are congruent]
Solution 3
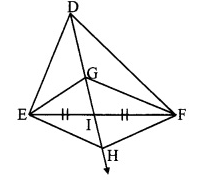
Given: Point G (centroid) is the point of concurrence of the medians of ∆DEF. DG = GH
To prove: GEHF is a parallelogram.
Solution:
Proof:
Let ray DH intersect seg EF at point I such that E-I-F.
∴ seg DI is the median of ∆DEF.
∴ El = FI ……(i)
Point G is the centroid of ∆DEF.
![]() [Centroid
divides each median in the ratio 2:1]
[Centroid
divides each median in the ratio 2:1]
∴ DG = 2(GI)
∴ GH = 2(GI) [DG = GH]
∴ GI + HI = 2(GI) [G-I-H]
∴ HI = 2(GI) - GI
∴ HI = GI ….(ii)
From (i) and (ii),
GEHF is a parallelogram [A quadrilateral is a parallelogram, if its diagonals bisect each other]
Solution 4
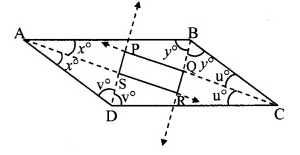
Given: ABCD is a parallelogram.
Rays AS, BQ, CQ and DS bisect ∠A, ∠B, ∠C and ∠D respectively.
To prove: PQRS is a rectangle.
Solution:
Proof:
∠BAS = ∠DAS = x° …(i) [ray AS bisects ∠A]
∠ABQ = ∠CBQ =y° ….(ii) [ray BQ bisects ∠B]
∠BCQ = ∠DCQ = u° …..(iii) [ray CQ bisects ∠C]
∠ADS = ∠CDS = v° ….(iv) [ray DS bisects ∠D]
□ABCD is a parallelogram. [Given]
∴ ∠A + ∠B = 180° [Adjacent angles of a parallelogram are supplementary]
∴ ∠BAS + ∠DAS + ∠ABQ + ∠CBQ = 180° [Angle addition property]
∴ x°+x°+ v° + v° = 180 [From (i) and (ii)]
∴ 2x° + 2v° =180
∴ x + y = 90° ……(v) [Dividing both sides by 2]
Also, ∠A + ∠D= 180° [Adjacent angles of a parallelogram are supplementary]
∴ ∠BAS + ∠DAS + ADS + ∠CDS = 180° [Angle addition property]
∴ x° + x° + v° + v° = 180°
∴ 2x° + 2v° = 180°
∴ x° + v° = 90° …..(vi) [Dividing both sides by 2]
In ∆ARB,
∠RAB + ∠RBA + ∠ARB = 180° [Sum of the measures of the angles of a triangle is 180°]
∴ x° + y° + ∠SRQ = 180° [A - S - R, B - Q - R]
∴ 90° + ∠SRQ = 180° [From (v)
∴ ∠SRQ = 180°- 90° = 90° …..(vi)
Similarly, we can prove
∠SPQ = 90° …(viii)
In ∆ASD,
∠ASD + ∠SAD + ∠SDA = 180° [Sum of the measures of angles a triangle is 180°]
∴ ∠ASD + x° + v° = 180° [From (vi)]
∴ ∠ASD + 90° = 180°
∴∠ASD = 180°- 90° = 90°
∴ ∠PSR = ∠ASD [Vertically opposite angles]
∴ ∠PSR = 90° …..(ix)
Similarly we can prove
∠PQR = 90° ..(x)
∴ In PQRS,
∠SRQ = ∠SPQ = ∠PSR = ∠PQR = 90° [From (vii), (viii), (ix), (x)]
∴ PQRS is a rectangle. [Each angle is of measure 90°]
Solution 5
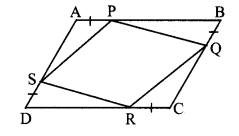
Given: ABCD is a parallelogram.
AP = BQ = CR = DS
To prove: PQRS is a parallelogram.
Solution:
Proof:
ABCD is a parallelogram. [Given]
∴ ∠B = ∠D ….(i) [Opposite angles of a parallelogram]
Also, AB = CD [Opposite sides of a parallelogram]
∴ AP + BP = DR + CR [A-P-B, D-R-C]
∴ AP + BP = DR + AP [AP = CR]
∴ BP = DR ….(ii)
In APBQ and ARDS,
seg BP ≅ seg DR [From (ii)]
∠PBQ ≅ ∠RDS [From (i)]
seg BQ ≅ seg DS [Given]
∴ ∆PBQ ≅ ∆RDS [SAS test]
∴ seg PQ ≅ seg RS …..(iii) [c.s.c.t]
Similarly, we can prove that
∆PAS ≅ ∆RCQ
∴ seg PS ≅ seg RQ ….(iv) [c.s.c.t]
From (iii) and (iv),
PQRS is a parallelogram. [A quadrilateral is a parallelogram, if pairs of its opposite angles are congruent]
Quadrilaterals Exercise 5.3
Solution 1
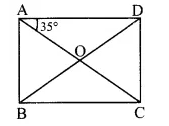
AC = 8 cm …(i) [Given]
ABCD is a rectangle [Given]
∴ BD = AC [Diagonals of a rectangle are congruent]
∴ BD = 8 cm [From (i)]
BO = 1/2 BD [Diagonals of a rectangle bisect each other]
∴ BO = 1/2 x 8
∴ BO = 4 cm
Side AD || side BC and seg AC is their transversal. [Opposite sides of a rectangle are parallel]
∴ ∠ACB = ∠CAD [Alternate angles]
∠ACB = 35° [ ∵∠CAD = 35°]
∴ BO = 4 cm, ∠ACB = 35°
Solution 2
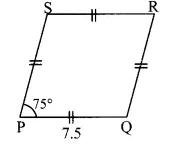
PQ = 7.5 cm [Given]
PQRS is a rhombus. [Given]
∴ QR = PQ [Sides of a rhombus are congruent]
∴ QR = 7.5 cm
∠QPS = 75° [Given]
∠QPS + ∠PQR = 180° [Adjacent angles of a rhombus are supplementary]∴ 75° + ∠PQR = 180°
∴ ∠PQR = 180° - 75°
∴ ∠PQR =105°
∠SRQ = ∠QPS [Opposite angles of a rhombus]
∴ ∠SRQ = 75°
∴ QR = 7.5 cm, ∠PQR = 105°,
∠SRQ = 75°
Solution 3

IJKL is a square. [Given]
∴ seg IK ⊥ seg JL [Diagonals of a square are perpendicular to each other]
∠ IMJ=90°
∠ JIL = 90° ……. (i) [Angle of a square]
∠JIK = 1/2∠JIL [Diagonals of a square bisect the opposite angles]
∠JIK = 1/2 (90°) [From (i)
∴ ∠JIK = 45°
∠IJK = 90° (ii) [Angle of a square]
∠LJK = 1/2∠IJK [Diagonals of a square bisect the opposite angles]
∠LJK = 1/2 (90°) [From (ii)]
∴ ∠LJK = 45°
∴ ∠LJK = 90°, ∠JIK = 45°, ∠LJK=45°
Solution 4

Let ABCD be the rhombus. AC = 20 cm, BD = 21 cm
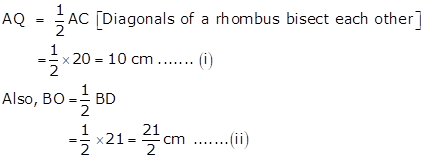
In ∆AOB, ∠AOB = 90° [Diagonals of a rhombus are perpendicular to each other]
∴ AB² = AO² + BO² [Pythagoras theorem]
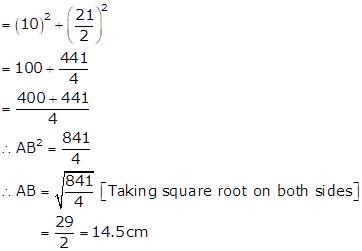
Perimeter of ABCD
= 4 x AB = 4 x 14.5 = 58 cm
∴ The side and perimeter of the rhombus are 14.5 cm and 58 cm respectively.
Solution 5
i. False.
All the sides of a rhombus are congruent, while the opposite sides of a parallelogram are congruent.
ii. False.
All the angles of a rectangle are congruent, while the opposite angles of a rhombus are congruent.
iii. True.
The opposite sides of a parallelogram are parallel and congruent. Also, its opposite angles are congruent.
The opposite sides of a rectangle are parallel and congruent. Also, all its angles are congruent.
iv. True.
The opposite sides of a rectangle are parallel and congruent. Also, all its angles are congruent.
All the sides of a square are parallel and congruent. Also, all its angles are congruent.
v. True.
All the sides of a rhombus are congruent. Also, its diagonals are perpendicular bisectors of each other.
All the sides of a square are congruent. Also, its diagonals are perpendicular bisectors of each other.
vi. False.
All the angles of a rectangle are congruent, while the opposite angles of a parallelogram are congruent.
Quadrilaterals Exercise 5.4
Solution 1
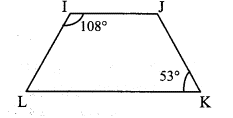
∠I = 108° [Given]
Side IJ || side KL and side IL is their transversal. [Given]
∴ ∠I + ∠L = 180° [Interior angles]
∴ 108° + ∠L = 180°
∴ ∠L = 180° - 108° = 72°
∠K = 53° [Given]
Side IJ || side KL and side JK is their transversal. [Given]
∴ ∠J + ∠K = 180° [Interior angles]
∴ ∠J + 53° = 180°
∴ ∠J= 180°- 53° = 127°
∴ ∠L = 72°, ∠J = 127°
Solution 2
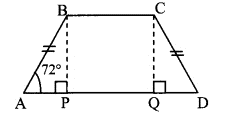
∠A = 72° [Given]
In □ABCD, side BC || side AD and side AB is their transversal. [Given]
∴ ∠A + ∠B = 180° [Interior angles]
∴ 72° +∠B = 180°
∴ ∠B = 180° - 72° = 108°
In ∆BPA and ∆CQD,
∠BPA ≅ ∠CQD [Each angle is of measure 90°]
Hypotenuse AB ≅ Hypotenuse DC [Given]
seg BP ≅ seg CQ [Perpendicular distance between two parallel lines]
∴ ∆BPA ≅ ∆CQD [Hypotenuse side test]
∴ ∠BAP ≅ ∠CDQ [c. a. c. t.]
∴ ∠A = ∠D
∴ ∠D = 72°
∴ ∠B = 108°, ∠D = 72°
Solution 3
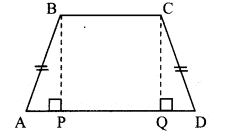
Given: side BC < side AD, side BC || side AD, side BA = side CD
To prove: ∠ABC ≅ ∠DCB
Construction: Draw seg BP ⊥ side AD, (A - P - D)
seg CQ ⊥ side AD, (A - Q - D)
Solution:
Proof:
In ∆BPA and ∆CQD,
∠BPA ≅ ∠CQD [Each angle is of measure 90°]
Hypotenuse BA ≅ Hypotenuse CD [Given]
seg BP ≅ seg CQ [Perpendicular distance between two parallel lines]
∴ ∆BPA ≅ ∆CQD [Hypotenuse side test]
∴ ∠BAP ≅ ∠CDQ [c. a. c. t.]
∴ ∠A = ∠D ….(i)
Now, side BC || side AD and side AB is their transversal. [Given]
∴ ∠A + ∠B = 180°…..(ii) [Interior angles]
Also, side BC || side AD and side CD is their transversal. [Given]
∴ ∠C + ∠D = 180° …..(iii) [Interior angles]
∴ ∠A + ∠B = ∠C + ∠D [From (ii) and (iii)]
∴ ∠A + ∠B = ∠C + ∠A [From (i)]
∴ ∠B = ∠C
∴ ∠ABC ≅ ∠DCB
Quadrilaterals Exercise 5.5
Solution 1
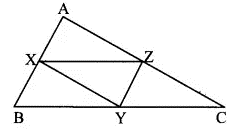
AC = 9 cm [Given]
Points X and Y are the midpoints of sides AB and BC respectively. [Given]
AB = 5 cm [Given]
Points Y and Z are the midpoints of sides BC and AC respectively. [Given]
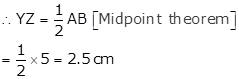
BC = 11 cm [Given]
Points X and Z are the midpoints of sides AB and AC respectively. [Given]
∴ XZ =![]() BC
[Midpoint theorem]
BC
[Midpoint theorem]
=![]() ×
11 = 5.5 cm
×
11 = 5.5 cm
l(XY) = 4.5 cm, l(YZ) = 2.5 cm, l(XZ) = 5.5 cm
Solution 2
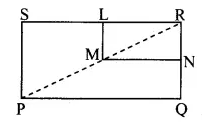
Given: PQRS and MNRL are rectangles. M is the midpoint of side PR.
Solution:
To prove:
i. SL = LR
ii. LN =![]() (SQ)
(SQ)
Proof:
PQRS and MNRL are rectangles. [Given]
∴ ∠S = ∠L = 90° [Angles of rectangles]
∠S and ∠L form a pair of corresponding angles on sides SP and LM when SR is their transversal.
∴eg, ML || seg PS …(i) [Corresponding angles test]
In ∆PRS,
Point M is the midpoint of PR and seg ML || seg PS. [Given] [From (i)]
∴ Point L is the midpoint of seg SR. ……(ii) [Converse of midpoint theorem]
∴ SL = LR
Similarly, for ∆PRQ, we can prove that,
Point N is the midpoint of seg QR. ….(iii)
In ∆RSQ,
Points L and N are the midpoints of seg SR and seg QR respectively. [From (ii) and (iii)]
∴ LN =![]() SQ
[Midpoint theorem]
SQ
[Midpoint theorem]
Solution 3
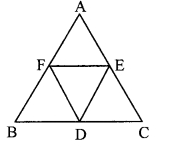
Given: ∆ABC is an equilateral triangle.
Points F, D and E are midpoints of side AB, side BC, side AC respectively.
To prove: ∆FED is an equilateral triangle.
Solution:
Proof:
∆ABC is an equilateral triangle. [Given]
∴ AB = BC = AC ….(i) [Sides of an equilateral triangle]
Points F, D and E are midpoints of side AB and BC respectively.
∴ FD =![]() AC
…..(ii) [Midpoint theorem]
AC
…..(ii) [Midpoint theorem]
Points D and E are the midpoints of sides BC and AC respectively.
![]()
Points F and E are the midpoints of sides AB and AC respectively.
∴ FE =![]() BC
BC
∴ FD = DE = FE [From (i), (ii), (iii) and (iv) ]
∴ ∆FED is an equilateral triangle.
Solution 4
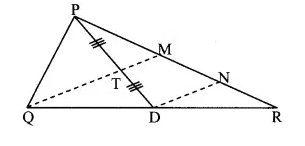
Given: seg PD is a median of ∆PQR. Point T is the midpoint of seg PD.
To Prove: ![]()
Construction: Draw seg DN || seg QM such that P-M-N and M-N-R.
Proof:
In ∆PDN,
Point T is the midpoint of seg PD and seg TM || seg DN [Given]
∴ Point M is the midpoint of seg PN. [Construction and Q-T-M]
∴ PM = MN [Converse of midpoint theorem]
In ∆QMR,
Point D is the midpoint of seg QR and seg DN || seg QM [Construction]
∴ Point N is the midpoint of seg MR. [Converse of midpoint theorem]
∴ RN = MN …..(ii)
∴ PM = MN = RN …..(iii) [From (i) and (ii)]
Now, PR = PM + MN + RN [ P-M-R-Q-T-M]
∴ PR = PM + PM + PM [From (iii) ]
∴ PR = 3PM
![]()
Quadrilaterals Exercise Problem Set 5
Solution 1(i)
(d) rhombus
Solution 1(ii)
In ∆ABC,
AC² = AB² + BC²
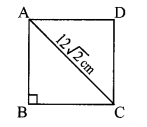
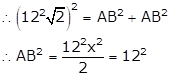
∴ AB = 12 cm
∴ Perimeter of ABCD = 4 x 12 = 48 cm
(c) 48 cm
Solution 1(iii)
2x = 3x - 40 … [Pythagoras theorem]
∴ x = 40°
(d) 40°
Solution 2
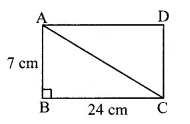
Let ABCD be the rectangle.
AB = 7 cm, BC = 24 cm
In ∆ABC, ∠B = 90° [Angle of a rectangle]
AC² = AB² + BC² [Pythagoras theorem]
= 7² + 24²
= 49 + 576
= 625
AC =![]() [Taking
square root of both sides]
[Taking
square root of both sides]
= 25 cm
∴ The length of the diagonal of the rectangle is 25 cm.
Solution 3
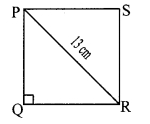
Let PQRS be the square of side x cm.
∴ PQ = QR = x cm …..(i) [Sides of a square]
∴ In ∆PQR, ∠Q = 90° [Angle of a square]
∴ PR² = PQ² + QR² [Pythagoras theorem]
∴ 13 = x + x [From (i)]
∴ 169 = 2x²

Solution 4
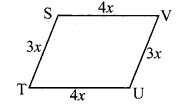
Let STUV be the parallelogram.
Ratio of two adjacent sides of a parallelogram is 3 : 4.
Let the common multiple be x.
ST = 3x cm and TU = 4x cm
∴ ST = UV = 3x cm
TU = SV = 4x cm …..(i) [Opposite sides of a parallelogram]
Perimeter of STUV = 112 [Given]
∴ ST + TU + UV + SV = 112
∴ 3x + 4x + 3x + 4x = 112 [From (i)]
∴ 14x = 112
![]()
∴ x = 8
∴ ST = UV = 3x = 3 x 8 = 24 cm
∴ TU = SV = 4x = 4 x 8 = 32 cm [From (i)]
∴ The lengths of the sides of the parallelogram are 24 cm, 32 cm, 24 cm and 32 cm.
Solution 5
PQRS is a rhombus. [Given]
PR = 20 cm and QS = 48 cm [Given]
∴ PT =![]() PR
[Diagonals of a rhombus bisect each other]
PR
[Diagonals of a rhombus bisect each other]
=![]() × 20 =
10 cm
× 20 =
10 cm
Also, QT =![]() QS
[Diagonals of a rhombus bisect each other]
QS
[Diagonals of a rhombus bisect each other]
=![]() × 48 =
24 cm
× 48 =
24 cm
ii. In ∆PQT, ∠PTQ = 90° [Diagonals of a rhombus are perpendicular to each other]
∴ PQ² = PT² + QT²[Pythagoras- theorem]
= 10²+ 24²
= 100 + 576
∴ PQ2= 676
∴ PQ =![]() [Taking
square root of both sides]
[Taking
square root of both sides]
= 26 cm
∴ The length of side PQ is 26 cm.
Solution 6
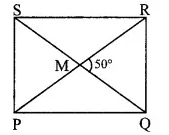
PQRS is a rectangle.
∴ PM =![]() PR …(i)
PR …(i)
MS =![]() QS …(ii)
[Diagonals of a rectangle bisect each other]
QS …(ii)
[Diagonals of a rectangle bisect each other]
Also, PR = QS …..(iii) [Diagonals of a rectangle are congruent]
∴ PM = MS ….(iv) [From (i), (ii) and (iii)]
In ∆PMS,
PM = MS [From (iv)]
∴ ∠MSP = ∠MPS = x° …..(v) [Isosceles triangle theorem]
∠PMS = ∠QMR = 50° ……(vi) [Vertically opposite angles]
In ∆MPS,
∠PMS + ∠MPS + ∠MSP = 180° [Sum of the measures of the angles of a triangle is 180°]
∴ 50° + x + x = 180° [From (v) and (vi)]
∴ 50° + 2x= 180
∴ 2x= 180-50
∴ 2x= 130
∴ x =![]() = 65°
= 65°
∴ ∠MPS = 65° [From (v)]
Solution 7
Solution:
Given: seg AB || seg PQ , seg AB ≅ seg PQ,
seg AC || seg PR, seg AC ≅ seg PR
To prove: seg BC || seg QR, seg BC ≅ seg QR
Proof:
Consider □ABQP,
seg AB || seg PQ [Given]
seg AB ≅ seg PQ [Given]
∴ ABQP is a parallelogram. [A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent]
∴ seg AP || seg BQ …..(i)
∴ seg AP ≅ seg BQ …..(ii) [Opposite sides of a parallelogram]
Consider □ACRP,
seg AC || seg PR [Given]
seg AC ≅ seg PR [Given]
∴ ACRP is a parallelogram. [A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent]
∴ seg AP || seg CR …(iii)
∴ seg AP ≅ seg CR …….(iv) [Opposite sides of a parallelogram]
Consider □BCRQ,
seg BQ || seg CR
seg BQ ≅ seg CR
∴ BCRQ is a parallelogram. [A quadrilateral is a parallelogram if a pair of its opposite sides is parallel and congruent]
∴ seg BC || seg QR
∴ seg BC ≅ seg QR [Opposite sides of a parallelogram]
Solution 8

Given : ABCD is a trapezium.
To prove:
Construction: Join points A and Q. Extend seg AQ and let it meet produced DC at R.
Proof:
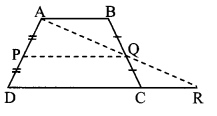
seg AB || seg DC [Given]
and seg BC is their transversal.
∴ ∠ABC ≅ ∠RCB [Alternate angles]
∴ ∠ABQ ≅ ∠RCQ ….(i) [B-Q-C]
In ∆ABQ and ∆RCQ,
∠ABQ ≅ ∠RCQ [From (i)]
seg BQ ≅ seg CQ [Q is the midpoint of seg BC]
∠BQA ≅ ∠CQR [Vertically opposite angles]
∴ ∆ABQ ≅ ∆RCQ [ASA test]
seg AB ≅ seg CR …(ii) [c. s. c. t.]
seg AQ ≅ seg RQ [c. s. c. t.]
∴ Q is the midpoint of seg AR. ….(iii)
In ∆ADR,
Points P and Q are the midpoints of seg AD and seg AR respectively. [Given and from (iii)]
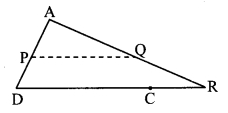
∴ seg PQ || seg DR [Midpoint theorem]
i.e. seg PQ || seg DC ……..(iv) [D-C-R]
But, seg AB || seg DC …….(v) [Given]
∴ seg PQ || seg AB [From (iv) and (v)]
In ∆ADR,
PQ =![]() DR [Midpoint
Theorem]
DR [Midpoint
Theorem]
=![]() (DC +CR)
[D-C-R]
(DC +CR)
[D-C-R]
=![]() (DC + AB)
[From (iii)]
(DC + AB)
[From (iii)]
PQ =![]() (AB + DC)
(AB + DC)
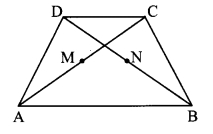
Solution 9
Solution:
Given: ABCD is a trapezium. AB || DC.
Points M and N are midpoints of diagonals AC and DB respectively.
To prove: MN || AB
Construction: Join D and M. Extend seg DM to meet seg AB at point E such that A-E-B.
Proof:
seg AB || seg DC and seg AC is their transversal. [Given]
∴ ∠CAB ≅ ∠ACD [Alternate angles]
∴ ∠MAE ≅ ∠MCD ….(i) [C-M-A, A-E-B]
In ∆AME and ∆CMD,
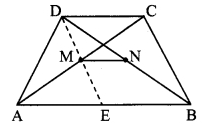
∠AME ≅ ∠CMD [Vertically opposite angles]
seg AM ≅ seg CM [M is the midpoint of seg AC]
∠MAE ≅ ∠MCD [From (i)]
∴ ∆AME ≅ ∆CMD [ASA test]
∴ seg ME ≅ seg MD [c.s.c.t]
∴ Point M is the midpoint of seg DE. …(ii)
In ∆DEB,
Points M and N are the midpoints of seg DE and seg DB respectively. [Given and from (ii)]
∴ seg MN || seg EB [Midpoint theorem]
∴ seg MN || seg AB [A-E-B]

