Class 8 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 7: Variation

(1)

Circumference (c) of a circle is directly proportional to its radius (r).

This can be written in symbol of variation as "c α r"

(2)

Consumption of petrol (l) in a car and distance travelled by that car (d) are in direct variation.

This can be written in symbol of variation as "l α d"

The cost of apples and their number are in direct variation i.e. x α y

∴ x = ky. where k is a constant of variation

Given in table, x = 1 and y = 8

Since, x = ky

∴ 1 = 8k

∴  

Hence, equation of variation is  

When y = 56

∴ when y = 56 then x = 7

When x = 12  

∴ y = 96

∴ When x = 12 then y = 96

When y = 160

∴ x = 20

When y = 160 then x = 20

Given that m α n

i.e. m = kn, where k is a constant of variation

Also, when m = 154, then n = 7

∴ 154 = 7k

∴ k = 22

So, the equation of variation is m = 22n

Now, when n = 14 and m = 22n

∴ m = 22 × 14 = 308

It is given that n varies directly as m i.e. n α m

So, n = km where k is a constant of variation.

Now, it is given that when m = 3 then n = 12

∴ 12 = 3k

∴ k = 4

Thus, the equation of variation is n = 4m

When m = 6.5, we need to find n.

Since, n = 4m

∴ n = 4 × 6.5 = 26.0

∴ when m = 6.5 then n = 26

When n = 28, we need to find m.

Since, n = 4m

∴ 28 = 4m

∴ m = 7

∴ when n = 28 then m = 7

When m = 1.25, we need to find n

Since, n = 4m

∴ n = 4 × 1.25 = 5

∴ when m = 1.25 then n = 5

It is given that y varies directly as square root of x.

∴ y α  

 where k is a constant of variation.

Now, when x = 16, then y = 24

∴ 24 = 4k

∴ k = 6

∴ Equation of variation is  and constant of variation is k = 6.

Let the total remuneration paid to labourers be Rs. y and x be the number of labourers.

It is given that y is in direct variation with x.

∴ y α x

∴ Equation of variation is y = kx, where k is a constant of variation.

Now, remuneration of 4 labours is Rs. 1000

i.e. when x = 4, then y = 1000

Since, y = kx

∴ 1000 = 4k

∴ k = 250

∴ Equation of variation is y = 250x

Now, we need to find the remuneration of 17 labourers

i.e. to find y when x = 17

Since, y = 250x

∴ y = 250 × 17 = Rs. 4250

Thus, the remuneration of 17 labourers is Rs. 4250.

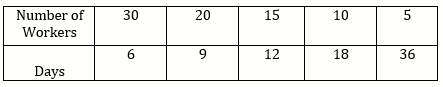
Let the number of workers be x and number of days be y.

The number of workers is in inverse variation with number of days.

∴ xy = k

(i)

Now, when x = 20 then y = 9

∴ k = 20 × 9 = 180

So, the equation of variation is xy = 180

(ii)

If y = 12, x = ?

Since, xy = 180

∴ 12x = 180

∴ x = 15

∴ when y = 12 then x = 10

(iii)

If x = 10, y = ?

Since, xy = 180

∴ 10y = 180

∴ y = 18

(iv)

If y = 36 then x = ?

Since, xy = 180

∴ 36x = 180

∴ x = 5

(1)

Given: p α  

∴ , where k is a constant of variation

∴ pq = k

If p = 15 and q = 4

∴ 15 × 4 = k

∴ k = 60

Thus, the equation of variation is pq = 60.

(2) z α  

∴  where k is a constant of variation

∴ zw = k

If z = 2.5 and w = 24 then

∴ 2.5 × 24 = k

∴ k = 60

∴ The equation of variation is zw = 60.

(3)  

∴  where k is a constant of variation

∴ st² = k

If s = 4 and t = 5 then

∴ 4 × 5² = k

∴ k = 100

∴ The equation of variation is st² = 100

(4)  

∴  

If x = 15 and y = 9

∴ 15 × √9 = k

∴ k = 15 × 3 = 45

∴ The equation of variation is 5

Let the number of apples in the boxes be x and number of boxes be y.

The number of apples in the boxes is in indirect variation with number of boxes.

∴  where k is a constant of variation

∴ xy = k

When x = 24 then y = 27

∴ 24 × 27 = k

∴ k = 648

∴ The equation of variation is xy = 648

When x = 36 then y = ?

Since, xy = 648

∴ 36y = 648

∴ y = 18

Thus, when 36 apples are filled in a box then 18 boxes will be needed.

(1)

It is given that wavelength of sound (l) and its frequency (f) has inverse variation.

, where k is a constant of variation.

∴  

(2)

It is given that intensity (I) of light varies inversely with the square of the distance (d) of a screen from the lamp.

∴ , where k is a constant of variation.

∴ Id² = k

It is given that x α  

∴ x =  where k is a constant of variation.

∴ x = k

When x = 40 then y = 16

∴ 40 ×  = k

∴ k = 40 × 4 = 160

Thus, the equation of variation is x = 160.

If x = 10 then y = ?

Since, x = 160

∴ 10 = 160

∴  = 16

∴ y = 16² = 256

Given that x varies inversely as y i.e.  

∴  where k is constant of variation

∴ xy = k

When x = 15 then y = 10

∴ 15 × 10 = k

∴ k = 150

Thus, the equation of variation is xy = 150

If x = 20 then y = ?

Since, xy = 150

∴ 20y = 150

∴ y = 7.5

(1)

Let the number of workers on a job be x and time taken by them to complete the job is y.

As the number of workers will increase the time taken by them to complete the job will decrease.

∴ xy = k, where k is constant of variation.

∴ This statement has inverse variation.

(2)

Let the number of pipes required to fill a tank be x and the time taken by them to fill the tank be y.

Now, when the number of pipes of same size will increase then the time taken by them to fill the tank will decrease.

∴ xy = k, where k is constant of variation.

∴ This statement has inverse variation.

(3)

Let the petrol filled in the tank of a vehicle be x liters and its cost be Rs. y.

As the petrol filled in the tank of a vehicle will increase, then its cost will also increase.

 where k is constant of variation.

∴ This statement has direct variation.

(4)

Let the area of circle be A and its radius be r.

As the radius of a circle increases, then its area will also increase.

 where k is constant of variation.

∴ This statement has direct variation.

Let the number of workers required to build a wall be x and the time taken by them to build a wall be y.

Here, the number of workers required to build a wall is inversely proportional to the time taken by them.

∴ xy = k, where k is constant of variation

If x = 15, then y = 48

∴ k = 15 × 48 = 720

∴ The equation of variation is xy = 720.

When y = 30

∴ 30x = 720

∴ x = 24

∴ 24 workers required to build a wall in 30 hours.

Let the number of bags be x and the time required to fill the bags be y.

Here, the number of bags will be directly proportional to the time required to fill the bags.

∴ x α y

∴ x = ky

If x = 120, then y = 3

∴ 3k = 120

∴ k = 40

∴ Equation of variation is x = 40y

Now, we need to find the time which is required to fill 1800 bags i.e. when x = 1800

If x = 1800

∴ 1800 = 40y

∴ y = 45

∴ The time required to fill 1800 bags is 45 minutes.

Let the speed of car be x km/hr and y hour be the time taken to travel.

As speed of a car will increase then time taken to travel by the car will decrease.

∴ xy = k, where k is the constant of variation

A car with speed 60 km/hr takes 8 hours to travel some distance.

i.e. if x = 60 km/hr, then y = 8 hours

∴ k = 60 × 8 = 480

∴ Equation of variation is xy = 480

When y = 7.5 hours

∴ 7.5y = 480

∴ y = 64

∴ Speed of the car should be 64 km/hr to cover the same distance in hours.

∴ Increase in speed should be 64 - 60 = 4 km/hr