Class 8 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 8: Quadrilateral : Constructions and Types
Quadrilateral : Constructions and Types Exercise Ex. 8.1
Solution 1
Rough diagram
![]()
Steps of Construction:
- Draw line OR = 4.4 cm.
- Draw a ray using vertex O and making an angle of 105˚.
- Draw a ray at R making an angle of 90˚.
- Taking O as the centre and radius 5.8 cm, draw an arc to cut on the ray at point M.
- Draw an angle of 58˚ at point M, intersecting the ray drawn from R. Name the point of intersection as E.
- □MORE is the required quadrilateral.
![]()
Solution 2
Rough diagram
![]()
Steps of construction:
- Draw seg DE = 4.5 cm
- Draw an arc with centre D and radius 7.2 cm.
- With centre E and radius 6.5 cm, draw another arc cutting the first arc and name the point of intersection as F.
- Draw seg DF and EF.
- With centre E and radius 7.8 cm, draw an arc on the opposite side of F.
- With centre D and radius 5.5 cm, draw an arc cutting the previous arc at point G.
- Draw seg EG and DG.
- □ DEFG is required quadrilateral.
![]()
Solution 3
Rough Diagram
![]()
Steps of Construction:
- Draw seg BC = 4.8 cm.
- Draw a ray at point B making an angle of 50˚.
- Draw a ray at point C making an angle of 140˚
- Taking B as centre and radius 6.4 cm, draw an arc to cut the ray (drawn from B) at point A.
- Draw a ray at point A making an angle of 70˚ to cut the ray drawn from C and name the point of intersection as D.
- □ ABCD is required quadrilateral.
![]()
Solution 4
Rough diagram
![]()
Steps of construction:
- Draw seg MO = 7.5 cm
- Taking O as centre and radius 6 cm, draw an arc on one side of OM.
- With centre M and radius 6 cm, draw an arc to cut the previous arc and name the point of intersection as L.
- Again taking O as centre and radius 4.5 cm, draw an arc on the other side of OM.
- With centre M and radius 4.5 cm, draw an arc cutting the previous arc (drawn in step 4) at point N.
- □ LMNO is required quadrilateral.
![]()
Quadrilateral : Constructions and Types Exercise Ex. 8.2
Solution 1
Rough Diagram:
![]()
Steps of Constructions:
1. Draw seg AB = 6 cm
2. Draw a ray AX from A making an angle of 90o.
3. Draw another ray BY from B making an angle of 90o.
4. With centre A and radius 4.5 cm, draw an arc cutting AX at D.
5. With centre B and radius 4.5 cm, drawn an arc cutting BY at C.
6. Draw seg DC.
7. ABCD is the required rectangle.
![]()
Solution 2
Rough diagram:
![]()
Steps of construction:
- Draw seg WX = 5.2 cm.
- Draw two rays WP and XQ from points W and X respectively making an angle of 90o each.
- Draw two arcs each with centres W and X and radius 5.2 cm intersecting the rays WP and XQ at Z and Y respectively.
- Join Y and Z.
- □ WXYZ is the required quadrilateral.
![]()
Solution 3
Rough diagram:
![]()
Steps of construction:
- Draw seg KL = 4 cm.
- Draw a ray KX at vertex K making an angle of 75˚ and a ray LY at L making an angle of 105˚.
- Taking K and L as centers with same radius 4 cm, draw two arcs to cut the rays KX and LY at N and M respectively.
- Join N and M.
- □ KLMN is a required quadrilateral.
![]()
Solution 4
![]()
From the diagram, ∆DAB is a right angled triangle.
Using Pythagoras theorem, we have
DA2 + AB2 = DB2
∴ 242 + AB2 = 262
∴ 576 + AB2 = 676
∴ AB2 = 676 - 576 = 100
∴ AB = 10 cm
Hence, the other side of rectangle is 10 cm.
Solution 5
![]()
The diagonals of a rhombus are perpendicular bisector of each other.
As AC = 16 cm and BD = 12 cm,
OA = OC = ![]() = 8 cm
= 8 cm
OB = OD = ![]() = 6 cm
= 6 cm
∆AOB is a right angled triangle at O.
Using Pythagoras theorem, we have
AO2 + BO2 = AB2
∴ AB2 = 82 + 62 = 64 + 36 = 100
∴ AB = 10 cm
∴ The side of a rhombus is 10 cm and perimeter = 4 × 10 = 40 cm.
Solution 6
![]()
Let us consider, square ABCD
Here, AB = BC = CD = AD = 8 cm
The diagonals of a square are perpendicular bisector of each other.
In right angled ∆ABC,
AC2 = AB2 + BC2
∴ AC2 = 82 + 82 = 64 + 64 = 128
∴ AC = ![]() cm
cm
Hence, the
diagonal of a square is ![]() cm.
cm.
Solution 7
![]()
Let ABCD be a rhombus.
The opposite angles of a rhombus are equal.
∴ ∠B = ∠D = 50˚
The adjacent angles of a rhombus are supplementary.
∴ ∠B + ∠C = 180˚
∴ 50˚ + ∠ C = 180˚
∴ ∠ C = 180˚ - 50˚ = 130˚
∠ C = ∠ A = 130˚
The remaining angles of a rhombus are 50˚, 130˚, 130˚.
Quadrilateral : Constructions and Types Exercise Ex. 8.3
Solution 1
Let the parallelogram be PQRS where ∠P = (3x - 2)° and ∠R = (50 - x)° are one pair of opposite angles.
The other pair of opposite angles be ∠Q and ∠S.
By the property of parallelogram, opposite angles of a parallelogram are congruent, we have
∠P = ∠R
∴ 3x - 2 = 50 - x
∴ 3x + x = 50 + 2
∴ 4x = 52
∴ x = 13
∴ ∠P = (50 - x)° = (50 - 13)° = 37°
∴ ∠R = 37°
As adjacent angles of a parallelogram are supplementary.
∴ ∠P + ∠Q = 180°
∴ 37° + ∠Q = 180°
∴ ∠Q = 180° - 37° = 143°
∴ ∠S = 143°
∴ The measures of the angles of the parallelogram are 37°, 143°, 37° and 143°.
Solution 2
In parallelogram XYZW,
(1)
Side XY = side WZ = 4.5 cm … Opposite sides of parallelogram
(2)
Side XW = side YZ = 8.2 cm … Opposite sides of parallelogram
(3)
Length OZ = Length OX = 2.5 cm … Diagonals bisect each other in parallelogram
(4)
Since, Diagonals bisect each other in parallelogram
∴ Length WY = 2 × length of WO = 2 × 3.3 = 6.6 cm
(5)
∠ WXY = ∠ WZY = 120˚ … Opposite angles are congruent in parallelogram
∠ XWY + ∠ WZY = 180˚ … Adjacent angles are supplementary
∴ ∠ XWZ + 120˚ = 180˚
∴ ∠ XWZ = 180˚ - 120˚
∴ ∠ XWZ = 60˚
Solution 3
Rough diagram:
![]()
Steps of Construction:
1. Draw seg BC = 7 cm.
2. Draw ∠B = 40˚ and B and C are supplementary angles of parallelogram.
∴ ∠B + ∠C = 180˚
∴ 40˚ + ∠C = 180˚
∴ ∠C = 180˚- 40˚
∴ ∠C = 140˚
3. From vertex C, draw a ray of an angle 140˚.
4. With center B and C with radius 3 cm. Cut the ray and name the points A and D respectively.
5. Join points A and D.
6. □ ABCD is a parallelogram.
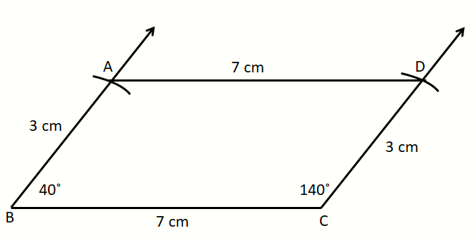
Solution 4
Let □ PQRS be the quadrilateral and given that their angles are in the ratio 1:2:3:4.
Let the angles be x, 2x, 3x, 4x.
The sum of all the angles in a quadrilateral is 360˚.
∴ x + 2x + 3x + 4x = 360˚
∴ 10x = 360˚
∴ x = 36˚
Hence, ∠ P = 36˚, ∠ Q = 2x = 72˚, ∠ R = 3x = 108˚, ∠S = 4x = 144˚
Angles P and S are supplementary, angles Q and R are supplementary.
Hence, side PQ || side RS.
Only one pair of side is parallel hence, □PQRS is a trapezium.
Solution 5
Rough Diagram:
![]()
Steps of Constructions:
- Draw seg AC which is diagonal of a quadrilateral BARC.
- Taking centre A and C with radius 4.2 cm. Draw two arcs on one side of seg AC. Point B is the intersection point. Join B, C and B, A.
- Taking centre A and C with radius 5.6 cm. Draw two arcs on the other side of seg AC. Point R is the intersection point.
- Join R, C and A, R.
- □BARC is the required quadrilateral.
![]()
Solution 6
Rough diagram:
![]()
Steps of Construction:
1. Draw seg QR = 5.6 cm.
2. Draw ray at vertex Q of an angle 110˚ = ∠ PQR.
3. Draw ray at vertex R of an angle 70˚ = ∠ SRQ.
4. With centre Q of radius 3.5 cm. Draw an arc to cut the ray and name the point of intersections as P.
5. With centre R of radius 3.5 cm. Draw an arc to cut the ray and name the point of intersections as S.
6. □ PQRS is a required quadrilateral.
PQ = 3.5 cm this information is unnecessary because opposite sides of a parallelogram are congruent.
![]()
