Class 8 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 19: Miscellaneous Excercise 2
Miscellaneous Excercise 2 Exercise Misc. Ex. 2
Solution 1(1)
(B)
Area of a circle = 1386 cm2
∴ πr2 = 1386
∴ 
r2 = 1386
∴ r2 =
1386 × 
= 441
∴ r = 21 cm
Circumference = 2πr
= 2 × 
×
21
= 44 × 3
= 132 cm
Solution 1(2)
(D)
The side of a cube is 4 m.
When the side is doubled, new side will be 8 m.
∴ Volume of original cube i.e. cube of side 4 m is 43 = 64
⇒ Volume of new cube i.e. cube of side 8 m is 83 = 512
Now, we know that 512 = 64 × 8
Hence, volume of new cube will be eight times the volume of original cube.
Solution 2
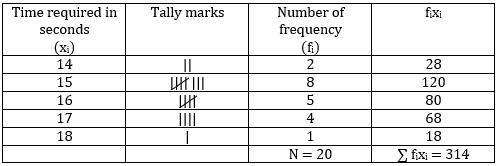
Mean = 
= 
= 15.7 seconds
Solution 3
Given that ∆EDF ≅ ∆ LMN
Congruent sides
Side DE = side LM
Side DF = side MN
Side EF = side LN
Congruent angles
∠ E = ∠ L
∠ D = ∠ M
∠ F = ∠ N
Solution 4
P = Original cost of machine = Rs. 2,50,000
R = rate of depreciation = -4% per annum
N = Number of years = 3 years
The cost of machine after 3 years
A = P(1 - 
N
A = 2,50,000 (1 - 
)3
A = 2,50,000 (1 - 
)3
A = 2,50,000 × 
× ×
A = 16 × 24 × 24 × 24
A = Rs. 221184
The cost of machine after the 3 years is Rs. 2,21,184.
Solution 5
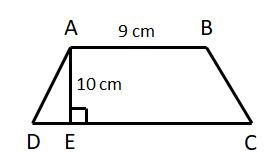
□ ABCD is a trapezium as opposite sides AB and CD are parallel.
Area of □ ABCD = 
× sum of lengths of parallel sides × height
∴ 115 = × (AB + CD) × AE
∴ 115 = × (9 + CD) × 10
∴ 
=
9 + CD
∴ CD = 23 - 9 = 14 cm
Solution 6
For cylindrical tank:
d = 1.75 m then r =
m
h = 3.2 m
Volume of cylinder = πr2h
= ×
× × 3.2
= 11 × 0.25 × 1.75 × 1.6
= 7.7 m3
= 7.7 × 1000 litre
= 7700 litre
The capacity of tank is 7700 litre.
Solution 7
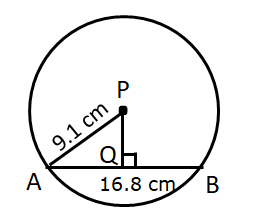
Above diagram shows the given information, where P is the centre and AB is the chord of a circle.
∴ AB = 16.8 cm and PA = 9.1 cm
∴ PQ ⟂ AB and AQ =
BQ = 
= 8.4 cm
In right angled ∆PQA, using Pythagoras theorem, we have
PQ2 + AQ2 = PA2
∴ PQ2 = PA2 - AQ2
= (9.1)2 - (8.4)2
= 82.81 - 70.56
= 12.25
∴ PQ = 3.5 cm
Hence, the distance from the centre of the circle to the chord is 3.5 cm.
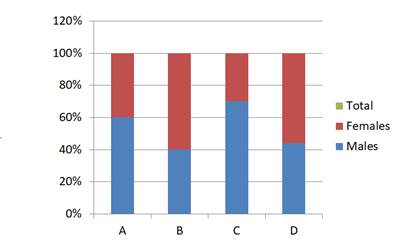
Solution 8
(1)
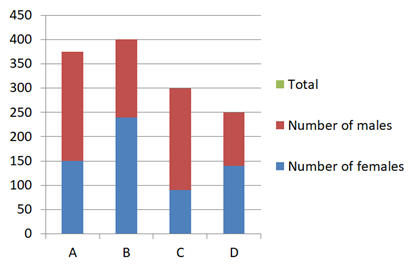
(2)
Percentage of males
in village A = 
×
100 = 
×
100 = 60%
Percentage of males
in village B = 
×
100 = 
×
100 = 40%
Percentage of males
in village C = 
×
100 = 
×
100 = 70%
Percentage of males
in village D = 
×
100 = 
×
100 = 44%
Solution 9(1)
17(x + 4) + 8(x + 6) = 11(x + 5) + 15(x + 3)
∴ 17x + 68 + 8x + 48 = 11x + 55 + 15x + 45
∴ 17x + 8x + 68 + 48 = 11x + 15x + 55 + 45
∴ 25x + 116 = 26x + 100
∴ 116 - 100 = 26x - 25x
∴ x = 16
Solution 9(2)
∴ 12y = 2(18 - 2y)
∴ 12y = 36 - 4y
∴ 12y + 4y = 36
∴ 16y = 36
Solution 9(3)
5(1 - 2x) = 9(1 - x)
∴ 5 - 10x = 9 - 9x
∴ 5 - 9 = 10x - 9x
∴ -4 = x
Solution 10
(1)
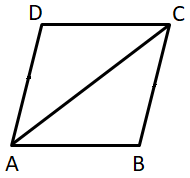
(2)
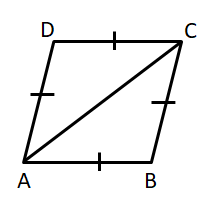
(3)
∆ADC ≅ ∆ABC
As all sides in triangle ADC and ABC are congruent then they are congruent by SSS test.
(4)
∆ADC ≅ ∆ABC
As all sides in triangle ADC and ABC are congruent then they are congruent by SSS test.
Hence, ∠DCA ≅∠BCA, and ∠DAC ≅ ∠BAC
(5)
Diagonal of a rhombus bisect its opposite angles.
Solution 11
In ∆PQR,
Let PR = a = 260 m, QR = b = 250 m, PQ = c = 170 m
Semi-perimeter = 
= 
= = 
= 340 m
By Heron's formula
Area of ∆ PQR = 
= 
= 
= 
= 
= 17 × 2 × 2 × 100 × 3
= 20400 sq. m
In ∆PSR,
PR = a = 260 m, PS = b = 240 m, RS = c = 100
Semi-perimeter = = 
= 
= 300 m
By Heron's formula
Area of ∆ RPQ =
= 
= 
= 
= 
= 100 × 3 × 4 × 10
= 12,000 sq. m
Area of farm = Area of ∆ PSR + Area of ∆ RPQ
= 12,000 + 20,400
= 32400 sq. m
1 hectare = 10, 000 sq. m
Area of farm = 
= 3.24 hectare
Solution 12
Let the total number of books in the library be x.
50% of total number of books is of Marathi i.e. 50% of x =
The books of English are 1/3rd of Marathi books i.e. 
= 
The books on mathematics are 25% of the English books i.e.
= 
Remaining books are 560.
Hence,
+ +
+ 560 = x
∴ 
+ 560 = x
∴ 
+ 560 = x
∴ 560 = x -
∴ 
= 560
∴ 
= 560
∴ x = 
= 1920
The total books in the library are 1920.
Solution 13
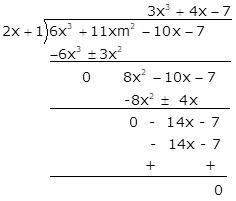
∴ Quotient = 3x2 + 4x - 7 and remainder = 0