Class 8 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 14: Compound interest
Compound interest Exercise Ex. 14.1
Solution 1
(1)
P = Rs. 2000, R = 5 p.c.p.a. N = 2 years
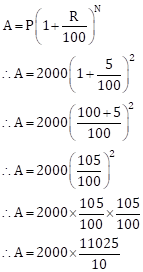
∴ A = Rs. 2205
CI = A - P
= 2205 - 2000
= Rs. 205
(2)
P = Rs. 5000, R = 8 p.c.p.a., N = 3 years
∴ A= 6298.56
CI = A - P
= 6298.56 - 5000
= Rs. 1298.56
(3)
P = Rs. 4000, R = 7.5 p.c.p.a., N = 2 years
∴ A = Rs. 4622.5
CI = A - P
= 4622.5 - 4000
= Rs. 622.5
Solution 2
Sameerrao has taken a loan of Rs. 12500 i.e. P = Rs. 12,500
Rate of 12 p.c.p.a i.e. R = 12 p.c.p.a.
For 3 years i.e. N = 3 years
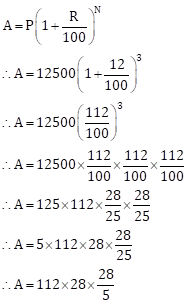
∴ A = Rs. 17561.6
Sameerrao should pay Rs. 17561.6 to clear his loan amount.
Solution 3
Shalaka has taken a loan of Rs.8000 i.e. P = Rs. 8000
at
a rate of 10
p.c.p.a.
i.e. R = 10.5 p.c.p.a
After two years i.e. N = 2 years
∴ A = Rs. 9768.2
CI = A - P
= 9768.2 - 8000
= Rs. 1768.2
Shalaka will have to pay Rs. 1768.2 as compound interest.
Compound interest Exercise Ex. 14.2
Solution 1
P = initial number of workers = 320,
R = rate of interest = 25 p.c.p.a.,
N = number of years = 2 years
∴ 
∴ 
∴ 
∴ 
∴ 
∴ A= 20 × 25
∴ A = 500
The number of workers after 2 years is 500.
Solution 2
P = Number of sheep initially = 200
N = 3 years
R = 8% every year
∴ 
∴ 
∴ 
∴ 
∴ 
∴ 
∴ A = 251.9424
As number of sheep must be an integer, it should be 252.
Solution 3
P = number of trees = 40,000
N = 3 years
R = 5% per year
∴
∴
∴
∴
∴
∴
∴ A = 46305
The expected number of trees after 3 years at the rate of 25% every year is 46,305.
Solution 4
P = cost of machine = Rs. 2,50,000
R = 10% per year
N = 2 years
The rate of depreciation means the rate is decreasing of a machine.
∴
∴
∴
∴
∴
∴ A = 202500
Depreciation = P - A
= 2,50,000 - 2,02, 500
= 47,500
The cost of machine after two years will be Rs. 47,500.
Solution 5
P = ?
N = 2 years
A = Rs. 4036.80
R = 16 p.c.p.a.
∴
∴
∴
P = 
∴ P = 3000
CI = A - P
= 4036.80 - 3000
= Rs. 1036.80
The compound interest is Rs. 1036.80.
Solution 6
P = Rs. 15,000
R = 12 p.c.p.a.
N = 3 years
∴
∴
∴
∴
A = 
∴ A = 21073.92
The amount to settle the loan after 3 years is Rs. 21073.92
Solution 7
A = Rs. 13924
N = 2 years
R = 18 p.c.p.a.
∴
∴
∴
∴
P = 13924 
∴ P = 10000
The principal is Rs. 10,000.
Solution 8
P = 16,000
N = 2 years
R =?
A = 17640
∴
∴
∴
∴ 
Taking square root on both sides
∴
∴
= 
∴ R = 5%
Rate of interest of population is 5% every year.
Solution 9
P = Rs. 700
A = Rs. 847
R = 10 p.c.p.a.
N =?
0
∴
∴
∴
∴
We know that 112 = 121 and 102 = 100
Hence, 700 amounts to 847 in 2 years.
Solution 10
P = Rs. 20,000
R = 8 p.c.p.a.
N = 2 years
Simple
interest = P × N ×
=
P× 2 ×
=
….(i)
CI = A - P
∴
∴
∴
∴
……(ii)
CI - SI
=
=
=
= 2 × 8 × 8
= 128
The difference between simple interest and compound interest is Rs. 128.