Class 10 MAHARASHTRA STATE TEXTBOOK BUREAU Solutions Maths Chapter 7 - Mensuration

Given:

Radius, r = 1.5 cm

Height, h = 5 cm

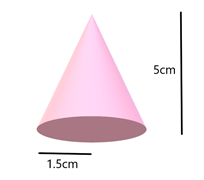
The volume of a cone

V = 𝜋 × 0.75 × 5

V = 3.75 × 3.14

V = 11.77 cm²

The volume of the cone is 11.77 cm².

Given,

Diameter of the sphere,

Therefore, radius (r)

r = 3 cm

Volume of sphere

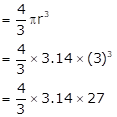
= 113.04 cm³

Volume of sphere is 113.04 cm³

Given,

Radius (r) of cylinder's base is 5cm

Height (h) of the cylinder is 40cm

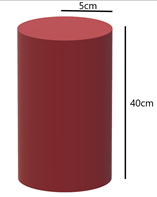
Thus,

Total surface area of the cylinder

TSA = 2𝜋rh + 2𝜋r²

TSA = 2𝜋r(h + r)

TSA = 2𝜋(5)(40 + 5)

TSA = 2𝜋(5)(45)

TSA = 2 × 3.14 × 5 × 45

TSA = 1413 cm²

The total surface area of the cylinder, TSA = 1413 cm²

Given;

Radius of the sphere, r=7cm

We have to find the surface area of the sphere.

SA = 4𝜋r²

SA = 616 cm²

The surface area of the sphere is 616 cm²

Given:

Dimensions of cuboid 44 cm × 21 cm × 12 cm 

Cuboid is melted in to a cone.

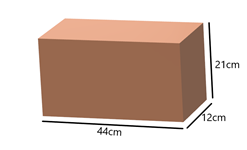
We have to radius of the cone.

Their volume will be equal thus,

V_cuboid = V_cone

r = 21 cm

The radius of the cone is 21cm.

Given:

Height of conical jug h_c = 10cm

Radius of the conical jug r_c = 3.5 cm

Height of cylindrical pot h_cy = 10cm

Radius of cylindrical pot r_cy = 7cm

We have to find the number of jugs of water the cylindrical pot hold.

Let the number of jugs be 'n'

V_cy = nV_c 

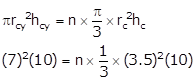
n = 12

The cylindrical pot can hold up to 12 jugs of conical jugs of water. 

Given:

Height of the cylinder, h_cy = 3 cm  

Area of base, A = 100 cm²

Volume of the solid figure, V_total = 500 cm³= 10cm  

As we can see the radii of the cone and cylinder are the same, therefore their base areas are also same.

We have to find the total height i.e., h_total = h_cy + h_c

V_total = V_cy + V_c 

h_c = 6 cm 

h_total = h_cy + h_c

h_total = 3 + 6

h_total = 9cm

The total height of the solid figure is 9 cm.

Given,

Radius,

Height of cone,

Height of cylinder,

We have to find the total area of the toy.

SA = SA_hemisphere+ SA_cylinder + SA_cone

SA = 2𝜋r² + 2𝜋r_cy +

SA = 2𝜋3² + 2𝜋(3)(40) + 𝜋(3)√4² + 3²

SA = 𝜋(18 + 240 + 15)

SA = 858 cm²

The surface area of the toy is 858 cm²

Given;

Radius of tablet, r_t = 7mm

Thickness of tablet, t = 5mm

Radius of wrapper,

Length of wrapper, l = 10 cm = 100 mm

We have to find the number of tablets wrapped.

Tablet:

Wrapper:

Let the number of tablets be 'n'.

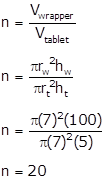
The number of tablets wrapped are 20. 

Given:

Radius, r = 3 cm

Height of the cone, h = 4 cm

We have to find the volume and surface area.

Volume

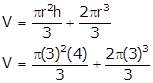
V = 12𝜋 + 18𝜋

V = 30𝜋

V = 94.2 cm³

SA = 2𝜋r² + 𝜋r√h² + r²

SA = 2𝜋(3)² + 𝜋(3)√(4)² + (3)²

SA = 18𝜋 + 15𝜋

SA = 33𝜋

SA = 103.62 cm²

The volume of the toy isand its surface area is 103.62 cm².

Given:

Diameter of ball, d = 42 cm

Radius,

We have to find the surface area and the volume of the ball.

SA = 4𝜋r²

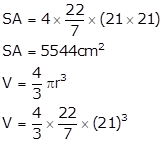
V = 38808 cm³

The surface area of the ball is 5544 cm² and the volume is 38808 cm³.

Given:

Radius of the cylinder,

Height of the water in the cylinder,

Diameter of sphere,

Thus, the radius of the sphere,

The volume of the water is

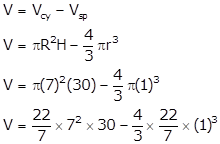
V = 4620 - 4.187

V = 4615.813 cm³.

The volume of water is 4615.813 cm³.

Given:

Radii of circular ends of frustum,

R = 14 cm

r = 7 cm

Height of the bucket, H = 30 cm

We have to find the volume(V) of water (in liters) it can hold.

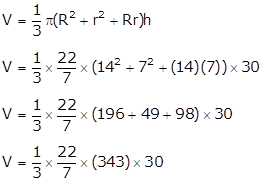
V = 10780 cm³

Volume of water

V = 10.780 Liters

The bucket can hold 10.78L in the bucket.

Given:

Radii of circular ends of frustum,

r = 14 cm

r = 6 cm

Height of the bucket, H = 6 cm

We have to find

(i) Curved surface area 

(ii) Total surface area 

(iii) Volume

The slant height (L),

L = 10 cm

(i)Curves surface area

CSA = 𝜋L(R + r)

CSA = (3.14)(10)(14 + 6)

CSA = (3.14)(10)(20)

CSA = 628 cm²

(ii) Total surface area

TSA = 𝜋(L(R + r) + (R² + r²))

TSA = 3.14()10)(14 + 6) + (14² + 6²))

TSA = 3.14(200 + 232)

TSA = 3.14(432)

TSA = 1356.48cm²

(iii) Volume

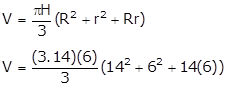
V = 2(3.14)(232 + 84)

V = 1984.48 cm³

The curved surface area, total surface area, and volume are

CSA = 628 cm², TSA = 1356.48 cm² and V = 1984.48 cm² respectively. 

Circumference (Let radius be r₁) = 132 cm

2𝜋r₁ = 132

r₁ = 21 cm

Circumference (Let radius be r₂) = 88 cm

2𝜋r₂ = 88

r₂ = 14 cm

Height of frustum, H = 24 cm

Therefore, slant height (L)

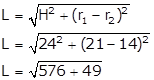
L = √625

L = 25 cm

The curved surface area is

CSA = 𝜋L(r₁ + r₂)

CSA = (3.14)(25)(21 + 14)

CSA = 2669 cm²

Given the radius of the circle is 10cm

The measure of the arc is 54°

We have to find the area of sector associated with the arc

A = 47.1 cm²

The area of the sector is A = 47.1 cm² 

Given;

Radius, r = 18 cm

Measure of arc θ= 80°

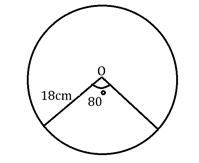
We have to find the length of the arc (L)

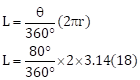
L = 25.12 cm

The length of the arc is 25.12cm.

Given,

Radius, r = 3.5 cm

Length of the arc AB is 2.2cm

We have to find the area of the circle (A)

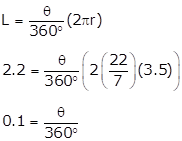
Θ = 36°

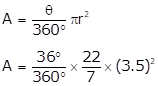
A = 3.85 cm²

The area of the sector OAB is A = 3.85 cm²

Given:

Radius, r = 10 cm

Area of the sector A = 100 cm²

We have to find the area of the major sector

A_major = A_circle - A_minor

A_major = 3.14(10)² - 100

A_major = 314 - 100

A_major = 214 cm²

The area of its corresponding major sector is 214 cm². 

Given;

Radius of circle, r = 15 cm

Area of sector is =30 cm²

We have to find the length of the arc (L)

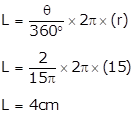
The length of the arc is 4 cm.

Given:

Radius, r = 7 cm

M(arc MON) = 60°

We have to find

(1) Area of circle

(2) A(OMBN)

(3) A(OMCN)

A = 𝜋r²

A =184 cm²

A(OMBN)

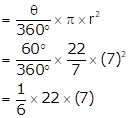
= 30.66 cm²

A(OMCN)

=A - A(OMBN)

= 184 - 30.66

= 153.33cm²

(1) Area of the circle (A), A = 184 cm²

(2) A(OMBN) = 30.66 cm²

(3) A(OMCN) = 153.33 cm²

Given:

Radius of the circle, r = 3.4cm

Perimeter PBAC = 12.8cm

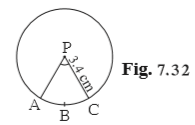
We have to find A(PABC)

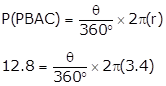
θ=215.81°

A(PABC) = 21.76 cm²

Area of the sector PABC is A(PABC) = 21.76 cm².

Given:

∠ROQ = ∠MON = 60°

OR = 7 cm

OM = 21 cm

We have to find lengths of arc RXQ and arc MYN

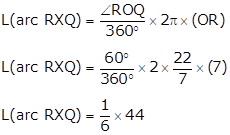
L(arc RXQ) = 7.33 cm

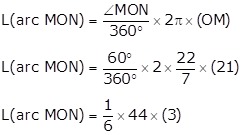
L(arc MON) = 22 cm

The lengths of the arcs are

L(arc RXQ) = 7.33 cm

L(arc MON) = 22 cm

Given:

A(P - ABC) = 154 cm²

Radius, r = 14cm

We have to find:

(1) ∠APC (2) l(arc ABC)

A(P - ABC) = 154 cm²

Θ =90°

Therefore, ∠APC = 90°

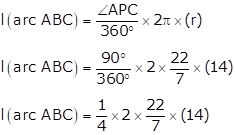
L(arc ABC) = 154 cm

Answer: (1) ∠APC = 90° (2) l(arc ABC) = 154 cm.

Given:

Radius, r = 7 cm

We have to find the area with measure of the arc is

(1)30° (2) 210° (3) Three right angles (270°)

(1)

A = 12.83 cm²

(2)

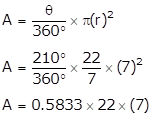
A = 89.83 cm²

(3)

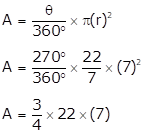
A = 115 cm²

(1) 30°, A = 12.83 cm²

(2) 210°, A = 89.83 cm²

(3) Right angles (90°), A = 115.5 cm²

Given:

Area of a minor sector, A = 3.85 cm²

Central angle θ = 36°

We have to find the radius of the circle

A = 3.85 cm²

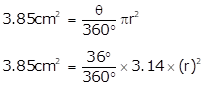
(r)² = 7.33

r = 2.21 cm

The radius of the circle is 2.21cm.

Given;

▭ PQRS is a rectangle

PQ = 14 cm

QR = 21 cm

We have to find the area x, y and z

x = 154 cm²

y = 38.5 cm²

z = A(▭ PQRS) - x - y

z = (14 × 21) - 154 - 38.5

z = 101.5cm²

The area are: x = 154 cm² y = 38.5 cm², z = 101.5 cm².

Given:

ΔLMN is an equilateral triangle

LM = 14 cm

Radius, r = 7 cm

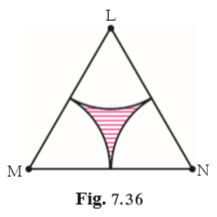
We have to find:

(1) A(ΔLMN)

(2) Area of any one of the sectors.

(3) Total area of all the three sectors.

(4) Area of the shaded region.

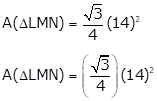
A(ΔLMN) = 84.87 cm²

Area of any one of the sectors

= 25.66 cm²

Total area of all the three sectors

= 3 × Area(sector)

= 3 × 25.66

= 77 cm²

Area of the shaded region

= A(Δ LMN) - 3A(sector)

= 84.87 - 77

= 7.87 cm²

Answer:

(1) A(ΔLMN) = 84.87 cm² 

(2) Area of any one of the sectors = 25.66 cm²

(3) Total area of all the three sectors = 77 cm²

(4) Area of the shaded region = 7.87 cm²

Given:

∠ABC = 45°

AC = 7√2 cm

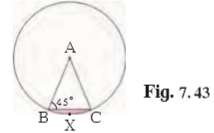
We have to the area of segment BXC

As, AB = AC = r

∠ABC = ∠ACB = 45°

In ΔABC,

∠ABC + ∠ACB + ∠BAC = 180°

45° + 45° + ∠BAC = 180°

∠BAC = 90°

Area of segment BXC (A_segment)

A_segment = A_sector- A_ΔABC

A_segment = 28 cm²

The area of segment BXC is A_segment = 28cm².

Given:

M(arc PQR) = 60°

OP = 10 cm

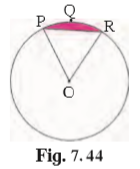
We have to find the area of the shaded region.

A = A(sector OPQR) - A(ΔPQR)

A=52.33 - 25(1.73)

A=9.08cm²

The area of the shaded region is A = 9.08 cm².

Given:

∠PAR = 30°,

AP = 7.5

We have to find the area of the segment PQR.

A = A(sector APQR) - A(ΔAPR)

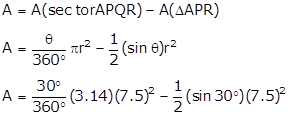
A = 14.718 - 14.0625

A = 0.655 units²

The area of the segment PQR A = 0.655 units². 

Given:

∠POQ = 90°

Area of shaded region = 114 cm² 

We have to find the radius.

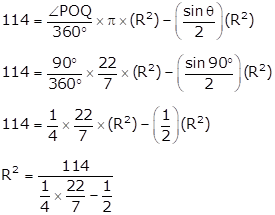
R² = 400

R = 20 cm

The radius of the circle is 20cm.

Given:

Radius, r = 15cm

The angle subtended at the centre, θ=60°

We have to find the area of the minor and major segment

Area of minor segment

= 117.75 - 97.3125

= 20.4375 cm²

Area of major arc

= A(circle) - A(min or arc)

= 𝜋r² - 20.4375

= (3.14)(15)² - 20.4375

= 706 - 20.4375

= 686.0625 cm²

Area of minor segment = 20.4375 cm²

Area of major arc = 868.0625 cm²

Given ratio

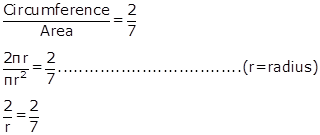
r = 7 units

Thus,

Circumference of the circle

2𝜋r

= 2 × 𝜋 × 7

= 14 𝜋units

(A) 14𝜋

Given:

m(arc)= 160°

L(arc) = 44cm

L(arc) = 44

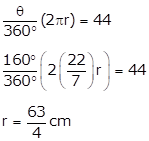
Circumference

= 2𝜋r

= 99 cm

Option (D) 99cm 

Given:

Θ = 90°

R = 7 cm

Perimeter of sector

= 14 + 11

= 25 cm

Option (B) 25cm

Given:

h = 24cm

r = 7cm

CSA = 𝜋rl

CSA = 22(25)

CSA = 550 cm²

Option (B) 550 cm²

Given:

CSA=440cm² 

R=5cm

CSA = 440

2𝜋rh = 440

2𝜋(5)(H) = 440

Option (A)

V_cone = V_cylinder

H_cone = 15 cm

Height of cone is 15cm.

Option (A) 15cm

V_cube = (side)³

V_cube = (0.01)³

V_cube = (1 × 10^-2)³

V_cube = (1 × 10^-6

V_cube = 0.000001 cm³

Volume of cube is 0.000001 cm³

Option (D) 0.000001 cm³

V_cube = (a)³

1 = (a)³

a = 1m

a = 100 cm

The side of cube is 100 cm

Option (C) 100 cm.

Given:

Height, h=21cm

Radius of top and bottom,

r_t = 20 cm

r_b = 15 cm

We have to find the capacity of the tub i.e., volume (in L)

V = 22(400 + 225 + 300)

V = 20350 cm³

V = 20.35 Litre

The capacity of the tub is 20.35 litre. 

Given:

Radius of plastic balls, R = 1 cm

Thickness of tube, t=2cm

Length of tube, l=90cm

Outer radius of tube, r₀ = 30 cm

Therefore, the inner radius, r_i= 28 cm

The volume of the balls will be equal to the volume of the tube.

Let the number of plastic balls melted, be 'n'.

V_balls = V_tube

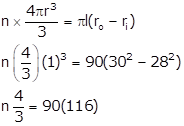
n = 7830

7830 balls were melted to make the tube. 

Given

Dimensions of metal parallel piped =l × b× h= 16 cm × 11cm × 10 cm

Thickness of coins, T=2mm=0.2cm

Diameter of coin, D=2cm

Therefore, the radius of coin, R=1cm

The volume of the metal will be volume of the coins.

Let the number of coins be 'N'

V_metal = V_coins

l × b × h = N × 𝜋R²T

N = 2800

The number of coins formed will be 2800. 

Given;

Diameter of roller, d=120cm

Length of the roller, l=84cm

Rotations required, n=200

We have to find the expenditure at the rate of Rs. 10 per m²

To find the expenditure we have to find the area covered by the roller

A = n × 𝜋dl

A = 6336000 cm²

A = 633.6 m²

Expenditure

= A × rate

= 633.6 × 10

= Rs. 6336

The expenditure to level the ground is Rs. 6336. 

Given;

Diameter of hollow sphere, d=12cm

Radius, r=6cm

Thickness, t=0.01m=1cm

Density, δ = 8.88gm/cm³

We have to find the outer surface area and mass of the hollow sphere

Surface area

= 4𝜋r²

= 4(3.14)(6)²

= 452.16 cm²

Mass

= 3383.16g

The outer surface area is 452.16cm² and mass is 3383.16g of the hollow sphere.

Given;

Diameter of cylindrical bucket, d=28cm

The radius of the cylindrical bucket, r=14cm

Height of cylindrical bucket, h=20cm

Height of the cone, H=14cm

We have to find the base area of the cone

The volume of sand will remain the same therefore,

V_cy = V_cone

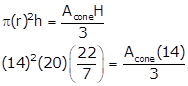
A_cone = 2640 cm²

The base area of the cone is 2640 cm². 

Given:

Radius of sphere, R=9cm

Diameter of wire, d=4mm

Radius of wire, r=1mm=0.2cm

We have to find length of wire

V_sphere = V_wire

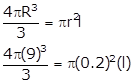
l = 24300 cm

l = 243m

The length of the wire is 243m. 

Given:

Radius of circle, r=6cm

Area of sector, A=15π sq.cm

We have to find measure of the arc, and length of the arc (L).

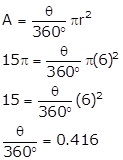
θ = 150°

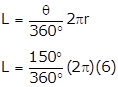
L = 5𝜋cm

The measure of the arc is 150°, and length of the arc is 5𝜋cm. 

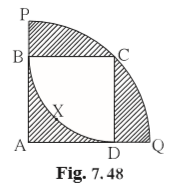
Side of square ABCD = radius of sector C-BXD = 20cm

Area of square = (side)² = 20² = 400 cm²

Area of shaded region inside the square

= Area of square ABCD - Area of sector C-BXD

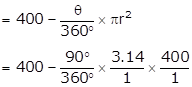
= 400 - 314

= 86 cm²

Radius of bigger sector = Length of diagonal of square ABCD

 = 20√2

Area of the shaded regions outside the square

= Area of sector A-PCQ - Area of square ABCD

=A(A - PCQ) - A(▭ABCD)

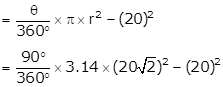
= 628 - 400

= 228 cm²

total area of the shaded region = 86 + 228 = 314 cm².

Let the radius of the bigger circle be R and that of smaller circle r.

OA, OB, OC and OD are the radii of the bigger circle

∴ OA = OB = OC = OD = R

PQ = PA = R

OQ = OB - BQ = (R - 9)

OE = OD - DE = (R - 5)

As the chords QA and EF of the circles with centre P intersect in the interior of the circle, so by the property of internal division of two chords of a circle,

OQ × OA = OE × OF

(R - 9) × 9 = (R - 5) × (R - 5)………(∵ OE = OF)

R² - 9R = R² - 10R + 25

R = 25 cm

AQ = 2r = AB - BQ

2r = 50 - 9 = 41

Given:

PA=8cm

PM=4cm

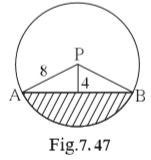
θ 60°

m(arc AB) = 2θ = 120°

In ΔPAM,

8² = 4² + (AM)²

64 = 16 + (AM)²

In ΔPAM,

8² = 4² + (AM)²

64 = 16 + (AM)²

AM = √48

AM = 4√3 cm

∴ AB = 2 AM

AB = 8√3 cm

Area of shaded portion

= A(sector) - A(Δ PAB)

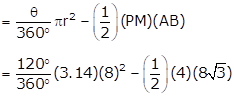
= 66.986 - 27.712

= 39.271 cm²

The area of the shaded region is 39.272 cm².