Class 10 LAKHMIR SINGH AND MANJIT KAUR Solutions Physics Chapter 1 - Electricity
Electricity Exercise 5
Solution 1
Solution 2
Solution 3
(b) Voltmeter is used to measure p.d.
Solution 4
Electric potential at a point is 1 volt means 1 joule of work is done in moving 1 unit positive charge from infinity to that point.
Solution 5
Potential difference = 1 V
Charge moved = 1C
![]()
Work done = Potential difference x Charge moved
= 1 x 1 = 1 J
Solution 6
Volt
Solution 7
Given,
Potential difference = 12 V, Charge moved = 2 C
We know that, ![]()
Work done= p.d. x charge moved
= 12 x 2
= 24 joules
Solution 8
Coulomb
Solution 9
One coulomb of charge is that quantity of charge which exerts a force of 9 x 109 Newton on an equal charge is placed at a distance of 1 m from it.
Solution 10
(a) volts; voltmeter; parallel
(b) conductor; insulator
Solution 11
Conductors:- Those substances through which electricity can flow are known as conductors. E.g., Copper, silver etc.
Insulators:- Those substances through which electricity cannot flow are known as insulators. E.g., Plastic, cotton etc.
Solution 12
Conductor:- Silver, Copper, Aluminum, Nichrome, Graphite, Mercury, Manganin
Insulators:- Sulphur, Cotton, Air, Paper, Porcelain, Mica, Bakelite, Polythene
Solution 13
The electric potential (or potential) at a point in an electric field is defined as the work done in moving a unit positive charge from infinity to that point.
Unit of electric potential is volt.
Solution 14
(a) ![]()
(b) V1=220 V, V2=230V, Charge moved=4C
Thus, the potential difference= V2- V1
=230-220
=10V
We know that, ![]()
Work done = Potential difference x Charge moved
= 10 x 4Work done = 40 joules
Solution 15
(a) Voltmeter
(b) Given : Potential difference=12V, Charge moved=1C
We know that, ![]()
Work done = Potential difference x charge moved
= 12 x 1 = 12 joules
Since work done on each coulomb of charge is 12 joules, the energy given to each coulomb of charge is also 12 joules.
Electricity Exercise 6
Solution 16
(a) Potential difference between two points in an electric circuit is defined as the amount of work done in moving a unit charge from one
point to the other point.
(b) The potential difference between two points is 1 volt means 1 joule of work is done in moving 1 coulomb of electric charge from one point to the other.
(c) Given: Work done = 250J, Charge moved = 20C
We know that,
(d) A voltmeter is a device which is used to measure the potential difference between two points in an electric circuit. Voltmeter is always connected in parallel across the two points where the potential difference is to be measured.
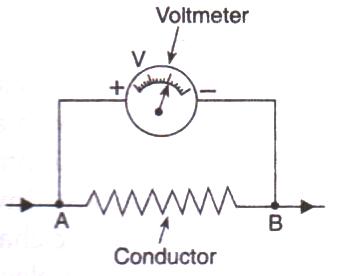
Solution 22
(a) If three cells of 2 volt each are connected in series to make a battery, then the total potential difference between terminals of the battery will be 6V.
(b) (i) Given: p.d. = 2V, Charge moved = 1C
We know that
Work done = p.d. x charge moved
= 2 x 1
Work done = 2 joules
(ii) Given: p.d. = 6V, Charge moved = 1C
Work done = p.d. x charge moved
= 6 x1
Work done = 6 joule
Solution 23
Copper has free electrons that are loosely held by the nuclei of the atoms. These free electrons result in conduction of electricity.
The electrons present in rubber are strongly held by the nuclei of its atoms. So, rubber does not have free electrons to conduct electricity.
Electricity Exercise 11
Solution 1
Ampere
Solution 2
Electric Current
Solution 3
Electrons
Solution 4
Electrons
Solution 5
(a) Conventional current flows from positive terminal of a battery to the negative terminal, through the outer circuit.
(b) Electrons flow from negative terminal to positive terminal of the battery (opposite to the direction of conventional current).
Solution 6
1A = 1C/s
Solution 7
Ampere
Solution 8
(a) 1 amp = 103 milli amp
(b) 1 amp = 106 micro amp
Solution 9
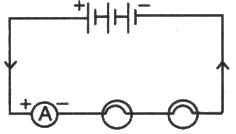
Ammeter is connected in series.
Solution 10
Ammeter is connected in series in a circuit whereas voltmeter is connected in parallel.
Solution 11
(i) Variable resistance
(ii) A closed plug key
Solution 12
Given, Q = 20 C, t=1s
I=?
We know that:
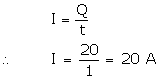
Solution 13
Given I=4amp, t=10s
Q=?
We know that:
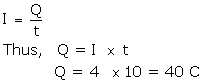
Solution 14
Given, Q=20C, t=40s
I=?
We know that:
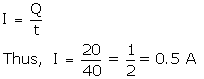
Solution 15
(a) electrons; closed
(b) amperes; ammeter; series
Solution 16
(a) Cell or battery helps to maintain potential difference across a conductor.
(b) Given: p.d. = 10 V, I = 2amp, t = 1 min = 60s
We know that:-
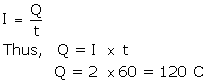
Solution 17
(a) An electric currrent is a flow of electric charges (electrons) through a conductor.
Potential difference between the ends of the wire makes electric current to flow in the wire.
(b) When 1 coulomb of charge flows through any cross-section of a conductor in 1 second, the electric current flowing through it is said to be 1 ampere.
Solution 18
Ammeter is a device used for the measurement of electric current. It is always connected in series with the circuit in which the current is to be measured.
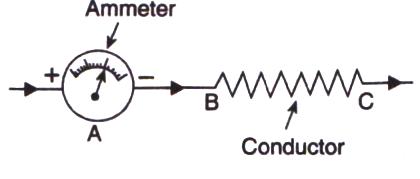
Solution 19
(b) I = 0.36 A, t = 15 min = 900 s
Solution 20
(a) The resistance of an ammeter should be very small so that it may not change the value of the current flowing in the circuit.
(b) The resistance of a voltmeter should be very large so that it takes a negligible current from the current.
Solution 21
Solution 22
A diagram which indicates how different components in a circuit have been connected by using the electrical symbols for the components is called a circuit diagram.
A voltmeter has a large resistance.
Solution 23
We know that
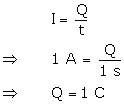
Now,
When charge is ![]() coulombs, number of electrons = 1
coulombs, number of electrons = 1
When charge is 1 coulomb, number of electrons =
Solution 24
Electricity Exercise 12
Solution 25
Solution 26
(a) Electric current is the flow of electric charges (electrons) in a conductor such as a metal wire.
SI unit of electric current is ampere.
(b) 1 ampere.
(c) An ammeter is used to measure electric current. It should be connected in series with the circuit.
(d) Conventional direction of flow of electric current is from positive terminal of a battery to the negative terminal, through the outer circuit. The direction of flow of electrons is opposite to the direction of conventional current, i.e. from negative terminal to positive terminal.
Solution 32
(a) Lamps are in series.
(b) Student has connected ammeter in parallel with lamps. It should be connected in series.
(c)
Solution 33
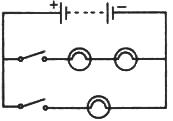
Solution 34
Solution 35
Electricity Exercise 18
Solution 1
Ohm's law
Solution 2
Solution 3
Electric resistance.
Solution 4
Insulators
Solution 5
Solution 6
Strength of electric current flowing in a given conductor depends on
(i) potential difference across the ends of the conductor
(ii) resistance of the conductor
Solution 7
Solution 8
Solution 9
Potential difference, V = 20V
Resistance, R = 5ohms
Current, I = ?
We know that
V=IR
20 = I x 5
I = 20/5 = 4 A
Solution 10
R = 20ohms
I = 2amp
We know that
V = IR
Thus,
V = 2 x 20
V = 40V
Solution 11
I = 5amp
p.d., V = 3V
We know that
V=IR
Thus,
3 = 5 x R
R = 3/5 = 0.6 ohm
Solution 12
current
Solution 13
Those substances which have very low electrical resistance are called as good conductors. E.g., copper and aluminium.
Those substances which have comparatively high resistance than conductors are known as resistors. E.g., nichrome and manganin.
Those substances which have infinitely high electrical resistance are called insulators. E.g., rubber and wood.
Solution 14
Conductor :- mercury, aluminum, iron, metal coin
Resistor :- manganin, nichrome
Insulator :- rubber, polythene, wood, bakelite, paper, thermocol
Electricity Exercise 19
Solution 15
Ohm's law gives a relationship between current (I) and potential difference (V). According to ohm's law: At constant temperature, the current flowing through a conductor is directly proportional to the potential difference across its ends.
If I is the current flowing through a conductor and V is the p.d. across its ends, then according to the ohm's law:
Solution 16
(a) The property of a conductor due to which it opposes the flow of current through it is called resistance of the conductor.
(b) V = 12volt, I=2.5 x 10-3 A
We know that
V=IR
R=V/I
R=12/(2.5X10-3)
R=4.8X103ohm = 4800 ohm
Solution 17
(a) 1 ohm is the resistance of a conductor such that when a potential difference of 1 volt is applied to its ends, a current of 1ampere flows through it.
(b) Its resistance will increase.
(c)
Solution 18
(a) Electricians wear rubber hand gloves while working with electricity because rubber is an insulator and protects them from electric shocks.
(b) I=6amp, R=40ohm
We know that
V=IR
V = 6 x 40 = 240 V
Solution 19
(i)
(ii) Since the graph is a straight line passing through the origin, so current is directly proportional to the potential difference.
Hence, the ration ![]() remains constant.
remains constant.
From graph, when V=1.5 volt, I=0.6 amp
So, ![]() =
=![]()
For p.d. 0.8V, 1.2v and 1.6V, the value of ![]() ratio remains the same i.e., 2.5 ohm.
ratio remains the same i.e., 2.5 ohm.
(iii) The resistance of the wire is equal to the ratio of potential difference applied and the current passing through it.![]()
Solution 20
(a) The ratio of potential difference and current is known as resistance.
(b)
(c) Ohm's law
(d) Potential difference = Current x Resistane
(e) V = 240 volt, I = 5ampWe know that
V=IR
240 = 5 x R
R = 240/5 = 48 ohm
Electricity Exercise 20
Solution 30
In first case,
I = 2.4 amp, V = 120 volt
V=IR
120 = 2.4 x R
R = 120/2.4 = 50 ohm
In second case,
V = 240 volt, R = 50 ohm
V = IR240 = I x 50
I =4.8 amp
Solution 31
Resistance.
Solution 32
(a) Ohm's law
(b) Temperature
Solution 33
In first case,
I = 0.02 amp, V = 10 volt
V=IR
10 = 0.02 x R
R = 10/0.02 = 500 ohm
In second case,
I = 250 x 10-3 amp, R = 500 ohm
V = IRV = 250 x 10-3 x 500
V = 125 volt
Solution 34
I = 200mA = 0.2 A
R = 4 x 103ohm = 4000 ohm
We know that
V=IR
V = 0.2 x 4000
V = 800 volt
Electricity Exercise 26
Solution 1
The resistance decreases.
Solution 2
Solution 3
Resistance of a conductor depends on the following factors:-
Length of the conductor, area of cross section of the conductor, nature of material of the conductor and temperature of the conductor
Solution 4
Solution 5
Iron
Solution 6
Solution 7
Nichrome
Solution 8
Nichrome is an alloy of nickel, chromium, manganese ad iron having a resistivity of about 60 times more than that of copper. It is used for making the heating elements of electrical heating appliances.
Solution 9
Nichrome alloy is used for making the heating elements of electrical appliances because:
(i) nichrome has very high resistivity
(ii) nichrome does not undergo oxidation (or burn) easily even at high temperature.
Solution 10
Because
(i) resistivity of an alloy is much higher than that of a pure metal
(ii) an alloy does not undergo oxidation (or burn) easily even at high temperature.
Solution 11
(a) A long piece of nichrome wire.
(b) A thin piece of nichrome wire.
Solution 12
(a) On decreasing the temperature, the resistance decreases.
(b) Presence of impurities in a metal increases the resistance.
Solution 13
Ohms; increases; increases; decreases.
Solution 14
(a) Resistivity is the characteristic property of a substance which depends on the nature of the substance and its temperature. It is numerically equal to the resistance between the opposite faces of a 1 m cube of the substance.
(b) l = 1m
r = d/2 = 0.2/2 mm = 0.1 mm = 0.0001m,
R = 10 ohm
We know that,
Solution 15
Solution 16
(a) Silver and copper are good conductors of electricity because they have free electrons available for conduction.
Solution 17
Current will flow more easily through thick wire because the resistance of the thick wire will be lesser than that of thin wire.
Solution 18
(a) Resistance of a conductor increases (or decreases) with increase (or decrease) in the length of the conductor.
(b) Resistance of a conductor decreases (increases) with increase (decrease) in the area of cross-section of the conductor.
(c) Resistance of a conductor increases on raising the temperature and decreases on lowering the temperature.
Solution 19
(a) If we take two similar wires of same length and same diameter, one of copper metal and other of nichrome alloy, we will find that the resistance of nichrome wire is about 60 times more than that of the copper wire. This shows that the resistance depends on the nature of material of the conductor.
Solution 20
(a) Resistance will increase.
(b) Resistance will decrease.
(c) Resistance will increase.
Solution 21
(a) By increasing the area of cross section, the resistance will decrease.
(b) By increasing the diameter, the resistance will decrease.
Electricity Exercise 27
Solution 22
Solution 23
Solution 24
(b) Ohm-meter
(c) 1. Resistance is the property of the conductor, while resistivity is the property of the material of the conductor.
2. Resistance of a conductor is the opposition to the flow of electric current through it. Resistivity of a substance is the opposition to the flow of electric current by a rod of that substance which is 1m long and 1m2 in cross section.
3. Resistance of a conductor depends on length, thickness, nature of material and temperature of the conductor; while resistivity of a substance depends on the nature of the substance and temperature.
(d) Resistivity of a substance depends on the nature of the substance and its temperature. It does not depend on the length or thickness of the conductor.
Solution 33
Solution 34
(a) Material Q with resistivity 2.63 X 10-8 ohm-m can be used for making electric wires because it has very low resistivity.
(b) Material R with resistivity 1.0 X 1015 ohm-m can be used for making handle of soldering iron because it has very high resistivity.
(c) Material P with resistivity 2.3 X 103 ohm-m can be used for making solar cell because it is a semiconductor.
Solution 35
(a) Good conductor = C (10 x 10-8ohm-m)
(b) Resistor = A (110 x 10-8 ohm-m)
(c) Insulator= B (1 x 1010 ohm-m)
(d) Semiconductor= D (2.3 x 103 ohm-m)
Solution 36
(a) E is best conductor of electricity due to its least electrical resistivity.
(b) C, because its resistivity is lesser than that of A.
(c) B, because it has the highest electrical resistivity.
(d) C and E, because of their low electrical resistivities.
Electricity Exercise 37
Solution 1
Solution 2
As per the law of combination of resistances in series,
R=R1+ R2+ R3+ R4+ R5
R=0.2+0.2+0.2+0.2+0.2=1ohm
Solution 3
According to the law of combination of resistance in parallel, the reciprocal of the combined resistance of a number of resistances connected in parallel is equal to the sum of the reciprocals of all the individual resistances.
Solution 4
Solution 5
Since the resultant resistance is less than the individual resistances, so the resistances should be connected in parallel.
Solution 6
In case of parallel combination, the resultant resistance will be less than either of the individual resistances.
Solution 7
R1=2ohm, R2=6ohm
Case I: (Parallel combination)
1/R = 1/R1+ 1/R2
1/R = 1/2 + 1/6 = 4/6
R = 6/4 = 1.5ohm
Case II: (Series combination)
R = R1 + R2 = 2+6 = 8ohm
Electricity Exercise 38
Solution 17
Solution 8
(a) By connecting in parallel: Since equivalent resistance will be
1/ R = 1/4 + 1/4 = 2/4 = 1/2
Therefore, R = 2 ohm
(b) By connecting in series : Since equivalent resistance will be
R = 4 ohm + 4 ohm = 8 ohm
Solution 9
Resistance of arrangement A is 10 ohm.
Combined resistance of arrangement B is caculated as follows:
1/R = 1/10 + 1/1000 = (100+1)/1000
R = 1000/101 = 9.9 ohm
Therefore, arrangement B has lower combined resistance.
Solution 10
Resistance of each part is R/2.
Resultant resistance R' is given by
1/R' = 2/R+2/R
R'=R/4
Solution 11
(a) R1=500ohm, R2=1000ohm
As per given figure,
R=R1+R2=500+1000=1500ohm.
(b) R1=2ohm, R2=2ohm
As per given figure,
1/R=1/R1+1/R2
1/R=1/2+1/2
R=1ohm
(c) R1=4ohm, R2=4ohm, R3=3ohm
As per given figure,1/R=1/R1+1/R2
1/R=1/4+1/4
R=2ohm
Total resistance =R+R3
=2+3=5ohm
Solution 12
R1=6ohm, R2=4ohm V=24V
The two resistances are connected in parallel.
Current across R1=I1=V/R1=24/6=4amp
Current across R2=I2=V/R2=24/4=6amp
Solution 13
(i) Series combination
When two or more resistances are connected end to end consecutively, they are said to be connected in series combination. The combined resistance of any number of resistances connected in series in equal to the sum of the individual resistances.
R=R1+R2+......
The resultant resistance is more than either of the individual resistances.
(ii) Parallel combination
When two or more resistances are connected between the same two points, they are said to be connected in parallel combination. The reciprocal of the combined resistance of a number of resistances connected in parallel is equal to the sum of the reciprocals of all the individual resistances.
1/R=1/R1+1/R2+........
The resultant resistance is less than either of the individual resistances.
Solution 14
R1=0.2ohm, R2=0.4ohm, R3=0.3ohm, R4=0.5ohm, R5=12ohm, V=9V
Resultant resistance=R1+ R2+ R3+ R4+ R5
R=0.2+0.4+0.3+0.5+12=13.4ohm
Thus the current flow through 12ohm resistance will be=V/R
I=9/13.4
I=0.67amp.
Solution 15
(a) Total resistance of the circuit=R1+R2=20+4=24ohm
(b) We know that
V=IR
Therefore,
6=I x 24
I=6/24=0.25amp
(c) p.d. across bulb=IR1=0.25X20=5V
(d) p.d. across resistance wire=IR2=0.25X4=1V
Solution 16
According to the diagram,
(i) Total current I=1amp is entering the parallel combination of R1 and R2. Let I1 current flow through R1 and I2 current flow through R2.
Then
(ii) p.d. across AB = IR3 = 1 x 5 = 5V
Equivalent resisyance between B and C is
1/R' = 1/R1 + 1/R2 = 1/10 + 1/15
1/R' = 5/30
R' = 6 ohm
Total resistance between A and C is R = 5+6 = 11 ohm
p.d. across AC = IR = 1x11 = 11V
(iii) Total resistance = R3 + R' = 5 + 6 = 11 ohm
Electricity Exercise 39
Solution 18
Given: Two resistors with resistances R1=5ohm and R2=10ohm, V=6volt
(a) For minimum current these two should be connected in series. For maximum current these two should be connected in parallel.
(b)In series,Total resistance = 5+10 = 15ohms
Therefore total current drawn = V/R = 6/15 = 0.4amps
In parallel,Total resistance R is given as
1/R=1/R1+1/R2
1/R=1/5+1/10
1/R=3/10
R=10/3ohm
Therefore total current drawn by the circuit = V/R = 6/(10/3) =1.8amps
Solution 19
(i) Total resistance of two resistors that are connected in parallel is
1/R' = 1/3+1/6
1/R' = 3/6
R' = 2ohms
Total resistance of the circuit = 2+4ohms = 6ohms
(ii) Total current flowing through the circuit=V/total resistance
I = 12/6 = 2amps
(iii) Potential difference across R1=R1 x I = 4 x 2 = 8V
Solution 20
Given :-
1 amp current is flowing through 5ohm resistor.
We know that in case of parallel connection, the p.d. across each resistor is same and is equal to the voltage applied.
Therefore, applied voltage, V = IR = 1 x 5 = 5V
So,
Current through 4 ohm resistor = V/R = 5/4 = 1.25 A
Current through 10 ohm resistor = V/R = 5/10 = 0.5 A
Solution 21
Solution 22
Given V=220V
RA = RB = 24 ohm
(a) Current drawn when only coil A is used:
I = V/RA = 220/24
=9.16amps
(b) Current drawn when coils A and B are used in series:
Total resistance, R = RA + RB = 24+24 = 48ohms
I = V/R = 220/48
=4.58amps
(c) Current drawn when coils A and B are used in parallel:
Total resistance, 1/R = 1/RA + 1/RB = 1/24 + 1/24 = 2/24 = 1/12
R=12ohms
I = V/R = 220/12
=18.33amps
Solution 23
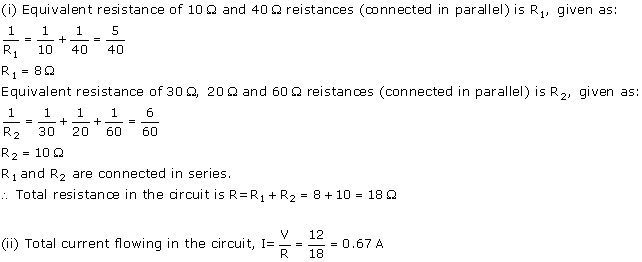
Electricity Exercise 40
Solution 24
V=12V
R1, R2 and R3 are connected in parallel.
(a) Current through R1 = V/R1 = 12/5 = 2.4 A
Current through R2 = V/R2 = 12/10 = 1.2 A
Current through R3 = V/R2 = 12/30 = 0.4 A
(b) Total current in the circuit = 2.4 + 1.2 + 0.4 = 4 A
(c) Total resistance in the circuit=R
1/R = 1/R1+ 1/R2 + 1/R3
1/R = 1/5 + 1/10 + 1/30
1/R = 10/30
R = 3 ohm
Solution 25
V = 4V,
R1 = 6 ohm, R2 = 8 ohm (in series)
(a) Combined resistance, R = R1 + R2 = 6+2 = 8ohm
(b) Current flowing, I = V/R = 4/8=0.5amp
(c) p.d. across 6ohm resistor = I x R1 = 0.5 x 6 = 3 V
Solution 26
V = 6V
R1 = 3 ohm, R2 = 6 ohm (in parallel)
(a) Combined resistance,
1/R = 1/R1 + 1/R2
1/R = 1/3 + 1/6 = 3/6 = 1/2
R = 2 ohm
(b) Current flowing in the main circuit, I = V/R = 6/2 = 3 A
(c) Current flowing in 3 ohm resistor = V/R1 = 6/3 = 2 A
Solution 28
Total current flowing through circuit, I = 6 A
R1 = 3 ohm, R2 = 6 ohm
(a) Combined resistance R is
1/R=1/3+1/6
1/R=3/6
R=2ohms
(b) p.d. across the combined resistance = IR = 6 x 2 = 12 V
(c) p.d. across the 3 ohm resistor = p.d. across the combined resistance = 12 V
(d) Current flowing through the 3 ohm resistor = V/R1 = 12/3 = 4 A
(e) Current flowing through the 6 ohm resistor = V/R2 = 12/6 = 2 A
Solution 27
Electricity Exercise 41
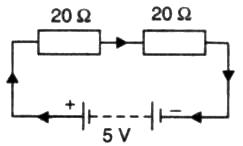
Solution 29
(a)
(b) Effective resistance = 20 + 20 = 40 ohms
(c) Current flowing through the circuit = I = V/R = 5/40 = 0.125 amps
(d) p.d. across each resistance = I x R = 0.125 x 20 = 2.5 V
Solution 30
V=6V, R1=2ohms, R2=3ohms
(a) Resistors are connected in parallel
(b) p.d. across each resistor is same and is equal to 6V.
(c) 2 ohms resistance have bigger share of current because of its lower resistance.
(d) Effective resistance=R
1/R=1/2+1/3
1/R=5/6
R=1.2ohms
(e) Current flowing through battery, I=V/R=6/1.2=5amps
Solution 33

Solution 31
Solution 32
Electricity Exercise 42
Solution 34
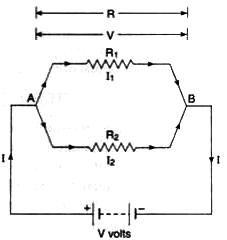
Suppose total current flowing the circuit is I, then the current passing through resistance R1 will be I1 and current passing through resistance R2 will be I2.
Total current =I=I1+I2
Let resultant resistance of this parallel combination is R. By applying the Ohm's law to the whole circuit, we get that I=V/R
Since the potential difference across the both the resistances is same, so applying the Ohm's law to each resistance we get that
I1=V/R1
I2=V/R2
Putting these eq in the above one, we get that
V/R=V/R1+V/R2
1/R=1/R1+1/R2
If two resistance are connected in parallel than, the resultant resistance will be
1/R=1/R1+1/R2
(b)
(i) Total reisitance=R
1/R=1/R1+1/R2
R2=3+2=5ohms
R1=5ohms
1/R=1/5+1/5
1/R=2/5
R=2.5ohms
(ii) Current flowing through the circuit
I=V/R=4/(2.5)
=1.6amps
Solution 35
Suppose total current flowing in the circuit is I, then the current passing through resistance R1 will be I1, current passing through resistance R2 will be I2 and current passing through resistance R3 will be I3.
Total current =I=I1+I2+I3
Let resultant resistance of this parallel combination is R. By applying the Ohm's law to the whole circuit, we get that
I=V/R
Since the potential difference across all the resistances is same, so applying the Ohm's law to each resistance we get that
I1=V/R1
I2=V/R2
I3=V/R3
Putting these eqs. in the above one, we get
V/R=V/R1+V/R2+ V/R3
1/R=1/R1+1/R2+ 1/R3
If two resistance are connected in parallel, then the resultant resistance will be
1/R=1/R1+1/R2+ 1/R3
(b) If switch is open, then only upper two resistances (connected in parallel) are in the circuit.
Effective resistance is 1/Req=1/R+1/R=2/R
Req = R/2
So the current=I=V/(R/2)=0.6A (given)
V/R = 0.3 A
When the switch closes, the third resistance also comes in the circuit. The effective resistance of the circuit becomes R/3
Hence, Current I = V/(R/3) = 3 (V/R) = 3 x 0.3 = 0.9 AElectricity Exercise 43
Solution 43
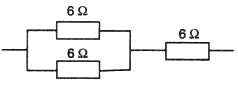
(i)
Resultant resistance for parallel circuit=R
1/R=1/6+1/6
1/R=2/6
R=3
Effective resistance=6+3=9ohms
(ii)
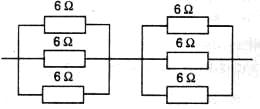
Resultant resistance for each parallel circuit=R
1/R=1/6+1/6+1/6
1/R=3/6
R=2
Therefore effective resistance=2+2=4ohms.
Solution 44
Two resistances when connected in parallel, resultant value is 2ohms.
Let the two resistances be R1 and R2.
If connected in series, then
9=R1+R2
R1=9-R2
If connected in parallel, then
1/2=1/R1+1/R2
From above equations we get that
1/2=(R1+R2)/R1R2
1/2=9/(9-R2) R2
9R2-R22=18
R22-9R2+18=0
(R2-6) (R2-3)=0
R2=6,3
So if R2=6ohms, then R1=9-6=3ohms.
If R2=3ohms, then R1=9-3=6ohms.
Solution 45
Given:
A resistor of 8ohm is connected in parallel with a resistor of X.
And resultant is 4.8.
Then X=?
We know that for parallel case
1/R=1/R1+1/X
1/4.8=1/8+1/x
1/4.8 - 1/8 = 1/x
After solving we get that
X=12ohms
Solution 46

Electricity Exercise 44
Solution 47
Given: Three resistances of 2ohms, 3ohms, 5ohms.
Their resultant, R=2.5ohms
Resistance of first line = 2+3 = 5 ohm
So, 1/R = 1/5 + 1/5
On solving we get that
R=2.5ohms
Solution 48
(a) Connect 2ohms resistor in series with a parallel combination of 3ohms and 6ohms.
(b) Connect 2ohms, 3ohms, and 6ohms in parallel.
Solution 49
(a) For obtaining the highest resistance by combining the given resistances, we must connect them in series.
We get,
R=4+8+12+24=48ohms
(b) For obtaining the lowest resistance by combining the given resistances, we must connect them in parallel.
We get,
1/R=1/4+1/8+1/12+1/24
On solving we get, R=2ohms
Solution 50
So, the resultant of these three resistances = 20+20+10 = 50ohms.
This 50ohms is in parallel with 30ohms. So resultant of these two will be
1/R=1/30+1/50
1/R=80/1500
R=18.75ohms
Now, the resistances 10 ohms, 18.75 ohms and 10 ohms are in series.
Therefore, resultant resistance = 18.75+10+10 = 38.75ohms.
Solution 51
Given: n=100, R=1 ohm
For obtaining the smallest resistance, these resistances are connected in parallel:
Equivalent resistance = 1/1 + 1/1 + 1/1.....100 times = 100/1
Req = 1/100 = 0.01 ohm
For obtaining the largest resistance, these resistances are connected in series:
Equivalent resistance = 1 + 1 + 1.....100 times = 100
Req = 100 ohm
Solution 52
For obtaining 250ohms, connect two 100ohms in series with a parallel combination of two 100ohms.
Solution 53
Req = R+R+R+R = 4R ohm
Total current in the circuit, I = V/R = 12/4R = 3/R
Reading of voltmeter A = Voltage across R1 = I x R1 = 3/R x R = 3V
Reading of voltmeter B= Voltage across R2 = I x R2 = 3/R x R = 3V
Reading of voltmeter C= Voltage across the series combination of R3 and R4 = I x (R3+R4) = 3/R x 2R = 6V
Solution 54
1/R = 1/16 + 1/16 + 1/16 + 1/16 = 4/16
R = 4 ohm
Four such combinations are connected in series, so total resistance = 4+4+4+4 = 16 ohm.
Solution 55
The total current of 0.5 A flowing in the circuit distributes equally in the two arms having lamps (since the lamps have same resistances). So the current through each of these arms is 0.25 A. Hence A2, A3, A4 and A5, all will read 0.25 A.
Electricity Exercise 47
Solution 1
No, they are wired in parallel.
Solution 2
All the other bulbs also stop glowing.
Solution 3
All the other bulbs keep glowing.
Solution 4
(a) Series
(b) Parallel
Solution 5
The two lamps (of 4V each) should be arranged in parallel with the two 2V cells.
Solution 6
A series arrangement is not used for connecting domestic electrical appliances in a circuit because if one electrical appliance stops working due to some defect, then all other appliance also stop working as the whole circuit is broken.
Solution 7
Different electrical appliances in a domestic circuit are connected in parallel because of the following advantages:
(i) If one electrical appliance stops working due to some defect, then all other appliances keep working properly.
(ii) Each electrical appliance has its own switch due to which it can be turned on or turned off independently, without affecting other appliances.
(iii) Each electrical appliance gets the same voltage as that of the power supply line.
Solution 8
(a) Parallel circuit
(b) Parallel circuit
(c) Series circuit
(d) Series circuit.
Solution 9
(a) circuit (ii)
(b) circuit (iii)
(c) circuit (iii)
Electricity Exercise 48
Solution 10
Parallel arrangement because if one electrical bulb stops glowing due to some defect the other will keep glowing.
Parallel arrangement takes more current from the battery due to its lesser equivalent resistance.
Solution 11
(a) Parallel circuits - Because if one electrical appliance stops working due to some defect, then all other appliances in the circuit will keep working properly.
(b) All the other lamps stop glowing.
(c) All lamps are connected in series.
(d)
Solution 14
(a)
(b) The brightness of the lamps can be changed by connecting the lamps in parallel.
Solution 15
(a) C will be the brightest. Voltage will be distributed equally between A and B, so they will have equal brightness but lesser than that of C.
(b) A gets the same voltage as before, so its brightness remains the same.
(c) If B burns put, A will also stop glowing because it is connected in series with B. However, brightness of C remains the same.
Solution 16
The brightness of two lamps arranged in parallel is much more those arranged in series.
Solution 17
(a) In case of series connection, if filament of one lamp breaks, the other wil stop glowing.
(b) In case of parallel connection, if filament of one lamp breaks, the other will keep glowing.
Electricity Exercise 49
Solution 18
(a) Turn the switch to right side so as the resistance decreases.
(b) Turn the switch to the left side so as the resistace increases.
Electricity Exercise 58
Solution 1
Electrical energy consumed by an electrical appliance depends on:
1. Power rating of the appliance.
2. Time for which the appliance is used.
Solution 2
60 watt bulb, because power is inversely proportional to the resistance.
Solution 3
Kilowatt-hour is the commercial unit of electric energy.
Solution 4
V = 220 V, P = 100W
R=?
We know that
P = V2/R
Thus
R = V2/P = 2202/100 = 484ohm
Solution 5
(i) joule
(ii) watt
Solution 6
(i) Electric power
(ii) Electric energy
Solution 7
Electric power has the unit of watt.
Solution 8
kWh is the short form of kilowatt-hour, which is the commercial unit of electrical energy.
Solution 9
P=V2/R
R is fixed.
V becomes double.
Now, P = (2V)2/R = 4 V2/R
So, the electric power becomes four times its previous value.
Solution 10
Other information is that it will consume energy at the rate of 36 J/s.
Solution 11
P=920W, V=230V, I=?
We know that
P = V x I,
920 = 230 x I
I = 920/230 = 4amp
Solution 12
When an electrical appliance consumes electrical energy at the rate of 1 joule per second, its power is said to be 1 watt.
1 watt = 1 volt x 1 ampere
Solution 13
One watt hour is the amount of electrical energy consumed when an electrical appliance of 1 watt power is used for 1 hour.
1 watt hour = 3600 joules
Solution 14
I=5amp, R=100ohms, t=2h
We know that
Electric energy consumed = P x t = I2Rt
= 52 x 100 x 2
= 5000 Wh
= 5 kwh
We know that 1kwh = 3.6 x 106 JTherefore, 5kwh = 5 x 3.6 x 106 J = 18 x 106 J
Solution 15
V=220V, I=0.5amp, P=?
We know that
P=VI=220X0.5
P=110 watt
Solution 16
(i) R = 300 ohm, I = 1 A, t = 1h
P = I2R = 12 x 300 = 300 W
E = P x t = 300 x 1 = 300 Wh
(ii) R = 100 ohm, I = 2 A, t = 1h
P = I2R = 22 x 100 = 400 W
E = P x t = 400 x 1 = 400 Wh
Hence, in case (ii), the electrical energy consumed per hour is more.
Solution 17
V=220V, P=2.2kW=2200W, t=3h
We know that
Electrical energy consumed = Pxt = 2.2x3 = 6.6 kWh
We have, P = V x I
2200 = 220 x I
I=10amp
Electricity Exercise 59
Solution 18
Solution 19
V=250V, I=0.4amp
(i) We know that
Power=VI=250X0.4=100watt
(ii) We have
P=I2R
100=0.42XR
R=625ohm
Solution 21
I=5amp, V=220volt, t=2h
P=?, E=?
P=VXI
=220X5
=1100watt
=1.1kW
Energy consumed, E=PXt
=1.1X2
=2.2kWh
Solution 22
Case 1: TV set
P=250W=0.25kWh
t=1h
Energy consumed=PXt=0.25X1=0.25kWh
Case 2: Toaster
P=1200W=1.2kW, t=10min=10/60=1/6h
Energy consumed=PXt=1.2X(1/6)=0.2kWh
Thus, TV uses more energy.
Solution 23
Solution 24
Given 2 lamps:P1=40W, P2=60W
V=220V
(a)
(b) Voltage across both the bulbs is same and is equal to 220V.
Current through 40W lamp = I1 = P1/V = 40/220 A
Current through 60W lamp = I2 = P2/V = 60/220 A
Total current drawn from the electric supply = 40/220 + 60/220 = 0.45 A
(a) Energy consumed by 40 W lamp in 1 hr, E1 = P1 x t = 40 x 1 = 40Wh
1Wh = 3.6 kJ
E1 = 40 x 3.6 = 144 kJ
Energy consumed by 60W lamp in 1 hr, E2 = P2 x t = 60 x 1 = 60Wh = 216 kJ
Total energy consumed = 144 + 216 = 360 kJ
Solution 25
Given V=230V, I=10amp
(a) P=VI
P=230X10
P=2300watt = 2300 J/s
(b) Energy consumed in minute = P x t = 2300 J/s x 60s = 138000 J
Solution 26
For heater:
P=2kW, t=4h
E=Pxt=2x4=8kWh
For TV:
P=200W=0.2kW, t=4h
E=Pxt=0.2x4=0.8kWh
Lamps:
P=100W=0.1kW, t=4h, n=3
E=nxPxt=3x0.1x4=1.2kWh
Total energy consumed = 8+0.8+1.2 = 10kWh
Cost of 1kWh = Rs. 5.50
Cost of 10kWh = Rs. 5.50 x 10 = Rs. 55
Solution 27
I=13amp, V=230V
Power=VI
=230X13
=2990W
P=2.99kW
Solution 28
Given :- V=230V, I=0.4amp
Rate at which electric energy is transferred = Power
Power = VxI
= 230 x 0.4
= 92 W = 92 J/s
Solution 29
(a) The rate at which electrical work is done or the rate at which electrical energy is consumed, is known as electric power.
It is given by
P=VI=watt
(b) Given: V=3V, I=0.5amp
(i) R=?
We know that V=IR3=0.5R
R=6ohms
(ii) Power of lamp=VI
=3x0.5
=1.5watt
(c) One kilowatt hour is the amount of electrical energy consumed when an electrical appliance having a power rating of 1 kilowatt is used for 1 hour.
1kWh=3.6x106J
(d) Given P=500W=0.5kW, t=20hr
We know that
Energy consumed =Pxt=0.5X20
=10kwh
Total cost =10xcost per uint
Cost per unit=Rs. 3.9 per unit
Therefore, total cost=10x3.9=Rs 39
Solution 20
Given
P=4kw, V=220v
(a) I=?
Power=VI=250XI
4000=250I
I=16amp
(b) R=?
P=I2R
P=162XR
R=4000/162
R=15.25ohm
(c) Energy consumed in two hour=PXt
=4X2
=8kw-hr
(d) If 1kwh=Rs 4.6
total cost=8 x 4.6=Rs 36.8
Electricity Exercise 60
Solution 41
By reducing the length of element the resistance will decrease.
Power is inversely proportional to resistance. So, this will result in more consumption of energy.
Solution 42
(a) Lamp; because least current is flowing through it.
(b) Large current drawn by the kettle; Earth connection needed.
(c) We know that
P=VI
V=240V, I=8.5A
P=240X8.5=2040W=2.04kW
(d) When connected to 240 V supply, P=2040W
R = V2/P = 2402/240
R=28.23ohm
Now, when V=120V, R=28.23ohm
I=V/R=120/28.23=4.25A
Solution 43
(a) 42919
(b) 42935
(c) 42935-42919=16 units
(d) 24 hours
(e) Cost of 1 unit = Rs. 5
Cost of 16 units = 16x5 = Rs. 80
Solution 44
P=10W, V=220V, I=5A
We know that
P=VI
=220X5
P=1100W
Power of one bulb=10W
Total no. of bulbs that can be connected=1100/10=110
Solution 45
Electricity Exercise 66
Solution 1
Heat produced is directly proportional to the square of current.
Solution 2
If current I is doubled, heat H will be four times.
Solution 3
Two effects of produced by electric current are:
(a) Heating effect
(b) Magnectic effect
Solution 4
Heating effect
Solution 5
Heating effect
Solution 6
Electirc heater and electric fuse.
Solution 7
Argon and nitrogen.
Solution 8
Filament type electric bulbs are not power efficien because most of the electric power consumed by the filament of a bulb appears as heat and only a small amount of electric power is converted into light.
Solution 9
The connecting cord of the heater made of copper does not glow because negligible heat is produced in it by passing current (because of its extremely low resistance); but the heating element made of nichrome glows because it becomes red-hot due to the large amount of heat produced on passing current (because of its high resistance).
Solution 10
(a) Heat produced, H=I2Rt
(b) Given: R=20ohm, I=5amp, t=30s
We know that H=I2Rt
H=52x20x30
H=15000 J
Solution 11
Heat produced by an electric current depends on the following factors:
(i) Heat produced is directly proportional to square of current.
(ii) Heat produced is directly proportional to resistance.
(iii) Heat produced is directly proportional to the time for which current flows.
Solution 12
(a) Joule's law of heating states that heat produced in joules when a current of I amperes flows in a wire of resistance R ohms for time t seconds is given by H = I2Rt.
Thus the heat produced in a wire is directly proportional to:
(i) Square of current
(ii) Resistance of wire
(iii) Time for which current is passed
(b) Given: R1=40ohms, R2=60ohms (in series), V=220V, t=30secWe know that
Total resistance, R=40+60=100ohms
By Ohm's law,
V=IR
I=V/R
I=220/100=2.2amp
Putting the values of I, R and t in eq. H=I2RT
H= 2.22 X 100 X 30
H= 14520 J
Solution 13
If air is filled in an electric bulb, then the extremely hot tungsten filament would burn up quickly in the oxygen of air. So, the electric bulb is filled with a chemically unreactive gas like argon or nitrogen. Thes gases do not react with the hot tungsten filament and hence prolong the life of the filament of the bulb.
Solution 14
Tungsten is used for making the filaments of electric bulbs because it has a very high melting point. Due to its very high melting point, the tungsten filament can be kept white hot without melting away. Also, tungsten has high flexibility and low rate of evaporation at high temperature.
Solution 15
The connecting wires of the heater get only slightly warm because they have extremely low resistance due to which negligible heat is produced in them by passing current.
Solution 16
Given: I=4amp, t=10min=10x60=600sec, H=2.88x104J
(a) We have
H=I2RT
28800=42xRx600
R=3ohms
We know that
P=I2xR
=42x3
P =48W
(b) V=?
We know that
V=IR
V=4x3
V=12V
Solution 17
Given: R=200ohms, I=2.5amp, t=1sec
We know that
H=I2RT
H=2.52X200X1
H = 1250 J/s
Solution 18
Given: R=8ohms, I=15amp, t=1sec
We know that
H=I2RT
H=152X8X1
H=1800J/s
Solution 19
Given: R=25ohms, V=12V, H=?, t=60sec
V=IR
12=25XI
I=0.48amp
We have
H=I2RT
H=0.482X25X60
H=345.6J
Solution 20
Given: H=100J, t=1sec, R=4ohms,
We know that
H=I2RT
100=I2X4X1
100/4=I2
I=5amp
V=IR
V=5X4
=20V
Solution 21
P=VI
I=P/V=12/12=1A
V=IR
R=V/I=12/1=12ohm
H=I2Rt
H=12x12x60
H=720J
(c) The heat produced by the heater will become one-fourth because heat produced is directly proportional to the square of the current.
(d) When an electric current is passed through a high resistance wire, the wire becomes very hot and produces heat. This effect is knows as heating effect of current. This effect is used in room heaters and electric ovens.
(e) Tungsten is used for making the filaments of an electric bulb.
Electricity Exercise 67
Solution 31
(a) S; because it has high resistivity of 11X10-7ohm-m (it is actually nichrome).
(b) Q; because it has very low resistivity of 1.7 X10-8ohm-m (it is actually copper).
(c) R; because it has very very high resistivity of 1.0X1015ohm-m (it is actually rubber).
Solution 32
(a) The filament wire becomes white hot where as other wires in the circuit do not get heated much.
(b) High resistance of filament wire accounts for this difference.
Solution 33
In series, because total resistance in series connection is more than that in parallel connection.
Solution 34
Given: V=220V, Pmin=360W, Pmax=840W
For minimum heating case:
We know that
Pmin=VI
360=220XI
I=1.63amp
R=V/I
R=220/1.63
R=134.96ohms
For maximum heating case:
We know that
Pmax=VI
840=220XI
I=3.81amp
R=V/I
R=220/3.81
R=57.74ohms
Solution 35
Electric iron , electric oven, water heater, room heater.