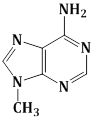
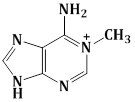
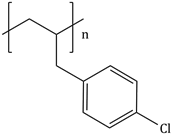
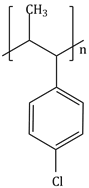
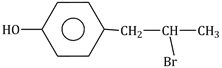Question Paper (Section wise)
-
1) Mobility of electron in a semiconductor is defined as the ratio of their velocity to the applied electric field. If, for an n-type semiconductor, the density of electrons is 1019 m-3 and their mobility is 1.6 m2 / (V. s) then the resistivity of the semiconductor (since it is an n-type semiconductor contribution of holes is ignored) is close to:
-
2 Ωm
-
4 Ωm
-
0.4 Ωm
-
0.2 Ωm
-
-
2) A copper wire is stretched to make it 0.5% longer. The percentage change in its electric resistance if its volume remains unchanged is
-
2.0%
-
2.5%
-
1.0%
-
0.5%
-
-
3) When the switch S, in the circuit shown is closed, then the value of current I will be
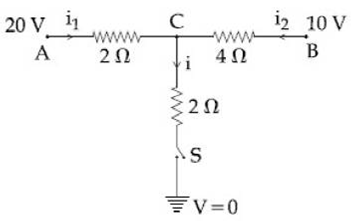
-
3 A
-
5 A
-
4 A
-
2 A
-
-
4) Consider the nuclear fission Ne20 ? 2He4 + C12. Given that the binding energy/ nucleon of Ne20, He4 and C12 are, respectively, 8.03 MeV, 7.07 MeV, and 7.86 MeV, Identify the correct statement:
-
energy of 12.4 MeV will be supplied
-
8.3 MeV energy will be released
-
energy of 3.6 MeV will be released
-
9.72 MeV of energy is released
-
-
5) Charges – q and + q located at A and B, respectively, constitute an electric dipole. Distance AB = 2a, O is the mid-point of the dipole OP is perpendicular to AB. A charge Q is placed at P where OP = y and y >> 2a. The charge Q experience an electrostatic force F. If Q is now moved along the equatorial line to P’ such that
 , the force on Q will be close to:
, the force on Q will be close to: 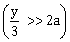
-
3F
-

-
9F
-
27F
-
-
6) A rigid massless road of length 3I has tow masses attached at each end as shown in the figure. The rod is pivoted at point P on the horizontal axis ( see figure). When released from initial horizontal position, its instantaneous angular acceleration will be :

-
-
7) There are two long co – axial solenoids of same length I. The inner and outer coils have radii r1 and r2 and number of turns per unit length n1 and n2 respectively. The ratio of mutual inductance to the self – inductance of the inner – coil is:
-
-
8) The resistance of the meter bridge AB in given figure is 4? With a cell emf ?=005 V and rheostat resistance Rh =2? the full point is obtained at some point J. When the cell is replaced by another one of emf ? = ?2 the same null point J is found for Rn=6 ?. The emf ?2 is:
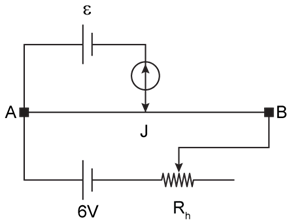
-
0.4 V
-
0.3 V
-
0.6 V
-
0.5 V
-
-
9) If the de Broglie wavelength of an electron is equal to 10-3 times the wavelength of a photon of frequency 6 × 1014 Hz, then the speed of electron is equal to: (Speed of light=3 × 10=8=m/s; Planck’s constant=6.63 × 10-34J.s; Mass of electron=9.1 × 10-31Kg)
-
1.1 × 106 m/s
-
1.7 × 106 m/s
-
1.8 × 106m/s
-
1.45 × 106 m/s
-
-
10) Two satellites, A and B, have masses m and 2m respectively. A is in a circular orbit of radius R, and B is in a circular orbit of radius 2R around the earth. The ratio of their kinetic energies, TA / TB, is:
-
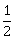
-
1
-
2
-
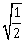
-
-
11) A block kept on a rough inclined plane, as shown in the figure, remains at rest upto a maximum force 2 N down the inclined plane. The maximum external force up the inclined plane that does not move the block is 10 N. the coefficient of static friction between the block and the plane is:
[Take g = 10 m / s2]
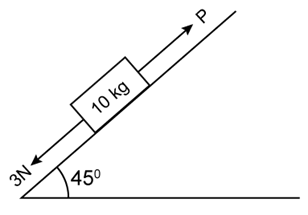
-
-
12) Two particles A, B are moving on two concentric circles of radii R1 and R2 with equal angular speed ?. At t = 0, their positions and direction of motion are shown in the figure:
The relative velocity
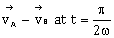 is given by:
is given by:
-
-
13) A parallel plate capacitor has 1µF capacitance. One of its two plates is given + 2µC charge and the other plate, +4µC charge. The potential difference developed across the capacitor is:
-
3V
-
1V
-
5V
-
2V
-
-
14) In a line of sight radio communication, a distance of about 50 km is kept between the transmitting and receiving antennas. If the height of the receiving antenna is 70m, then the minimum height of the transmitting antenna should be: (Radius of the Earth = 6.4×106m)
-
32 m
-
40 m
-
51 m
-
20 m
-
-
15) The very long, straight and insulated are kept at 90° angle from each other In xy – plane as shown in the figure.
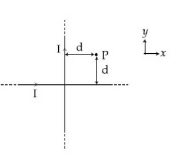
These wires cary currently of equal magnitude I, whose directions are shown in the figure. The met magneti field at point P will be:
-
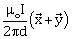
-
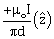
-
Zero
-
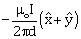
-
-
16) The figure shows a Young’s double slit experimental setup. It is observed that when a thin transparent sheet of thickness t and refractive index ? is put in front of one of the slits, the central maximum gets shifted by a distance equal to n fringe widths. If the wavelength of light used is ?, t will be:
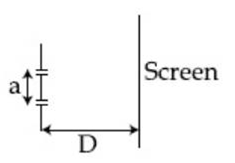
-
-
17) The stream of a river is flowing with a speed of 2 km/h. A swimmer can swim at a speed of 4 km/h. What should be the direction of the swimmer with respect to the flow of the river to cross the river straight?
-
60°
-
90°
-
120°
-
150°
-
-
18) The pressure wave, P = 0.01 sin [1000t – 3x] NM–2, corresponds to the sound produced by a vibrating blade on a day when atmospheric temperature is 0°C. On some other day when temperature is T, the speed of sound produced by the same blade and at the same frequency is found to be 336 ms–1. Approximate value of T is:
-
12°C
-
11°C
-
15°C
-
4°C
-
-
19) Two blocks A and B of masses mA = 1kg and mB = 3 kg are kept on the table as shown in figure. The coefficient of friction between A and B is 0.2 and between B and the surface of the table is also 0.2. The maximum force F that can be applied on B horizontal, so that the block A does not slide over the block B is:
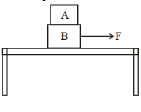
-
8 N
-
16 N
-
12 N
-
40 N
-
-
20) The formula X = 5YZ2 X and Z have dimensions of capacitance and magnetic field respectively. What are the dimensions of Y in Sl units?
-
[M-2 L0 T-4 A-2]
-
[M-3 L-2 T8 A-1]
-
[M-2 L-2 T-6 A3]
-
[M-1 L-2 T4 A2]
-
-
21) In a Young’s double slit experiment the ratio of the slit’s width is 4:1. The approximate value of ratio of the intensity of maxima to minima, close to central fringe on the screen will be _______.
-
22) A man (mass = 50 kg) and his son (mass = 20 kg) are standing on a frictionless surface facing each other. The man pushes his son so that he starts moving at a speed of 6 ms-1 with respect to the man. The speed of the man with respect to the surface is ______ m/s.
-
23) The transfer characteristic curve of a transistor, having input and output resistance 100 ? and 100 k? respectively, is shown in the figure. For the given case the Voltage gain is ________ kV.
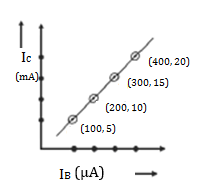
-
24) A gas can be transported from point A to point B using one of two processes: ACB or ADB. When the path ACB is used, 40 J of heat enters the system. If path ADB is used, the system's work down is 10 J, and the heat flow into the system in path ADB is _____ J.
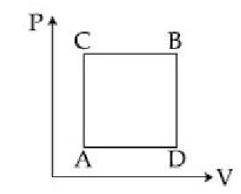
-
25) A conducting circular loop made of a thin wire, has area 3.5 × 10-3 m2 resistance 40 ?. It is placed perpendicular to a time dependent magnetic field B(t) = (0.4T) sin (50?t). The field is uniform in space. Then the net charge flowing through the loop during t = 0 s and t = 10 ms is close to ______ ?C.
-
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
-
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
-
1) Adsorption of a gas follows Freundlich adsorption isotherm. In the given plot, x is the mass of gas adsorbed on mass m of the adsorbent at pressure.
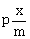 is proportional to
is proportional to
-
p2
-
p1/4
-
p1/2
-
p
-
-
2) The ore that contains both iron and copper is
-
Copper pyrites
-
malachite
-
dolomite
-
azurite
-
-
3) Aluminium is usually found in + 3 oxidation state. In contrast, thallium exists in + 1 and + 3 oxidation states. This is due to
-
inert pair effect
-
diagonal relationship
-
lattice effect
-
lanthanoid contraction
-
-
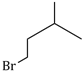
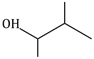
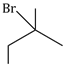
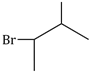
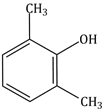
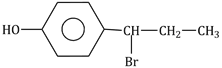
4) The major product 'Y' in the following reaction is:

-
-
5) An ideal gas undergoes isothermal compression from 5 m3 to 1m3 against a constant external pressure of 4 NM-2. Heat released in this process is used to increase the temperature of 1 mole of Al. If molar heat capacity of AI is 24 Jmol-1K-1, the temperature of Al is increase by:
-
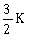
-
2 K
-
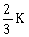
-
1 K
-
-
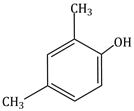
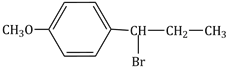
6) The major product of the following reaction is:

-
-
7) The correct match between item I and item II is:
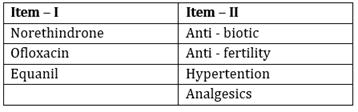
-
a – q, b – r, c – s
-
a – q, b – p, c - r
-
a – r, b – p, c - s
-
a – r, b – p, c - r
-
-
8) The freezing point of diluted milk sample is found to be – 0.2°C, while it should have been 0.5°C for pure milk. How much water been added to pure milk to make the diluted sample?
-
1 cup of water to 2 cups of pure milk
-
3 cups of water to 2 cups of pure milk
-
1 cup of water to 3 cups of pure milk
-
2 cups of water to 3 cups of pure milk
-
-
9) The complex ion that will lose its crystal field stabilization energy upon oxidation of its metal to +3 state is
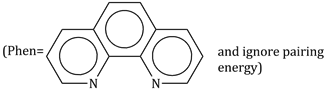
-
[Ni(phen)3]2+
-
[Zn(phen)3]2+
-
[Co(phen)3]2+
-
[Fe(phen)3]2+
-
-
10) The combination of plots which do not represent isothermal expansion of an ideal gas is:
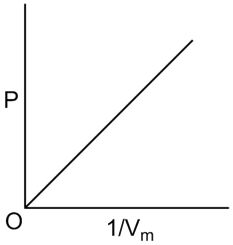
(a)
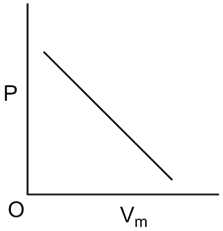
(b)
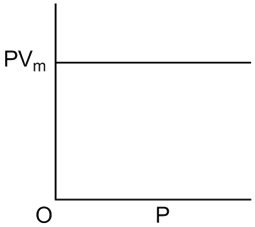
(c)
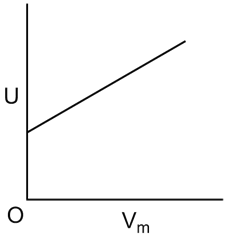
(d)
-
(b) and (d)
-
(a) and (c)
-
(b) and (c)
-
(a) and (d)
-
-
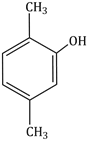
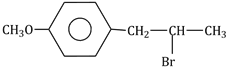
11) The major product in the following conversion is:
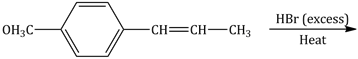
-
-
12) The idea of froth floatation method came from a person X and this method is related to the process Y of ores. X and Y, respectively, are:
-
Washer woman and concentration
-
fisher woman and concentration
-
fisher man and reduction
-
washer man and reduction
-
-
13) In the following reaction; xA? yB
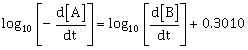
‘A’ and ‘B’ respectively can be:
-
C2H2 and C6H6
-
n-Butane and lso-butane
-
N2O4 and NO2
-
C2H4 and C4H8
-
-
14) The major product in the following reaction is:

-
-
15) The compound that inhibits the growth of tumors is:
-
trans – [Pd (Cl)2 (NH3)2]
-
trans – [Pt (Cl)2 (NH3)2]
-
cis – [Pd (Cl)2 (NH3)2]
-
cis – [Pt (Cl)2 (NH3)2]
-
-
16) For 1 molal aqueous solution of the following compounds, which one will show the highest freezing point?
-
[Co(H2O)3Cl3].3H2O
-
[Co(H2O)6Cl3
-
[Co(H2O)5Cl]Cl2.H2O
-
[Co(H2O)4Cl2]Cl.2H2O
-
-
17) The major product of the following reaction is:

-
-
18) Sodium salt of an organic acid ‘X’ produces effervescence with conc. H2SO4. ‘X’ reacts with the acidified aqueous CaCl2 solution to give a white precipitate which decolourises acidic solution of KMnO4. ‘X’ is:
-
HCOONa
-
CH3COONa
-
Na2C2O4
-
C6H5COONa
-
-
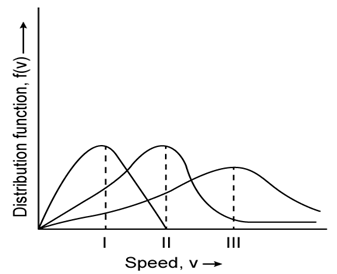
19) Points I, II and III in the following plot respectively correspond to (Vmp : most probable velocity)
-
Vmp of N2 (300K); Vmp of H2(300K); Vmp of O2(400K)
-
Vmp of H2 (300K); Vmp of N2(300K); Vmp of O2(400K)
-
Vmp of O2 (400K); Vmp of N2(300K); Vmp of H2(300K)
-
Vmp of N2 (300K); Vmp of O2(400K); Vmp of H2(300K)
-
-
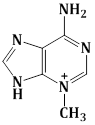
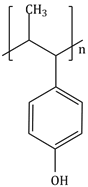
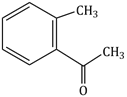
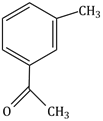
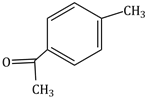
20) Compound A (C9H10O) shows positive iodoform test. Oxidation of A with KMnO4/KOH gives acid B(C8H6O4). Anhydride of B is used for the preparation of phenolphthalein. Compound A is:
-
-
21) The amount of sugar (C12H22O11) in grams required to prepare 2 L of its 0.1 M aqueous solution is:
-
22) Number of ‘Br’ will be added to product of the following reactions is/are

-
23) If Ksp of Ag2CO3 is 8 × 10-12, the molar solubility of Ag2CO3 in 0.1 M AgNO3 is 8 ×10-x M, x is
-
24) In the IUPAC name of the following compounds the position of carbon for nitro group is carbon no.____

-
25) Number of –Cl present in the product of the following reaction is:
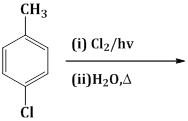
-
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
-
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25
-
1) 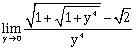
-
exists and equals
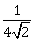
-
exists and equals
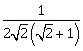
-
exists and equals

-
does not exist
-
-
2) The area (in sq. units) bounded by the parabola y = x2 – 1, the tangent at the point (2, 3) to it and the y – axis is:
-
-
3) Let a1, a2…. a30 be an A. P.,
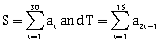 . If a5 = 27 and S – 2T = 75, then a10 is equal to:
. If a5 = 27 and S – 2T = 75, then a10 is equal to:-
52
-
57
-
47
-
42
-
-
4) The length of the chord of the parabola x2 = 4y having equation
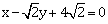 is
is -
-
5) Let
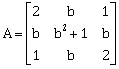 where b > 0. Then the minimum value of
where b > 0. Then the minimum value of 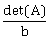 is:
is: -
-
6) A helicopter is flying along the curve given by y - x3/2 = 7, (x ? 0). A solider positioned at the point
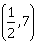 wants to shoot down the helicopter when it is nearest to him. Then this nearest distance is:
wants to shoot down the helicopter when it is nearest to him. Then this nearest distance is: -
-
7) If q is false and p ? q ? r is true, then which one of the following statement is a tautology?
-
(p ? r) ? (p ? r)
-
(p ? r) ? (p ? r)
-
p ? r
-
p ? r
-
-
8) The straight line x + 2y = 1 meets the coordinate axes at A and B. A circle is drawn through A, B and the origin. Then the sum of perpendicular distances from A and B on the tangent to the circle at the origin is:
-
-
9) In a triangle, the sum of lengths of two sides is x and the product of the lengths of the same two sides is y. If x2 – c2 = y, where c is the length of the third side of the triangle, then the circum-radius of the triangle is:
-
-
10) There are m men and two women participating in a chess tournament. Each participant plays two games with every other participant. If the number of games played by the men between themselves exceed the number of games played between the men and the women by 84, then the value of m is:
-
12
-
11
-
9
-
7
-
-
11) Let S be the set of all real values of ? such that a plane passing through the points (-? 2, 1, 1), (1, - ?2, 1) and (1, 1, - ?2) also passes through the point (-1, -1, 1). Then S is equal to:-
-
-
12) If the angle of elevation of a cloud from a point P which is 25 m above a lake be 30° and the angle of depression of reflection of the cloud in the lake from P be 60°, then the height of the cloud (in meters) from the surface of the lake is:
-
60
-
50
-
45
-
42
-
-
13) Suppose that the points (h, k), (1, 2) and (–3, 4) lie on the line L1. If a line L2 passing through the points (h, k) and (4, 3) is perpendicular to L1, then
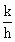 equals:
equals:-
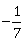
-
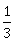
-
3
-
0
-
-
14) The tangent to the parabola y2 = 4x at the point where it intersects the circle x2 + y2 = 5 in the first quadrant, passes through the point:
-
-
15) Two vertical poles of heights, 20 m and 80m stand apart on a horizontal plane. The height (in meters) of the point of intersection of the lines joining the top of each pole to the foot of the other, from the horizontal plane is:
-
18
-
12
-
16
-
15
-
-
16) Let
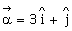 and
and 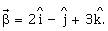 If
If 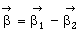 , where
, where 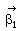 is parallel to
is parallel to 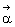 and
and 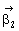 is perpendicular to
is perpendicular to 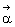 , then
, then  is equal to:
is equal to: -
-
17) If one end of a focal chord of the parabola, y2 = 16x is at (1, 4), then the length of this focal chord is:
-
25
-
24
-
22
-
20
-
-
18) Let ? and ? be the roots of the equation x2 + x + 1 = 0. Then for y ? 0 in R,
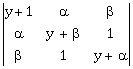 is equal to:
is equal to:-
y (y2 – 3)
-
y3 – 1
-
y3
-
y (y2 – 1)
-
-
19) Let a1, a2, a3,…. be an A. P with a6 = 2. Then the common difference of this A. P., which maximizes the product a1a4a5 is:
-
-
20) The smallest natural number n, such that the coefficient of x in the expansion of
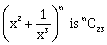 is:
is:-
38
-
58
-
23
-
35
-
-
21) Let
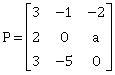 where a ∈ R. Suppose Q = [qij ] is a matrix satisfying PQ = kI3 for some non – zero k ∈ R. If q23 = –k/8 and |Q| = k2/2, then a2 + k2 is equal to __________.
where a ∈ R. Suppose Q = [qij ] is a matrix satisfying PQ = kI3 for some non – zero k ∈ R. If q23 = –k/8 and |Q| = k2/2, then a2 + k2 is equal to __________. -
22) The coefficie0nt of x18 in the product
(1+x)(1-x)10 (1+x+x2)9is:
-
23) A 2m ladder leans against a vertical wall. The top of the ladder begins to slide down the wall at the rate 25 cm/sec. If the rate (in cm/sec.) at which the bottom of the ladder slides away from the wall on the horizontal ground when the top of the ladder is 1m above the ground is
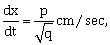 then (p – q) is equal to ___________.
then (p – q) is equal to ___________. -
24) Let
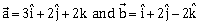 are two vectors. The magnitude of a unit vector which is perpendicular to both the vectors
are two vectors. The magnitude of a unit vector which is perpendicular to both the vectors 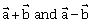 is _______.
is _______. -
25) If the curves y2 = 6x, 9x2 + by2 =16 intersect each other at right angles, then the value of 2b is:
-
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
-
- 16
- 17
- 18
- 19
- 20
- 21
- 22
- 23
- 24
- 25