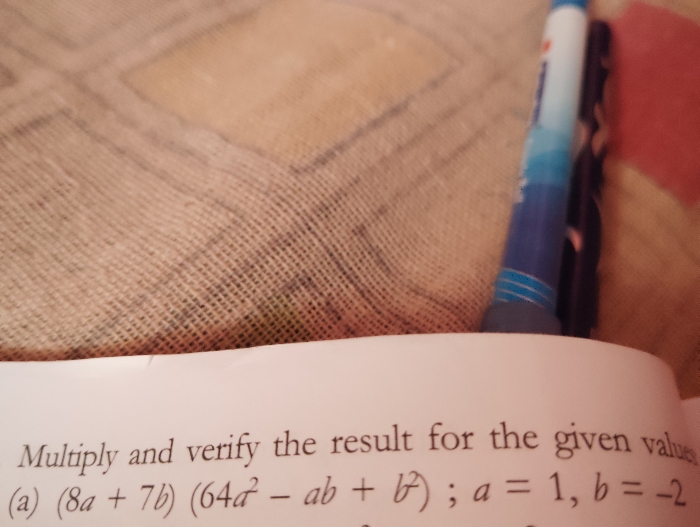Doubts and Solutions
OR
NEET NEET - Biology
Asked by anujr9937 | 04 May, 2024, 07:57: PM
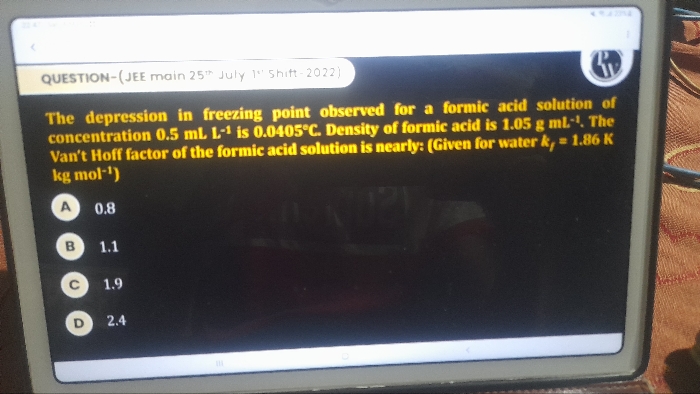
CBSE XII Science - Maths
Asked by rp978841 | 04 May, 2024, 07:37: PM
CBSE VIII - Maths
Asked by ptech3383 | 04 May, 2024, 06:10: PM